EDP Sciences logo
Subscriber Authentication Point
Search
Menu
Home All issues Volume 683 (March 2024) A&A, 683 (2024) A92 Full HTML
Open Access
Issue
A&A
Volume 683, March 2024
Article Number A92
Number of page(s) 8
Section Astrophysical processes
DOI https://doi.org/10.1051/0004-6361/202348582
Published online 11 March 2024

© The Authors 2024

Licence Creative CommonsOpen Access article, published by EDP Sciences, under the terms of the Creative Commons Attribution License (https://creativecommons.org/licenses/by/4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

This article is published in open access under the Subscribe to Open model.

Open access funding provided by Max Planck Society.

1. Introduction

The photochemical alteration of ices on silicate or carbonaceous dust grains within the dense molecular clouds of the interstellar medium offers an efficient pathway for the generation of complex organic molecules (COMs; Potapov et al. 2019; Chuang et al. 2023). A significant number of these interstellar COMs are either prebiotic themselves or are deemed potential precursors to such molecules, bearing critical relevance in the context of the origin of life (Pearce et al. 2017, 2022; Paschek et al. 2022; Oba et al. 2022). Recent laboratory experiments conducted in a space-simulated environment have substantiated this concept, demonstrating the formation of prebiotic compounds, including sugars, peptides, and nucleobases, through the processing of simple ice mixtures (Meinert et al. 2016; Oba et al. 2019; Krasnokutski et al. 2022).

Formamide (NH2CHO), among the most simple molecules containing the CHNO composition with an amide bond, is regarded as a crucial precursor in the genesis of prebiotic compounds (Saladino et al. 2012). Observations of formamide extend to diverse space environments, including galactic centres, star-forming regions, and comets (e.g. Blake et al. 1986; Turnerco 1991; Bisschop et al. 2007; Mendoza et al. 2014; López-Sepulcre et al. 2015; Bockelée-Morvan et al. 2000; Adande et al. 2013; Goesmann et al. 2015). Notably, a mass spectrometric analysis of the comet 67P/Churyumov–Gerasimenko, conducted by the Philae lander, unveiled a relative abundance of 1.8% for NH2CHO with respect to H2O (Goesmann et al. 2015). Furthermore, NH2CHO ice has been tentatively identified through the Infrared Space Observatory towards NGC 7538 IRS9 and W33A (Raunier et al. 2004; Gibb et al. 2004). The formation of NH2CHO in space is hypothesised to occur through a sequence of ion-molecule and neutral-neutral reactions in the gaseous phase, while solid NH2CHO emerges from the processing of interstellar ice mantles that coat dust grains (and references therein López-Sepulcre et al. 2019).

The decomposition of the NH2CHO molecule has been studied both theoretically and experimentally. Quantum mechanical computations have revealed that NH2CHO thermally dissociates into hydrogen cyanide (HCN), water (H2O), ammonia (NH3), carbon monoxide (CO), isocyanic acid (HNCO), and hydrogen (H2; Nguyen et al. 2011). Additionally, under the influence of a high-energy laser spark, NH2CHO gas at room temperature dissociates into nitrous oxide (N2O), hydroxylamine (NH2OH), and methanol (CH3OH), as identified through infrared (IR) spectroscopy (Ferus et al. 2011). The UV photolysis (∼5 eV) of NH2CHO on inert gas matrices at low temperatures (< 30 K) results in no dissociation but only a tautomeric conversion to formimidic acid (H(OH)C=NH; Maier & Endres 2000; Duvernay et al. 2005).

In simulated conditions designed to mimic interstellar environments, the treatment of NH2CHO ice using various processing agents, including ions, electrons, H atoms, and UV photons, has been studied. In an experiment, where NH2CHO ice was subjected to 200 keV H+ on an olivine substrate at 20 K, a range of products, including CO, CO2, N2O, HNCO, the ammonium ion (NH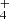 4 + $ _4^+ $), and the isocyanate ion (OCN−), were identified using IR spectroscopy (Brucato et al. 2006a). When the same experiment was replicated on an inert silicon substrate at the same temperature, NH3 and cyanide ions (CN−) were additionally generated alongside the previously mentioned products (Brucato et al. 2006b). Meanwhile, when NH2CHO ice was deposited on silica nanopowder at 70 K and exposed to 1 keV electrons, only OCN− was observed as a product (Dawley et al. 2014a). In a separate series of experiments, irradiating pure formamide at 30 K with 1 keV electrons resulted in the formation of OCN−, CO, and CO2 (Sivaraman et al. 2014). Furthermore, the reaction of H atoms with NH2CHO ice led to the production of HNCO through H-abstraction reactions (Haupa et al. 2019; Suhasaria & Mennella 2020).

The processing of NH2CHO ice using UV photons alone has undergone extensive examination under different conditions. In one instance, NH2CHO ice exposed to a xenon lamp (with energies around 4.8 eV) at 63 K showed no signs of degradation (Corazzi et al. 2020). However, the same research group observed a higher degree of formamide degradation on oxide minerals (specifically, titanium dioxide and magnesium-aluminium oxide) compared to silicates (like forsterite and antigorite). When subjected to Lyα radiation (10.2 eV), both pure NH2CHO and NH2CHO mixed with water, deposited on silica nanopowder at 70 K, resulted in the production of CO and OCN− (Dawley et al. 2014a).

In a recent study, Lyα irradiation of NH2CHO ice deposited on an inert CsI surface at 12 K led to the formation of HCN, CO, CO2, HNCO, and NH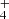 4 + $ _4^+ $ OCN− (Suhasaria & Mennella 2022). This study allowed the determination of destruction cross-sections for NH2CHO, enabling a comparison of the destructive effects of H atoms, cosmic rays, and UV photons on solid NH2CHO under simulated conditions resembling dense interstellar clouds. Our findings indicate that UV photons have an order of magnitude stronger impact on formamide degradation than cosmic rays, but their effect is three orders of magnitude weaker than that of H atoms. It is worth noting that this last experiment did not account for crucial experimental parameters such as the influence of the grain analogue surface beneath the icy mantles and the phase of the ice.

In this study we investigate the importance of a substrate at 16 K under Lyα radiation. It is noteworthy that considering the chemical properties of grains and subjecting ices to energetic processes on the surface of interstellar dust analogs has become a common practice. Pioneering experiments on this subject were conducted roughly two decades ago. In those experiments, hydrogenated carbon grains covered with a water ice cap were exposed to energetic processing to examine the efficiency of CO and CO2 formation (Mennella et al. 2004, 2006), but the role of silicate surfaces was not investigated.

Returning to our current experimental investigation, this study also employed Fourier transform infrared (FTIR) spectroscopy to evaluate the changes in NH2CHO ice after irradiation, ultimately determining destruction cross-sections. Additionally, the research explores the impact of the formamide ice phase on its response to UV irradiation. We would expect such conditions in translucent molecular clouds, protostars, and the surfaces of protoplanetary discs.

2. Experimental methods

Experiments were conducted within a high vacuum chamber, as has been extensively detailed in prior works (Suhasaria & Mennella 2020, 2022). Here, we provide a concise overview of the setup’s key components pertinent to this study. The central chamber, maintaining a base pressure lower than 10−8 mbar, is equipped with a closed-cycle helium cryostat and a dosing unit. In one series of experiments, a bare cesium iodide (CsI) window was affixed as a substrate on the cryostat’s cold finger, maintaining a temperature of 16 K. In another series, before mounting on the cold finger, CsI was coated with an amorphous silicate layer. To create this coating, we employed an unpolished natural olivine rock (dunite; Webster-Addie, North Carolina) as the target for laser ablation. We utilised a 1064 nm Nd-YAG laser (Continuum Model Surlite II) with a pulse duration of 5–7 ns, a frequency of 10 Hz, and a power density of 108 W cm−2 in Q-switch mode. Electron dispersive X-ray (EDX) analysis determined that the resulting sample possessed an Mg/Si atomic ratio of 1.7. Field emission scanning electron microscopy (FESEM) images revealed that the silicate sample exhibited a distinctive structure characterised by a fluffy structure of amorphous grain aggregates. For a comprehensive summary on the preparation of the amorphous film, we refer to Mennella et al. (2020).

NH2CHO (EMSURE grade; Merck) was purified through multiple freeze-pump-thaw cycles and was deposited onto the substrate from the dosing unit under pressures lower than 10−6 mbar. In the initial half of the experiment, we irradiated amorphous formamide, while in the latter half, we used crystalline formamide. To achieve this, we deposited formamide ice at 16 K to create amorphous ice in the first experiment. In the second experiment, we heated the amorphous formamide ice until the crystalline phase transition and then cooled it back to 16 K for further investigation.

To examine the impacts induced by UV photons, NH2CHO ice films were subjected to irradiation using a microwave-excited hydrogen flow discharge lamp, connected to the main chamber via a magnesium fluoride (MgF2) window. Lyα (10.2 eV) emission constituted 97% of the total UV emission under the chosen operational conditions (Mennella et al. 2006). The UV beam was inclined at an angle of 22.5° relative to the substrate’s normal. Throughout sample irradiation, the UV flux (fluence) was monitored by measuring the current (charge) generated via the photoelectric effect on a platinum wire positioned between the source and the substrate. Further information regarding wire sensor calibration and flux measurements at the substrate location can be found in Mennella et al. (2006). In all experiments, the NH2CHO ice sample remained optically thin at the employed UV wavelengths (Suhasaria & Mennella 2022).

The NH2CHO ice films were studied in situ both before and during irradiation in transmittance mode, within the mid-IR range, at a 2 cm−1 resolution. This analysis employed a FTIR spectrometer (Bruker Vertex 80V) fitted with a Mercury Cadmium Telluride (MCT) detector. For each individual measurement, 1024 scans were co-added. The background was acquired for every spectral measurement of NH2CHO before and after each irradiation step.

3. Results and discussion

3.1. Irradiation of amorphous formamide on amorphous silicates

Figure 1 displays the IR spectrum of amorphous NH2CHO on amorphous silicate at a temperature of 16 K prior to UV irradiation. The fundamental vibration modes associated with this spectrum are outlined in Table 1. Notably, the C–H stretch feature exhibits a blueshift, whereas the N–H stretches and the C=O stretch display redshifts in comparison to the corresponding modes observed for amorphous NH2CHO on a CsI substrate (Suhasaria & Mennella 2022). These observed spectral shifts are likely attributed to the formamide molecule interacting with distinct adsorption sites or forming different adduct structures at the surface of the amorphous silicate compared to those on the CsI surface. The thickness of the ice layer is measured at 29.5 ML, which is equivalent to 0.03 μm. The method of determining the thickness of the ice has already been described in detail in a previous work (Suhasaria & Mennella 2022).

thumbnail Fig. 1.

Mid-IR spectrum of a 29.5 monolayer (ML) NH2CHO film at 16 K on amorphous silicates, shown by black line. After irradiation with a UV fluence of 3.7 × 1018 photons cm−2 (red line), changes in the spectrum are observed, highlighted by arrows. The symbols ν indicate stretching vibrations, and the subindex a signifies an antisymmetric mode. For comparison, we also present the spectrum of a 29.5 ML thick amorphous NH2CHO film on a blank CsI substrate following the same UV fluence as above, (blue line; Suhasaria & Mennella 2022). The red and blue curves have been vertically shifted by 0.008 and −0.03, respectively, for clarity. We note that the amorphous phase of ice is denoted by “A”.

Table 1.

IR absorption band positions of (I) amorphous NH2CHO on CsI at 12 K, (II) amorphous NH2CHO on amorphous silicates at 16 K, (III) crystalline NH2CHO on CsI at 16 K, and (IV) crystalline NH2CHO on CsI at 170 K.

Figure 1 also presents the IR spectrum of NH2CHO ice on amorphous silicate after exposure to maximum UV photon fluence (3.7 × 1018 photons cm−2) considered in the experiment. As the UV irradiation increases, the IR signal associated with NH2CHO ice diminishes and at the same time gives rise to new IR peaks in the spectrum. The most prominent of these peaks is observed at 2160 cm−1, attributed to the asymmetric stretching mode of N=C=O in OCN− (Gerakines et al. 2004). Additionally, a weaker feature around 1475 cm−1 corresponds to NH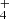 4 + $ _4^+ $ (Raunier et al. 2004). The OCN− band exhibits a slight shoulder at 2142 cm−1, attributed to the symmetric stretching mode of CO. Furthermore, the peak at 2338 cm−1 is associated with the asymmetric stretching mode of CO2. For comparison, an IR spectrum of 29.5 ML NH2CHO ice on a CsI substrate, devoid of silicates, underwent the same UV photon exposure. In this case, additional products such as NH3, HNCO, and HCN were detected alongside CO2, OCN−, and CO (Suhasaria & Mennella 2022). The difference in product formation we observed shares similarities with prior findings in a different setting, that is, when formamide ice was exposed to 200 keV protons in the presence or absence of an underlying olivine surface (Brucato et al. 2006b). In their work, they proposed that the presence of metal cations within the silicate matrix can facilitate acid-base reactions, resulting in distinct products. In our case, the natural olivine surface contains Mg2+ ions, which might account for the absence not only of NH3 (base) but also of HNCO and HCN (acids) in our experimental outcomes. This hypothesis could be further examined by performing analogous experiments on different surfaces, like carbon, which does not contain metal cations. Nevertheless, these results underscore the pivotal role played by the amorphous silicate film in modulating the photochemistry of formamide molecules under UV irradiation.

Figure 2 shows the evolution of normalised column densities, N(t) (unitless), of N–H, C–H, and C=O stretching modes of amorphous NH2CHO on amorphous silicate at 16 K as a function of the UV photon fluence, FUV. The experimental data was fitted following the first-order kinetic relation

N ( t ) = [ 1 − a 1 ( 1 − e − σ d F UV ) ] . $$ \begin{aligned} {N(t)} = [{1- a_1(1- e^{-\sigma _{d}F_{\rm UV}})]} .\end{aligned} $$(1)

thumbnail Fig. 2.

Normalised column densities of N–H (a), C–H (b), and C=O (c) are represented by filled black circles for amorphous NH2CHO on amorphous silicates at 16 K, as the UV photon fluence increases. These values are normalised using the corresponding initial column densities. The error arising due to baseline subtraction was taken into account in the error estimation. When the error bar is not visible, it indicates that the error is within the size of the filled circle. Additionally, the best fit of Eq. (1) to the data is depicted with solid red lines. The estimated destruction cross-section (σd) and the asymptotic destruction (a1) are also provided.

The equation deals with the destruction process under the boundary condition that incorporates a residual reactant at FUV = ∞. Our analysis of the experimental data in Fig. 2 allowed us to deduce the cross-sections and asymptotic values for NH2CHO destruction (σd, a1). After subjecting NH2CHO to a UV photon fluence of 3.7 × 1018 photons cm−2 for all three stretching modes, we observed that the destruction cross-section remained consistent, within the error. This suggests that the underlying silicate surface does not introduce any discernible variation in the chemical bond cleavage of the corresponding functional groups when exposed to UV photons. This outcome closely mirrors our findings during UV photon irradiation of NH2CHO ice on an uncoated CsI substrate. Furthermore, in the Lyα irradiation experiments involving NH2CHO on an uncoated CsI surface, we estimated a cross-section of σd, NH2 = 1.9 ± 0.2 × 10−18 cm2 for the -NH2 mode after a UV photon fluence of 6.5 × 1018 photons cm−2. In the current experiment, the estimated value of σd, NH2 = 1.6 ± 0.3 × 10−18 cm2 remains consistent within the range of errors. Therefore, while an amorphous silicate film may play a role in preventing the synthesis of certain molecular species, it does not exert any noticeable influence on the overall rate of destruction of the parent formamide molecule.

The average of the three estimated asymptotic fit parameters a1, N − H, a1, CH, and a1, CO after a UV photon fluence of 3.7 × 1018 photons cm−2 in formamide on amorphous silicate is 0.69 in the present experiment compared to 0.77 in the previous experiment for the same UV fluence in formamide on a blank CsI substrate (Suhasaria & Mennella 2022). This implies that formamide experiences comparatively less degradation when it is applied to the silicate substrate. This outcome contrasts with the findings of a different experiment involving the UV photolysis of pure formamide and formamide adsorbed on silicates (antigorite and forsterite), conducted using a UV-enhanced Xenon lamp (Corazzi et al. 2020). In that study, it was observed that pure formamide did not exhibit degradation, whereas the presence of silicates led to the gradual deterioration of the formamide molecule. This lack of degradation in pure formamide occurred because the energy of photons from the xenon lamp was much lower than that of Lyα.

Figure 3 depicts the variation in the column density of newly formed photo products, which has been normalised to the initial column density of NH2CHO ice film. This variation is presented as a function of UV fluence. As the CO peak appears only as a very weak shoulder to the OCN− peak, it wasn’t feasible to determine the column density of CO independently. Instead, we have simplified the analysis by deducing the column density of the OCN− peak, taking into account the OCN− band strength, which also encompasses a minor contribution from the CO peak. In Fig. 3a, we observe that the OCN− species form swiftly and prominently at the beginning of UV photolysis. However, their formation rate decelerates considerably after reaching a UV fluence of 1 × 1018 photons cm−2. In contrast, the formation of CO2, although not as swift as OCN−, exhibits a modest increase until reaching approximately 1.9 × 1018 photons cm−2 of UV fluence, after which it declines. In Fig. 3, there is also a comparison of the trends in the development of OCN− and CO2 subsequent to the irradiation of an NH2CHO ice film on an uncoated CsI substrate. It is important to note that in this particular case the column density of OCN− does not include any contribution from CO. In general, the trends in the formation of both OCN− and CO2 appear to be similar in these two scenarios, but this similarity is observed only up to a UV fluence of 1.5 × 1018 photons cm−2. Beyond this point, there is a consistent decline in the production of both these products as the UV fluence increases. In the case of UV irradiation of formamide deposited on silicates, a notable divergence in the CO2 trend at higher UV fluences is observed between the two scenarios. At a UV fluence of approximately 1.4 × 1018 photons cm−2, there is a roughly 15% increase in the formation of both OCN− and CO2 for formamide on CsI compared to formamide deposited on silicates.

thumbnail Fig. 3.

Evolution in normalised column densities of OCN− (a) and CO2 (b), represented by filled red circles, as a function of increasing UV photon fluence on amorphous NH2CHO adsorbed on amorphous silicates. The normalisation factor is the column density of NH2CHO before UV irradiation. The IR absorption areas were determined through Gaussian fitting, with error bars representing one standard deviation. In cases where the error bars are not visible, they are within the size of the data points. These results are compared with the evolution data of OCN− and CO2, indicated by filled blue triangles, following UV irradiation of amorphous formamide on a clean CsI substrate (Suhasaria & Mennella 2022).

3.2. Irradiation of crystalline formamide on CsI

Prior to delving into the irradiation of crystalline formamide ice on CsI, it is essential to address the ice preparation method and the observed alterations in the IR spectrum. The IR spectrum of 23.4 ML of amorphous NH2CHO at 16 K is displayed in Fig. 4. As the temperature incrementally exceeds 160 K, a significant change takes place in the amorphous formamide ice. By the time it reaches 170 K, the transformation becomes remarkably clear in the shape of the IR bands, displaying a sharper and more pronounced appearance, in contrast to the broad IR spectrum of the amorphous formamide. To enable a meaningful comparison, we have also incorporated the IR spectrum of crystalline formamide at 170 K, subsequent to its cooling back to 16 K. It is crucial to emphasise that the spectra of crystalline formamide ice at these two temperatures display minor differences, and the transition from amorphous to crystalline form was irreversible. Table 1 provides the vibration modes for crystalline formamide at both temperatures and shows good agreement with previous results (Sivaraman et al. 2013; Dawley et al. 2014b; Slavicinska et al. 2023). Sivaraman et al. (2013) previously observed four additional peaks in the range of 2820 to 2460 cm−1 when they conducted IR measurements following the re-cooling of their crystalline ice from 210 K to 30 K. They argued that this might be attributed to the formation of dimers or potentially polymers of formamide during the annealing process, leading to the emergence of new IR bands. In our own investigation, we have identified analogous peaks in crystalline formamide ice at 16 K, centred around 2779 cm−1, 2740 cm−1, 2707 cm−1, 2527 cm−1, and 2473 cm−1. Some of these peaks are also observable in crystalline ice at 170 K, specifically at 2764 cm−1, 2711 cm−1, 2520 cm−1, and 2472 cm−1.

thumbnail Fig. 4.

Comparison of mid-IR spectra for a 23.4 ML NH2CHO film deposited on a CsI substrate under different temperature conditions. The top curve in black represents the spectrum at 16 K immediately after deposition. The middle curve in red corresponds to the spectrum after heating the sample to 170 K, and the bottom curve in blue represents the spectrum upon cooling back to 16 K. The ice upon warming up crystallises and shows sharp features that are irreversible even after cooling back to 16 K. For clarity in visualisation, the black, red, and blue curves have been offset along the vertical axis by 0.04, 0.04, and −0.04 units, respectively. It is worth noting that the crystalline phase of ice is indicated as “C” in the spectra.

Moving to Fig. 5, we present the IR spectrum of crystalline NH2CHO ice both before and after exposure to UV photons. Notably, even following the initial fluence of 1.9 × 1017 photons cm−2, the entire crystalline ice has undergone transformation into an amorphous state, accompanied by the appearance of trace quantities of products. Initially, when comparing the evolution of stretching modes for N–H, C–H, and C=O in crystalline NH2CHO as a function of UV photon exposure with the best fit to the experimental data (represented as a solid black line) for the UV-induced degradation of amorphous NH2CHO on a CsI substrate at 12 K (Suhasaria & Mennella 2022) in Fig. 6, minor differences are apparent. However, by subtracting the initial UV exposure and designating the second irradiated point as the new starting point, then re-normalising the column densities of N–H, C–H, and C=O with respect to the column density of the new first irradiated point, it becomes evident that the newly adjusted data points for N–H and C–H closely align with the best fit. This serves as further confirmation that our irradiation is indeed directed towards the amorphous ice subsequent to the initial UV exposure on the crystalline ice.

thumbnail Fig. 5.

Mid-IR spectrum of a 23.4 ML crystalline NH2CHO film on CsI at 16 K (blue line) and after irradiation with UV fluences of 1.9 × 1017 photons cm−2 (black line) and 3.7 × 1018 photons cm−2 (red line), respectively. The crystalline ice is rapidly transformed to the amorphous form after just the first irradiation step, as is seen from the black line. The spectrum of a 29.5 ML amorphous NH2CHO film on a blank CsI substrate after a fluence of 3.7 × 1018 photons cm−2 (green line) is also shown for comparison (Suhasaria & Mennella 2022). The black curve is offset in ordinate by −0.01 for the sake of clarity.

thumbnail Fig. 6.

Normalised column densities of the N–H (a), C–H (b), and C=O (c) (filled blue circles) of crystalline NH2CHO on CsI at 16 K with increasing UV photon fluence. The normalisation factor is the corresponding initial column densities. For the sake of simplicity, the evolution is compared with the best fit to the data (solid black line) presented for amorphous NH2CHO on CsI at 12 K (Suhasaria & Mennella 2022). The two results tend to match if the first data point of the crystalline ice is subtracted and re-normalised (red triangles).

Continued irradiation of the ice results in further exposure of the amorphous formamide, leading to the formation of products identical to those observed after irradiating amorphous formamide on a blank CsI substrate, as is shown in Fig. 5. The key distinction lies in the fact that starting with an amorphous ice counterpart results in higher yields of these products. This outcome can be comprehended by considering that the molecular rearrangement of amorphous formamide ice occurs when annealed to 170 K, but maybe partially inhibited the formation of the UV products (Dawley et al. 2014a).

3.3. Irradiation of crystalline formamide on CsI at low irradiation doses

To assess the destruction cross-section or, in other terms, the amorphisation cross-section of crystalline ice, we conducted a meticulous experiment involving lower UV fluences, roughly one order of magnitude below those employed in the above experiment. With this in mind, we started irradiating 18.9 ML of crystalline NH2CHO at 16 K. Figure 7 displays the initial IR spectrum of this crystalline ice, along with corresponding spectra after multiple intervals of low-dose UV fluence. The ice retained its crystalline structure until the UV fluence reached 1.3 × 1017 photons cm−2. Beyond this threshold, the ice transformed entirely into the amorphous phase. A visual analysis of Fig. 7 reveals that, as the UV fluence increased, the distinct crystalline features gradually broadened and the peak intensity diminished. The emergence of photoproducts coincided with the loss of most of the formamide ice crystalline structure. For cross-section estimation, we relied on the initial data points when the crystalline ice fully transitioned into the amorphous phase and new products began to appear. In Fig. 8, we illustrate the trend in normalised column densities for the N–H, C–H, and C=O stretching modes of crystalline NH2CHO with increasing UV photon fluence. We fitted the experimental data with Eq. (1), yielding an estimated average cross-section of σd, UV = 1.5 × 10−17 cm2. Notably, this value was approximately an order of magnitude higher than that observed for the destruction of amorphous ice.

thumbnail Fig. 7.

Evolution of the mid-IR spectra of a 18.9 ML NH2CHO film on a blank CsI substrate at 16 K during the UV irradiation of crystalline formamide ice. The blue line indicates the fluence when the crystalline ice is completely amorphised. The red, green, blue, and pink curves are offset in ordinate by −0.001, −0.003, −0.002, and −0.008, respectively, for the sake of clarity.

thumbnail Fig. 8.

Normalised column densities of the N–H (a), C–H (b), and C=O (c) of crystalline NH2CHO on CsI at 16 K at low UV fluences (filled black squares). The normalisation factor is the corresponding initial column densities. The best fit to the data (solid red lines) is also shown. The estimated amorphisation rate (σa) and the asymptotic transformation (b) for NH2CHO are also provided.

These results diverge significantly from the findings regarding the radiation processing of amorphous and crystalline H2O, CH3OH, and N2O (Mifsud et al. 2022b,a). In their studies, they investigated the difference in the degradation between amorphous and crystalline ices due to 2 keV electrons using FTIR. Their primary discovery indicated that amorphous ice exhibited a rapid decay of their IR features compared to that in the crystalline ice. This phenomenon was more pronounced in polar ices, such as H2O (1.84 D) and CH3OH (1.69 D), than in weakly polar N2O (0.17 D) ice. The rationale behind this conclusion was that the extensive hydrogen-bonded network imparted greater stability to the crystalline structure than the amorphous ice structure, particularly in polar ices. However, in our present experiment, we observe precisely the opposite behaviour with a highly polar formamide molecule (3.71 D), characterised by an extensive hydrogen-bonded network. At this juncture, we can only speculate that the differing molecular arrangements of the formamide molecule in the two ice phases, coupled with thermal annealing to crystalline temperatures, result in dimerisation or, to some extent, polymerisation of the formamide molecule (Sivaraman et al. 2013). In summary, a comprehensive examination and comparison of radiation-induced decay patterns across different ice phases is essential for all the molecules of astrochemical interest.

4. Astrophysical implications

Up to this point, solid-state formamide has been tentatively detected exclusively in the IR spectra of massive young stellar objects (MYSOs) W33A and NGC 7538 IRS 9, as has been observed through ISO observations. In contrast, formamide has been unequivocally identified in the gas phase within numerous distinct interstellar sources, including comets and the vicinity of hot cores associated with low-mass young stellar objects (LYSOs). These observations suggest that formamide may exist within a range of thermal environments, potentially residing on the surfaces of interstellar dust grains before transitioning to the gas phase through desorption processes.

Furthermore, laboratory experiments have indicated that formamide can be formed through both atom addition (non-energetic) and energetic processes (Kaňuchová et al. 2016; Dulieu et al. 2019; Chuang et al. 2022). This implies that formamide could be present in dark interstellar clouds as part of mixed ices and potentially exist as a relatively pure molecule in the proximity of protoplanetary discs, where desorption temperatures (∼180 K; Chaabouni et al. 2018) are reached. It is worth noting that while ices in dark clouds are generally considered to be mostly amorphous, those near protoplanetary discs are more likely to exhibit crystalline characteristics.

To accurately determine the solid-state abundances of formamide in a specific region, one must consider not only the mechanisms by which formamide can form in these environments, but also how these ices are destroyed under varying conditions. Successful predictions from gas grain chemical models require the consideration of numerous parameters that impact the behaviour of these ices. Among these factors, the underlying surface and the phase of the ice, whether amorphous or crystalline, are particularly crucial and are addressed in the experimental work presented here.

Our investigation has revealed a destruction cross-section of approximately 1.9 × 10−18 cm2 for amorphous formamide ice when it is deposited on amorphous silicates or CsI. This finding is particularly intriguing in the context of ice degradation within dense cloud regions, as it appears that the presence or absence of the underlying refractory silicate grain surface does not significantly affect the kinetics. However, it is worth noting that the silicate refractory grains do play a crucial role in shaping the outcome of reactions during the UV processing of ice mantles (here formamide), by providing a unique reaction environment at the grain ice interface. This influence directly impacts the formation of products. Notably, in the presence of silicates, we observe the formation of CO, CO2, and OCN−, but there is a clear absence of NH3, HNCO, and HCN. It is also highly plausible that when ice is irradiated on carbon and silicate refractory grains, it may follow distinct chemical pathways. Therefore, it is vital to consider the significant impact of the underlying grain surface in astrochemical modelling and not overlook its role.

As was already discussed above, the presence of crystalline formamide ice in dense cloud regions is likely contingent upon the proximity of an embedded protostar. To contextualise the cross-section value determined through our current experimental efforts, we must estimate the residence time of the crystalline ice within a dense cloud environment solely exposed to internal UV radiation. It is important to note that this estimate represents an absolute upper limit, as the presence of either embedded or external stars would drastically alter the UV flux. Assuming a standard UV flux for dense regions, denoted as ϕUV = 4.8 × 103 photons cm−2 s−1 (Mennella et al. 2003), crystalline formamide ice exhibits a notably brief lifespan. A cross-section of 1.5 × 10−17 cm2 translates to a mere existence of 4 × 105 yr. This lifespan is two orders of magnitude shorter than the typical duration of dense cloud environments, which typically endure for 3 × 107 yr. Consequently, the likelihood of detecting crystalline ice within the dense interstellar clouds is significantly diminished.

5. Conclusions

In this experimental investigation, we examined the impact of Lyα irradiation on NH2CHO ice at a temperature of 16 K while considering the influence of both the underlying silicate substrate and the phase (amorphous versus crystalline) of the formamide ice on its degradation kinetics. Through the use of FTIR spectroscopy, we were able to identify the products generated during the irradiation of formamide under varying conditions.

Our findings reveal that the destruction cross-section of amorphous formamide ice remains consistent, whether or not it is in contact with an amorphous silicate substrate or not. However, the presence of amorphous silicate does inhibit the formation of NH3, HNCO, and HCN within the ice following UV processing. This effect is likely attributable to the silicate surface’s ability to facilitate acid-base reactions.

It is a widely held belief that amorphous ice degrades more rapidly than its crystalline counterpart, primarily because a significant portion of the initial energy is expended on disrupting the regular lattice structure found in crystalline ice. In the case of formamide ice subjected to UV photoprocessing, our investigation reveals that the destruction cross-section is approximately one order of magnitude greater in crystalline formamide ice compared to amorphous formamide ice.

Consequently, crystalline formamide ice is more susceptible to degradation, and therefore more challenging to detect within the environment of dark interstellar clouds. As the recently launched James Webb Space Telescope offers enhanced sensitivity and spectral resolution, it holds the potential to identify formamide within molecular ices. The insights obtained from our study could prove valuable for such detection efforts.

Acknowledgments

This work has been supported by the European Research Council under the Horizon 2020 Framework Program via the ERC Advanced Grant Origins 83 24 28, the project PRIN-INAF 2016 “The Cradle of Life- GENESIS- SKA” (General Conditions in Early Planetary Systems for the rise of life with SKA) and the Vector Stiftung (P2023-0152).

References

  1. Adande, G. R., Woolf, N. J., & Ziurys, L. M. 2013, Astrobiology, 13, 439 [NASA ADS] [CrossRef] [Google Scholar]
  2. Bisschop, S. E., Jørgensen, J. K., Van Dishoeck, E. F., & De Wachter, E. B. M. 2007, A&A, 465, 913 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  3. Blake, G. A., Sutton, E. C., Masson, C. R., & Phillips, T. G. 1986, ApJS, 60, 357 [NASA ADS] [CrossRef] [Google Scholar]
  4. Bockelée-Morvan, D., Lis, D., Wink, J. E., et al. 2000, A&A, 353, 1101 [Google Scholar]
  5. Brucato, J. R., Baratta, G. A., & Strazzulla, G. 2006a, A&A, 455, 395 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  6. Brucato, J. R., Strazzulla, G., Baratta, G. A., Rotundi, A., & Colangeli, L. 2006b, Orig. Life Evol. Biosph., 36, 451 [NASA ADS] [CrossRef] [Google Scholar]
  7. Chaabouni, H., Diana, S., Nguyen, T., & Dulieu, F. 2018, A&A, 612, A47 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  8. Chuang, K.-J., Jäger, C., Krasnokutski, S. A., Fulvio, D., & Henning, T. 2022, ApJ, 933, 107 [CrossRef] [Google Scholar]
  9. Chuang, K.-J., Jäger, C., Sie, N.-E., et al. 2023, ApJ, 956, 57 [NASA ADS] [CrossRef] [Google Scholar]
  10. Corazzi, M. A., Fedele, D., Poggiali, G., & Brucato, J. R. 2020, A&A, 636, A63 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  11. Dawley, M. M., Pirim, C., & Orlando, T. M. 2014a, J. Phys. Chem. A, 118, 1228 [NASA ADS] [CrossRef] [Google Scholar]
  12. Dawley, M. M., Pirim, C., & Orlando, T. M. 2014b, J. Phys. Chem. A, 118, 1220 [NASA ADS] [CrossRef] [Google Scholar]
  13. Dulieu, F., Nguyen, T., Congiu, E., Baouche, S., & Taquet, V. 2019, MNRAS, 484, L119 [NASA ADS] [CrossRef] [Google Scholar]
  14. Duvernay, F., Trivella, A., Borget, F., et al. 2005, J. Phys. Chem. A, 109, 11155 [NASA ADS] [CrossRef] [Google Scholar]
  15. Ferus, M., Kubelik, P., & Civis, S. 2011, J. Phys. Chem. A, 115, 12132 [NASA ADS] [CrossRef] [Google Scholar]
  16. Gerakines, P. A., Moore, M. H., & Hudson, R. L. 2004, Icarus, 170, 202 [Google Scholar]
  17. Gibb, E. L., Whittet, D. C. B., Boogert, A. C. A., & Tielens, A. G. G. M. 2004, ApJS, 151, 35 [NASA ADS] [CrossRef] [Google Scholar]
  18. Goesmann, F., Rosenbauer, H., Bredehöft, J. H., et al. 2015, Science, 349, 6247 [CrossRef] [Google Scholar]
  19. Haupa, K. A., Tarczay, G., & Lee, Y.-P. 2019, J. Am. Chem. Soc., 141, 11614 [CrossRef] [Google Scholar]
  20. Kaňuchová, Z., Urso, R. G., Baratta, G. A., et al. 2016, A&A, 585, A155 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  21. Krasnokutski, S. A., Chuang, K.-J., Jäger, C., Ueberschaar, N., & Henning, T. 2022, Nat. Astron., 6, 381 [NASA ADS] [CrossRef] [Google Scholar]
  22. López-Sepulcre, A., Jaber, A. A., Mendoza, E., et al. 2015, MNRAS, 449, 2438 [CrossRef] [Google Scholar]
  23. López-Sepulcre, A., Balucani, N., Ceccarelli, C., et al. 2019, ACS Earth Space Chem., 3, 2122 [CrossRef] [Google Scholar]
  24. Maier, G., & Endres, J. 2000, Eur. J. Org. Chem., 2000, 1061 [CrossRef] [Google Scholar]
  25. Meinert, C., Myrgorodska, I., De Marcellus, P., et al. 2016, Science, 352, 208 [NASA ADS] [CrossRef] [Google Scholar]
  26. Mendoza, E., Lefloch, B., López-Sepulcre, A., et al. 2014, MNRAS, 445, 151 [NASA ADS] [CrossRef] [Google Scholar]
  27. Mennella, V., Baratta, G. A., Esposito, A., Ferini, G., & Pendleton, Y. J. 2003, ApJ, 587, 727 [Google Scholar]
  28. Mennella, V., Palumbo, M. E., & Baratta, G. A. 2004, ApJ, 615, 1073 [NASA ADS] [CrossRef] [Google Scholar]
  29. Mennella, V., Baratta, G. A., Palumbo, M. E., & Bergin, E. A. 2006, ApJ, 643, 923 [NASA ADS] [CrossRef] [Google Scholar]
  30. Mennella, V., Ciarniello, M., Raponi, A., et al. 2020, ApJ, 897, L37 [NASA ADS] [CrossRef] [Google Scholar]
  31. Mifsud, D. V., Hailey, P. A., Herczku, P., et al. 2022a, Eur. Phys. J. D, 76, 87 [NASA ADS] [CrossRef] [Google Scholar]
  32. Mifsud, D. V., Hailey, P. A., Herczku, P., et al. 2022b, Phys. Chem. Chem. Phys., 24, 10974 [NASA ADS] [CrossRef] [Google Scholar]
  33. Nguyen, V. S., Abbott, H. L., Dawley, M. M., et al. 2011, J. Phys. Chem. A, 115, 841 [NASA ADS] [CrossRef] [Google Scholar]
  34. Oba, Y., Takano, Y., Naraoka, H., Watanabe, N., & Kouchi, A. 2019, Nat. Commun., 10, 4413 [NASA ADS] [CrossRef] [Google Scholar]
  35. Oba, Y., Takano, Y., Furukawa, Y., et al. 2022, Nat. Commun., 13, 2008 [NASA ADS] [CrossRef] [Google Scholar]
  36. Paschek, K., Kohler, K., Pearce, B. K., et al. 2022, Life, 12, 404 [NASA ADS] [CrossRef] [Google Scholar]
  37. Pearce, B. K., Pudritz, R. E., Semenov, D. A., & Henning, T. K. 2017, Proc. Nat. Academy Sci., 114, 11327 [NASA ADS] [CrossRef] [Google Scholar]
  38. Pearce, B. K. D., Molaverdikhani, K., Pudritz, R. E., Henning, T., & Cerrillo, K. E. 2022, ApJ, 932, 9 [NASA ADS] [CrossRef] [Google Scholar]
  39. Potapov, A., Theulé, P., Jäger, C., & Henning, T. 2019, ApJ, 878, L20 [NASA ADS] [CrossRef] [Google Scholar]
  40. Raunier, S., Chiavassa, T., Duvernay, F., et al. 2004, A&A, 416, 165 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  41. Saladino, R., Crestini, C., Pino, S., Costanzo, G., & Mauro, E. D. 2012, Phys. Life Rev., 9, 84 [NASA ADS] [CrossRef] [Google Scholar]
  42. Sivaraman, B., Sekhar, B. N. R., Nair, B. G., Hatode, V., & Mason, N. J. 2013, Spectrochim. Acta A, 105, 238 [NASA ADS] [CrossRef] [Google Scholar]
  43. Sivaraman, B., Nair, B. G., Sekhar, B. N. R., et al. 2014, Chem. Phys. Lett., 608, 404 [NASA ADS] [CrossRef] [Google Scholar]
  44. Slavicinska, K., Rachid, M. G., Rocha, W. R. M., et al. 2023, A&A, 677, A13 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  45. Suhasaria, T., & Mennella, V. 2020, A&A, 641, A88 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  46. Suhasaria, T., & Mennella, V. 2022, A&A, 662, A73 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  47. Turnerco, B. E. 1991, ApJS, 76, 617 [NASA ADS] [CrossRef] [Google Scholar]

All Tables

Table 1.

IR absorption band positions of (I) amorphous NH2CHO on CsI at 12 K, (II) amorphous NH2CHO on amorphous silicates at 16 K, (III) crystalline NH2CHO on CsI at 16 K, and (IV) crystalline NH2CHO on CsI at 170 K.

In the text

All Figures

thumbnail Fig. 1.

Mid-IR spectrum of a 29.5 monolayer (ML) NH2CHO film at 16 K on amorphous silicates, shown by black line. After irradiation with a UV fluence of 3.7 × 1018 photons cm−2 (red line), changes in the spectrum are observed, highlighted by arrows. The symbols ν indicate stretching vibrations, and the subindex a signifies an antisymmetric mode. For comparison, we also present the spectrum of a 29.5 ML thick amorphous NH2CHO film on a blank CsI substrate following the same UV fluence as above, (blue line; Suhasaria & Mennella 2022). The red and blue curves have been vertically shifted by 0.008 and −0.03, respectively, for clarity. We note that the amorphous phase of ice is denoted by “A”.
In the text
thumbnail Fig. 2.

Normalised column densities of N–H (a), C–H (b), and C=O (c) are represented by filled black circles for amorphous NH2CHO on amorphous silicates at 16 K, as the UV photon fluence increases. These values are normalised using the corresponding initial column densities. The error arising due to baseline subtraction was taken into account in the error estimation. When the error bar is not visible, it indicates that the error is within the size of the filled circle. Additionally, the best fit of Eq. (1) to the data is depicted with solid red lines. The estimated destruction cross-section (σd) and the asymptotic destruction (a1) are also provided.
In the text
thumbnail Fig. 3.

Evolution in normalised column densities of OCN− (a) and CO2 (b), represented by filled red circles, as a function of increasing UV photon fluence on amorphous NH2CHO adsorbed on amorphous silicates. The normalisation factor is the column density of NH2CHO before UV irradiation. The IR absorption areas were determined through Gaussian fitting, with error bars representing one standard deviation. In cases where the error bars are not visible, they are within the size of the data points. These results are compared with the evolution data of OCN− and CO2, indicated by filled blue triangles, following UV irradiation of amorphous formamide on a clean CsI substrate (Suhasaria & Mennella 2022).
In the text
thumbnail Fig. 4.

Comparison of mid-IR spectra for a 23.4 ML NH2CHO film deposited on a CsI substrate under different temperature conditions. The top curve in black represents the spectrum at 16 K immediately after deposition. The middle curve in red corresponds to the spectrum after heating the sample to 170 K, and the bottom curve in blue represents the spectrum upon cooling back to 16 K. The ice upon warming up crystallises and shows sharp features that are irreversible even after cooling back to 16 K. For clarity in visualisation, the black, red, and blue curves have been offset along the vertical axis by 0.04, 0.04, and −0.04 units, respectively. It is worth noting that the crystalline phase of ice is indicated as “C” in the spectra.
In the text
thumbnail Fig. 5.

Mid-IR spectrum of a 23.4 ML crystalline NH2CHO film on CsI at 16 K (blue line) and after irradiation with UV fluences of 1.9 × 1017 photons cm−2 (black line) and 3.7 × 1018 photons cm−2 (red line), respectively. The crystalline ice is rapidly transformed to the amorphous form after just the first irradiation step, as is seen from the black line. The spectrum of a 29.5 ML amorphous NH2CHO film on a blank CsI substrate after a fluence of 3.7 × 1018 photons cm−2 (green line) is also shown for comparison (Suhasaria & Mennella 2022). The black curve is offset in ordinate by −0.01 for the sake of clarity.
In the text
thumbnail Fig. 6.

Normalised column densities of the N–H (a), C–H (b), and C=O (c) (filled blue circles) of crystalline NH2CHO on CsI at 16 K with increasing UV photon fluence. The normalisation factor is the corresponding initial column densities. For the sake of simplicity, the evolution is compared with the best fit to the data (solid black line) presented for amorphous NH2CHO on CsI at 12 K (Suhasaria & Mennella 2022). The two results tend to match if the first data point of the crystalline ice is subtracted and re-normalised (red triangles).
In the text
thumbnail Fig. 7.

Evolution of the mid-IR spectra of a 18.9 ML NH2CHO film on a blank CsI substrate at 16 K during the UV irradiation of crystalline formamide ice. The blue line indicates the fluence when the crystalline ice is completely amorphised. The red, green, blue, and pink curves are offset in ordinate by −0.001, −0.003, −0.002, and −0.008, respectively, for the sake of clarity.
In the text
thumbnail Fig. 8.

Normalised column densities of the N–H (a), C–H (b), and C=O (c) of crystalline NH2CHO on CsI at 16 K at low UV fluences (filled black squares). The normalisation factor is the corresponding initial column densities. The best fit to the data (solid red lines) is also shown. The estimated amorphisation rate (σa) and the asymptotic transformation (b) for NH2CHO are also provided.
In the text

Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.

Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.

Initial download of the metrics may take a while.