EDP Sciences logo
Subscriber Authentication Point
Search
Menu
Home All issues Volume 666 (October 2022) A&A, 666 (2022) A134 Full HTML
Open Access
Issue
A&A
Volume 666, October 2022
Article Number A134
Number of page(s) 11
Section Atomic, molecular, and nuclear data
DOI https://doi.org/10.1051/0004-6361/202244330
Published online 19 October 2022

© M. Sanz-Novo et al. 2022

Licence Creative CommonsOpen Access article, published by EDP Sciences, under the terms of the Creative Commons Attribution License (https://creativecommons.org/licenses/by/4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

This article is published in open access under the Subscribe-to-Open model. Subscribe to A&A to support open access publication.

1 Introduction

Observation of amino acids and their most essential isomers and potential precursors in the interstellar medium (ISM) should be crucial for revealing the chemistry that may have led to life's origin (Ehrenfreund et al. 2001). In particular, the central question of whether glycine (CH2(NH2)C(O)OH) exists or not in the ISM is one of the most pursued targets in astrochemistry. Although several attempts to observe glycine have been reported (Hollis et al. 2003; Cunningham et al. 2007; Jones et al. 2007; Jiménez-Serra et al. 2016, 2020), its detection has never been confirmed (Snyder et al. 2005). Nevertheless, it has been found in the coma of comets 67P/Churyumov–Gerasimenko through in situ mass spectrometry (Altwegg et al. 2016). The presence of glycine in the volatile cometary material thus strongly suggests the existence of a process capable of generating amino acids in cold environments (Bizzocchi et al. 2020). Hence, as a prerequisite step for its astronomical identification, the rotational spectra of glycinamide (CH2 (NH2)C(O)NH2; Alonso et al. 2018; Kisiel et al. 2022) and aminoacetonitrile (CH2(NH2)CN; Kolesniková et al. 2017), which are relevant intermediates in the Strecker synthesis of glycine; hydantoin (CH2C(O)NHC(O)NH; Alonso et al. 2017) and hydantoic acid (C(O)OHCH2NHC(O)NH2; Kolesniková et al. 2019), potential glycine precursors through a different hydrolytic pathway (Ozeki et al. 2017), and the glycine isomer glycolamide (CH2(OH)C(O)NH2); Sanz-Novo et al. 2020) have been recently reported. In addition, Sanz-Novo et al. (2019) very recently carried out a computational study of the potential energy surfaces (PES) corresponding to the formation reactions of several protonated glycine isomers. Surprisingly, the only exothermic process with no net activation barrier led to protonated acetohydroxamic acid [CH3C(O)NH2OH]+. Its formation could therefore be feasible under interstellar conditions. Consequently, the corresponding neutral counterpart, CH3C(O)NHOH, might be a candidate molecule to be searched for in the ISM. Our previous simulations (Sanz-Novo et al. 2019) suggest that this glycine isomer should be searched for in the ISM, eventually, but there is no experimental rotational data available for this molecular system.

Hydroxamic acids (HA) constitute a family of natural compounds with the formula (R1CONR2OH; R1 and R2 as organic residues), and usually exhibit biological activity (Miller 1989; Bugg 2014; Muri et al. 2002; Marmion et al. 2004; Pal & Saha 2012; Das et al. 2017). HA are N-hydroxy substituted derivatives of amines and involve, like amides, the fragment of the simplest protein structure HNC = O. Moreover, it is of great astrochemical interest to study peptide-like bond molecules, given that this type of bond can play an essential role in the generation of proteins in the early stages of Earth (Belloche et al. 2017; Colzi et al. 2021). The simplest molecule containing a peptide bond, formamide (NH2CHO), was detected in the interstellar medium (ISM) in the 1970s in Sagittarius B2 (Rubin et al. 1971) and later in Orion KL (Turner 1991). Since then, several amides have been identified in the ISM, such as cyanamide (NH2CN, Ligterink et al. 2020), acetamide (CH3C(O)NH2, Hollis et al. 2006; Halfen & Ziurys 2011; Belloche et al. 2019; Ligterink et al. 2020, 2022) and more recently, N-methylformamide (CH3NHCHO, Ligterink et al. 2020; Colzi et al. 2021), whose interstellar detection was finally confirmed after the previous tentative detection reported in Belloche et al. (2017). Following this, we further propose the study of acetohydroxamic acid (CH3C(O)NHOH, hereafter AHA) as an appealing target for interstellar detection.

This compound is a highly hygroscopic solid with a melting point of 88–90 °C and very low vapor pressure, which represents a challenge for high-resolution rotational spectroscopy due to its intricate vaporization. Except for the Fourier transform infrared (FTIR) studies by matrix isolation techniques (Saldyka & Mielke 2004, 2018), it has only been investigated in the condensed phases by X-ray spectroscopy (Bracher & Small 1970), remaining unexplored in the gas phase. Lately, efficient procedures have been devoted to generating the neutral forms of proteinogenic amino acids by laser ablation, allowing their conformational investigation using Fourier transform microwave (FTMW) techniques (Alonso & López 2015). The laser ablation technique enables an ultra-fast vaporization process of organic and biological molecules from the solid-state to the gas phase and further probes them in the isolated conditions of a supersonic jet (Alonso et al. 2021). Following this approach, we present the first high-resolution rotational study of AHA. In this work, we aim to provide a precise set of spectroscopic constants of this glycine isomer including the values of the 14N nuclear quadrupole coupling constants to permit its distinct possible identification in low-frequency regions using, for instance, the Green Bank Telescope (GBT) and the Yebes 40 m telescope. For low-frequency observations (i.e., those conducted in the Q band), accurate experimental data of the hyperfine structure can help the robust identification of molecules in the ISM. Furthermore, new gas-phase theoretical and experimental studies exploring related species should represent a benchmark investigation in constraining models of the formation of amino acids in space, and in predicting what other complex molecules might be awaiting detection in the ISM.

Here, we describe in Sect. 2 the experimental setup that was used to measure the spectra of AHA. In Sects. 2.2 and 2.3, we present the results and discussion of the analysis of the rotational spectra. The astronomical results of a search for AHA toward the molecular clouds TMC-1 and G+0.693-0.027 are reported in Sect. 3 (see Sects. 3.1 and 3.2, respectively). Finally, we present in Sect. 4 the conclusions of this work.

2 Laboratory spectroscopy

2.1 Rotational spectra measurements

With the aim of providing rotational signatures of the isolated AHA free from environmental disturbances, we used broadband chirped pulse Fourier transform microwave spectroscopy equipped with a laser ablation vaporization system (LA-CP-FTMW, Peña et al. 2013). In the experimental procedure, finely powdered AHA was mixed with a small amount of a commercial copolymeric binder as well as a small quantity (~5%) of starch (wheat amylum), which was used as a thickening and gluing agent; these ingredients were pressed into cylindrical rods, which were ablated by using the third harmonic (355 nm, 20 mJ per pulse) of an Nd-YAG picosecond laser. The vaporized products were seeded in neon at a backing pressure of 10 bar and adiabatically expanded into the vacuum chamber of the spectrometer, and then probed by laser ablation broadband chirped pulse Fourier transform microwave spectroscopy. A high-power excitation pulse of 300 W was used to polarize the molecules at frequencies from 6.8 to 18 GHz (in three different intervals: 6.8–14.2, 14–16, and 16–18GHz). Up to 60000 individual free induction decays (FIDs), with four FIDs on each valve cycle at a 2 Hz repetition rate, were averaged in the time domain, and then a Fourier transform was performed to obtain the broadband frequency domain spectrum.

Afterward, we employed our double-resonance (DR-LA-MB-FTMW) configuration (Sanz-Novo et al. 2020; Mori et al. 2009) to extend the frequency coverage of our microwave data up to 40 GHz. In this technique, we employ an additional synthesizer and send higher-in-frequency radiation into the Fabry– Pérot cavity of the FTMW spectrometer. Meanwhile, we monitor a microwave transition by the FTMW system and indirectly detect transitions induced by a population change and/or a loss of coherence of the monitored transition. Hence, each line was observed as a change in the intensity of the monitored transition and measured using an active background subtraction method. We note that the hyperfine structure can also be resolved using this technique. Consequently, this configuration is of great importance because it enables taking measurements in the same frequency region covered by astronomical surveys conducted at centimeter wavelengths, for instance with the GBT, and also serves as a straightforward connection between the microwave and possible millimeter-wave data.

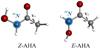thumbnail Fig. 1

Structures of the AHA conformers, showing the presence of a 14N nucleus. V3 is the barrier height associated to the methyl internal rotation motion. Color-coding: carbon atoms are in grey; nitrogen atoms are in blue, oxygen atoms are in red, and hydrogen atoms are in white.

2.2 Jet-cooled broadband spectrum

The AHA molecule is rather flexible an exhibits Z(zusammen, cis)-E(entgegen, trans) isomerization due to internal rotation around the C–N bond (see Fig. 1). In the gas phase the Z- and E- conformers are of similar stability, but their interconversion is hindered by barriers greater than 70.3 kJ mol−1 (8455 K) (Saldyka & Mielke 2018). Before starting the experimental study and to guide the spectral search, we extended the previous theoretical calculations (Sanz-Novo et al. 2019) to all the low-energy conformers of AHA. The presence of the 14N nucleus in our molecule is expected to be a helpful tool for identifying structures, since it introduces hyperfine rotational probes at defined sites of AHA, depending on the chemical environment of the 14N nucleus. Thus, the treatment and interpretation of the quadrupole hyperfine structure of AHA is needed to conclusively unveil the observed species. Two individual relaxed potential-energy scans were computed, choosing the HCCO torsional angle as the driving coordinate to study the methyl internal rotation. Thus, intermediate barrier height values were predicted (see Table 1), pointing to a splitting of the same order of magnitude as that expected in the 14N quadrupole hyperfine structure.

The broadband spectrum of AHA from 6.8 to 14.2 GHz is shown in Fig. 2a. According to the predictions of Table 1, the two plausible conformers are expected to be near-prolate asymmetric tops with a nonzero μa and μb component of the electric dipole moment. In this context, their R-branch μa-type spectra are expected to show distinguishing arrangements of individual Ka transitions spaced approximately B + C. Furthermore, all the observed transitions were split into several close hyperfine components showing the characteristic pattern due to a 14N nucleus, which confirms the presence of a single nitrogen atom in the observed species. Moreover, additional splittings of several MHz were observed in most of the transitions, which significantly complicated the data analysis (see illustration in Fig. 2b). Given that AHA is a closed-shell molecule, no other fine or hyperfine structure in the rotational spectra except that arising from the coupling between the internal and overall rotation is expected to occur. Thus, we attributed the splitting to the internal rotation of the methyl group causing the occurrence of the A–E doublets (Gordy & Cook 1984). Taking this into account, we were able to detect two sets of strong μa-type R-branch progressions (J+1)0,J+1 ← J0,J and (J + 1)1,J+1 ← J1,J, corresponding to the A-symmetry state of rotamer I (see illustration in Fig. 2b).

Afterward, we employed the XIAM program (Hartwig & Dreizler 1996) to predict the E transitions using the theory-calculated barrier to the internal rotation V3. The subsequent iterative fitting and prediction procedure allowed us to observe new a-type transitions and to extend our measurements to the R-branch b-type spectrum. Although the resolution of our broadband CP-FTMW technique was sufficient to partly resolve the 14N nuclear quadrupole hyperfine patterns for some transitions, in the first step we did not attempt to analyze the hyperfine structure. Instead, we measured and fitted (Pickett 1991) the intensity-weighted mean of the hyperfine line cluster to a rigid rotor Hamiltonian to generate a preliminary set of rotational constants (A = 10642.7, B = 4106.3 and C = 3024.2 in MHz) for rotamer I.

After excluding the lines belonging to rotamer I, another weaker progression of a-type R-branch transitions was discovered and assigned to another rotamer (labeled II). The assignments were confirmed by predicting and measuring new a- and b-type lines (additional rotational lines), which are also subjected in some cases to a different A–E splitting. After the corresponding fitting, a first set of constants (A = 8951.5, B = 4320.7 and C = 3022.8 in MHz) were derived from a rigid rotor analysis.

The fundamental basis for the identification of the observed rotamers is derived from the values of the rotational constants. The comparison of the experimental and the predicted rotational parameters in Table 1 shows that the observed rotational constants are in good agreement with those B2PLYPD3 calculated for the Z- and E-conformers. Therefore, this allows the unambiguous conformational identification of rotamers I and II as the lowest-lying energy Z- and E-AHA conformers, respectively.

Table 1

Theoretical and experimental spectroscopic parameters for the A-symmetry state of the observed conformers of AHA (A-Reduction, Ir-representation).

2.3 Fine and hyperfine structure: narrowband spectrum

A more puzzling task was the resolution of the fine and hyperfine structure. First, the A–E split structure attributed to the methyl internal rotation motion can be generally used as a molecular “fingerprint” to search for a molecule in an astronomical line survey (Cernicharo et al. 2016; Belloche et al. 2017). Moreover, it is known that for interstellar searches using surveys that done at centimeter wavelengths (i.e., those conducted with telescopes such as GBT and Yebes 40m), the recognition of hyperfine patterns in the observed spectra significantly help in the proper identification of interstellar molecules (McCarthy & McGuire 2021). As an example, the analysis of the hyperfine structure of several N-bearing species, such as N-protonated isocyanic acid (H2NCO+, Rodríguez-Almeida et al. 2021b) which has been detected toward G+0693-0.027 along with ethyl isocyante (C2H5NCO, Kolesniková et al. 2018), was required for its conclusive line-by-line identification. In this context, sensitive data at centimeter wavelengths are crucial because in TMC-1 and in G+0.693-0.027 the excitation temperatures of the molecules are very low, and hence the brightest lines of this relatively complex species fall at those frequencies. These interstellar sources have recently been suggested to be among the best targets to search for large molecular systems (McGuire et al. 2021; Rivilla et al. 2021b), further supporting centimeter-wave studies of as-yet-undiscovered interstellar candidates. Hence, we employed our narrowband LA-MB-FTMW spectrometer (León et al. 2021) to completely resolve both fine and hyperfine structures. We initially used the SPFIT/SPCAT program package (Pickett 1991) to analyze hyperfine patterns for the previously measured A and E lines of Z-AHA (see Fig. 3). This scrutiny was followed by measuring additional hyperfine components of several a- and b-type R-branch lines. Once we completed the analysis, new R-branch transitions belonging to the E-conformer were identified.

In a quest to extend the measurements of the Z-conformer (global minimum and also most reasonable interstellar candidate) to higher frequencies and to allow for a direct comparison with spectral line surveys carried out with Yebes 40 m and GBT telescopes, we used our double-resonance (DR) configuration, which also enables the resolution of the hyperfine components (see additional details in Sect. 2). Subsequently, we performed a fit (Pickett 1991) including our broadband and narrowband microwave data as well as the double-resonance lines of the A-symmetry state. We used the A-reduction Hamiltonian in the Ir representation (Watson 1977), supplemented with an additional term that accounts for the nuclear quadrupole coupling interactions (Foley 1947; Robinson & Cornwell 1953). The Hamiltonian was set up in the coupled basis set (F = J + I) to label the energy levels involved in each rotational transition in terms of the quantum numbers J, Ka, Kc, and F. The effective derived spectroscopic parameters for Z-AHA, including the 14N quadrupole coupling constants (χaa, χbb,;χcc), are listed in the first column of Table 1. We also provide a sample list of the measured transitions of Z-AHA in Tables 2 and A.3, while a sample list of E-AHA is presented in Table A.4.

Furthermore, the same sets of A and E lines were used to experimentally determine the internal rotation barrier V3 for both conformers using the inertial axis method, which is already implemented in the XIAM program (Hartwig & Dreizler 1996). Z-AHA presents a barrier height of 255.379 (62) cm−1, which is in close agreement with the theoretically predicted data (276.2 cm−1). Like the Z-form, E-AHA also exhibits a splitting due to the methyl internal rotation, which in this case is slightly more hindered due to the crowded environment of the methyl group (464.19 (69) cm−1). The results derived from the A/E XIAM fit, including the 14N nuclear quadrupole hyperfine structure, are presented in Table A.1. Nevertheless, it should be noted that in the XIAM fit we were not able to include all the measured lines since some of them showed an unresolved A–E splitting. Additionally, in cases where the A-E splitting is resolvable in the astronomical spectra, a fit that reproduces both A- and E-symmetry states is convenient to search for the molecule in the ISM. Therefore, for astronomical purposes, we performed an effective single-state fit based on perturbation approximations as implemented in the SPFIT program (Pickett 1991; see Table A.2). This approach works reasonably well in certain cases including relatively small data sets of low-J values obtained in supersonic expansion measurements, for example the present investigation, and allows us to reproduce our complete experimental data set. It has been described in detail and employed to analyze molecules such as o-chlorotoluene (Gerhard et al. 2003), pyruvic acid (Kisiel et al. 2007) and pyruvonitrile (Kraśnicki et al. 2010).

Finally, in Table 3 we provide the rotational (Qr) and vibrational (Qv) partition functions of the Z-AHA. We used SPCAT (Pickett 1991) to compute the values of Qr from first principles at typical temperatures, as applied in the JPL database (Pickett et al. 1998) using the spectroscopic constants derived from the single-state effective fit and the following expression (Gordy & Cook 1984): 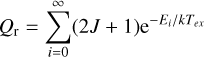Qr=∑i=0∞(2J+1)e−Ei/kTex${Q_{\rm{r}}} = \sum\limits_{i = 0}^\infty {(2J + 1){{\rm{e}}^{ - {E_i}/k{T_{ex}}}}}$(1)

Additionally, the values of (Qr) were multiplied by the nuclear spin statistics contribution (Qn = 3 due to the presence of a 14N nucleus) and the total spin weight [Qs = (2I + 1)3 = 8, where I = 1/2]. We note that the last correction factor is needed to account for the spin weight of the CH3. A similar approach was followed by Groner et al. (2007). On the other hand, the vibrational part, Qv, was estimated employing an anharmonic approximation and Eq. (3.60) of Gordy & Cook (1984) as 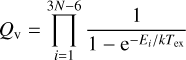Qv=∏i=13N−611−e−Ei/kTex${Q_{\rm{v}}} = \prod\limits_{i = 1}^{3N - 6} {{1 \over {1 - {{\rm{e}}^{ - {E_i}/k{T_{{\rm{ex}}}}}}}}}$(2)

where all the vibrational modes were taken into account. The frequencies of the normal modes were computed at the MP2/aug-cc-pVTZ level of theory using a second-order perturbation treatment (VPT2, Hoy et al. 1972), which includes a full cubic force field (CFF) and semi-diagonal quartic force constants. Thus, the total partition function, Qtot can be calculated as the product of Qr and Qv.

In summary, we explored the rotational spectrum of acetohydroxamic acid in the microwave region (up to 40 GHz). We provided very accurate laboratory data as a preliminary step to search for the molecule toward different regions of the ISM. Finally, a line catalogue including both A- and E-symmetry states and also the 14N hyperfine structure was generated to be eventually compared with diverse observational data sets, such as the GBT GOTHAM survey (McGuire et al. 2020), the Yebes 40 m QUIJOTE line survey (Cernicharo et al. 2021), and the Yebes 40 m survey toward G+0.693-0.027 (Rodríguez-Almeida et al. 2021a), as well as Effelsberg 100 m observations (Sanz-Novo et al. 2020). Of the two experimentally detected conformers, Z-AHA is the global minimum, which further motivates its interstellar search; the highest-energy conformer, E-AHA, is located at 772.8 cm−1 (1112 K). This experimental microwave data enable a confident search of acetohydroxamic acid toward the above-mentioned low-frequency surveys, which have been proven to be the best data sets to look for large molecules based on multiple first detections performed over the last few years (see e.g. McGuire et al. 2021; Cernicharo et al. 2021; Rivilla et al. 2022a; Jimenez-Serra et al. 2022).

thumbnail Fig. 2

Rotational spectrum of AHA. (a) Broadband LA-CP-FTMW spectrum of AHA from 6.8 to 14.2 GHz. Strong a-type R-branch (J + 1)0,J+1 ← J1,j and (J + 1)1,J+1 ← J0,J transitions of rotamer I (Z-AHA, in blue) are shown, as well as some transitions of rotamer II (E-AHA, in red). (b) Zoom-in on the broadband spectrum showing the 10,1 ← 00,0 rotational transition for both acetohydroxamic acid species highlighting the A–E doublets due to the internal rotation of the methyl group. The intensity is given in arbitrary units.
thumbnail Fig. 3

Partially resolved hyperfine pattern for the 30,3 ← 21,2 transition of the A-symmetry ground state of Z-AHA (LA-CP-FTMW experiment) and completely resolved hyperfine structure using the cavity-based (LA-MB-FTMW) experiment, where each transition appears as Doppler doublets due to the sub-Doppler resolution of the spectrometer. The simulated spectrum is also depicted for comparison (in blue). The intensity is given in arbitrary units.

3 Interstellar search for Z-AHA

3.1 TMC-1 dark starless core

Using the line catalogs generated from this work, we searched for acetohydroxamic acid toward the cold, dark starless core TMC-1 using datafromthe100m Robert C. Byrd Green Bank Telescope (GBT) obtained as part of the GOTHAM (GBT Observations of TMC-1: Hunting Aromatic Molecules) project. A detailed description of the project is provided in McGuire et al. (2020), and the data used are from the third data reduction (“DR3”) described in Barnum et al. (2022).

The observations target the “cyanopolyyne peak” of the region located at (J2000) α = 04h41m42s.50 δ = +25°41′26″.8. The frequency coverage spans roughly 8–11.6 GHz and 18–29.5 GHz, with a few small gaps and with a frequency resolution of 1.4 kHz corresponding to a velocity resolution of 0.01–0.05 km s−1 across the full range. The noise level of the data vary over the full range; ultimately the survey is intended to reach ~2–3 mK RMS noise in 0.1 km s−1 channels. Much of the DR3 data nears this already, but some portions have higher levels (~10 mK).

No signal is seen from acetohydroxamic acid in these observations. To derive an upper limit, we assume that the transitions within range would be well described by a single excitation temperature (Tex) and use the MOLSIM Python module (Lee et al. 2021) to simulate the spectrum using the formalisms of Turner (1991). Although most molecules in TMC-1 are known to show signal in 3–4 closely spaced velocity components centered around υlsr = 5.8 km s−1 (Xue et al. 2020; Dobashi et al. 2018, 2019), the relative intensities of these components are not consistent between species. We therefore elected to derive the limit assuming a single lineshape with a FWHM of ∆V = 0.3 km s−1 centered at a υlsr = 5.8 km s−1. The source was assumed to fill the beam, and the excitation temperature was taken to be Tex = 7 K.

Using these parameters, we derived a 3σ upper limit to the column density of acetohydroxamic acid of NT ≤ 1 × 1013 cm−2 which translates to a molecular abundance with respect to molecular hydrogen of 1 × 10−9, assuming a value of N(H2) = 1022 cm−2 (Gratier et al. 2016). Figure 4 shows a simulation of the acetohydroxamic acid lines used to constrain this limit near 25 GHz overlaid on the GOTHAM observations. For comparison, a 3σ upper limit derived using the same procedure for its isomer glycine (conformer I) is also ≤l×1013 cm−2, assuming the same physical parameters.

Table 2

Sample list of the measured transition frequencies for the A-symmetry state of AHA.

Table 3

Rotational and vibrational partition functions of Z-AHA.

thumbnail Fig. 4

Simulation of acetohydroxamic acid emission at the 3σ upper limit column density derived toward TMC-1 using the parameters outlined in the text (in red) overlaid on the GOTHAM observations of TMC-1 (in black). The features shown arise from the 31,з–20,2 set of transitions. The spectra have been adjusted to the nominal source υlsr = 5.8 km s−1.

3.2 G+0.693-0.027 molecular cloud

We searched for AHA conformers toward the G+0.693-0.027 molecular cloud, located in the Sgr B2 region of the Galactic Center. This source exhibits a rich molecular complexity, and numerous molecular species have been detected for the first time toward it (see, e.g., Rivilla et al. 2019, 2020, 2021b,a, 2022a,b; Rodríguez-Almeida et al. 2021a,b; Jimenez-Serra et al. 2022; Zeng et al. 2021). We used a sensitive unbiased spectral survey performed with the Yebes 40m (Guadalajara, Spain) and the IRAM 30 m (Granada, Spain) telescopes. The position switching observations were centered at α(J2000.0)= 17h47m22s, δ(J2000.0) = −28°21′27″. The Yebes 40 m observations covered a spectral range from 31.075 GHz to 50.424 GHz, while the IRAM 30 m observations cover the spectral range 71.770 to 116.720 GHz. More detailed information of the observational survey is presented in Rivilla et al. (2021b); Rodríguez-Almeida et al. (2021a).

We implemented the spectroscopy presented in this work into the MADCUBA package1; version 11 March 2022 (Martin et al. 2019). Using the Spectral Line Identification and Modeling (SLIM) tool of MADCUBA, we generated a synthetic spectra of AHA under the assumption of local thermodynamic equilibrium (LTE), and compared them with the observed spectra. As occurs in TMC-1, the molecule is not detected. To derive the upper limit to the column density, we used the brightest transitions according to the simulated LTE that appear unblended with emission from other molecules. These transitions fall in the Q-band survey performed with the Yebes telescope, and are the 61,6–50,5 and 70,7–61,6 transitions (left and right panels of Fig. 5, respectively). For the LTE simulated spectrum, we used the typical physical parameters found in this molecular cloud for many other molecules (see, e.g., Requena-Torres et al. 2008; Zeng et al. 2018): excitation temperature of Tex = 8 K, FWHM of 20 km s−1, and a radial velocity of υlsr = 68 km s−1. We obtained а 3σ upper limit of NT ≤ 2.7 × 1013cm−2, which translates into a molecular abundance compared to molecular hydrogen ≤2.0 × 10−10, using N(H2) = 1.35 × 1023 cm−2 (Martin et al. 2008). To provide a reference, the column density derived for AHA is of the same order (a factor of ~2 lower) as that derived for its isomer glycine (conformer I) toward this cloud assuming the same physical parameters (Jiménez-Serra et al. 2020), similarly to what was found toward TMC-1.

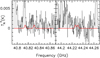thumbnail Fig. 5

Simulation of acetohydroxamic acid emission at the 3σ upper limit column density derived toward G+0.693-0.027 using the parameters outlined in the text (in red) overlaid on the observations (black line and in gray histogram). The features shown arise from the 61,6–50,5 transition (left) and 70,7–61,6 transition (right).

4 Conclusions

We presented the first high-resolution rotational study of acetohydroxamic acid (CH2C(O)NHOH), a relevant glycine isomer, using broadband and narrowband rotational spectroscopies in combination with a laser ablation vaporization device. We characterized two distinct Z- and E-conformers of AHA in the supersonic expansion. Accurate experimental values of the 14N nuclear quadrupole coupling constants together with the rotational constants and also the barrier to the internal rotation (V3) of the methyl group were provided for both conformers, in order to perfectly reproduce the spectrum to enable their identification in the ISM using low-frequency surveys. New laboratory data were subsequently employed to search for this glycine isomer toward two prominent astronomical sources, TMC-1 and G+0.693-0.027. AHA remains undetected toward both sources and, interestingly, the derived upper limit to its column density lies close to that of glycine. Our results constrain the abundance of AHA in the ISM and provide additional insights into the chemistry of amino acid-related species in space. Moreover, these data can be confidently employed in future searches using new and even more sensitive spectral line surveys at low frequencies.

Acknowledgements

The authors thank the financial fundings from Ministerio de Ciencia e Innovación (PID2019-111396GB-I00), the Ministerio de Economia Industria y Competitividad (Grant AYA2017-87515-P) Junta de Castilla y León (Grants VA010G18 and Grant VA244P20), and the European Research Council under the European Union's Seventh Framework Programme (FP/2007–2013)/ERC-2013-SyG, Grant Agreement no. 610256 NANOCOSMOS. V.M.R. has received funding from the Comunidad de Madrid through the Atracción de Talento Investigador (Doctores con experiencia) Grant (COOL: Cosmic Origins Of Life; 2019-T1/TIC-15379) and from the Agencia Estatal de Investigación (AEI) and the Ayuda RYC2020-029387-I funded by MCIN/AEI/10.13039/501100011033. I.J.-S. has received partial support from the Spanish State Research Agency (AEI; project number PID2019-105552RB-C41).The National Radio Astronomy Observatory is a facility of the National Science Foundation operated under cooperative agreement by Associated Universities, Inc.

Appendix A Complementary Tables

In Table A.1 we present the results from the XIAM A/E fit for Z- and E-AHA. In Table A.2 we provide the results from the effective single-state fit, as implemented in the SPFIT program (Pickett 1991) for both conformers. In Table A.3 and Table A.4 we provide a sample list of the measured transition frequencies for the E-symmetry state of Z-AHA and for both A- and E- states of E-AHA, respectively.

Table A.1

Experimental spectroscopic parameters obtained from the XIAM A/E fit of Z- and E-AHA (A-Reduction, Ir-Representation for the Z-conformer).

Table A.2

Experimental spectroscopic parameters for the effective single-state fit of Z- and E-AHA (A-Reduction, Ir-Representation for the Z-conformer).

Table A.3

Sample list of the measured transition frequencies for the E-symmetry state of Z-AHA.

Table A.4

Sample list of the measured transition frequencies for the A- and E-symmetry states of E-AHA using the LA-CP-FTMW spectrometer.

References

  1. Alonso, J.L., & Lopez, J.C. 2015, Microwave Spectroscopy of Biomolecular Building Blocks, eds. A.M. Rijs, & J. Oomens (Cham: Springer International Publishing), 335 [Google Scholar]
  2. Alonso, E.R., Kolesnikova, L., & Alonso, J.L. 2017, J. Chem. Phys., 147, 124312 [CrossRef] [Google Scholar]
  3. Alonso, E.R., Kolesnikova, L., Bialkowska-Jaworska, E., et al. 2018, ApJ, 861, 70 [NASA ADS] [CrossRef] [Google Scholar]
  4. Alonso, E., Leon, I., & Alonso, J. 2021, The role of the Intramolecular Interactions in the Structural Behavior of Biomolecules: Insights from Rotational Spectroscopy, 93 [Google Scholar]
  5. Altwegg, K., Balsiger, H., Bar-Nun, A., et al. 2016, Sci. Adv., 2, e1600285 [NASA ADS] [CrossRef] [Google Scholar]
  6. Barnum, T.J., Siebert, M.A., Lee, K.L.K., et al. 2022, J. Phys. Chem. A, 126, 2716 [NASA ADS] [CrossRef] [Google Scholar]
  7. Belloche, A., Meshcheryakov, A.A., Garrod, R.T., et al. 2017, A&A, 601, A49 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  8. Belloche, A., Garrod, R.T., Müller, H.S.P., et al. 2019, A&A, 628, A10 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  9. Bizzocchi, L., Prudenzano, D., Rivilla, V.M., et al. 2020, A&A, 640, A98 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  10. Bracher, B.H., & Small, R.W.H. 1970, Acta Crystallogr. B, 26, 1705 [CrossRef] [Google Scholar]
  11. Bugg, T. 2014, ChemBioChem, 15, 2467 [CrossRef] [Google Scholar]
  12. Cernicharo, J., Kisiel, Z., Tercero, B., et al. 2016, A&A, 587, L4 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  13. Cernicharo, J., Agundez, M., Cabezas, C., et al. 2021, A&A, 656, A21 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  14. Colzi, L., Rivilla, V.M., Beltran, M.T., et al. 2021, A&A, 653, A129 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  15. Cunningham, M.R., Jones, P.A., Godfrey, P.D., et al. 2007, MNRAS, 376, 1201 [NASA ADS] [CrossRef] [Google Scholar]
  16. Das, P., Gupta, G., Velu, V., et al. 2017, Biomed. Pharmacother., 96, 361 [CrossRef] [Google Scholar]
  17. Dobashi, K., Shimoikura, T., Nakamura, F., et al. 2018, ApJ, 864, 82 [NASA ADS] [CrossRef] [Google Scholar]
  18. Dobashi, K., Shimoikura, T., Ochiai, T., et al. 2019, ApJ, 879, 88 [NASA ADS] [CrossRef] [Google Scholar]
  19. Ehrenfreund, P., Bernstein, M.P., Dworkin, J.P., Sandford, S.A., & Allamandola, L.J. 2001, ApJ, 550, L95 [CrossRef] [Google Scholar]
  20. Foley, H.M. 1947, Phys. Rev., 71, 747 [NASA ADS] [CrossRef] [Google Scholar]
  21. Gerhard, D., Hellweg, A., Merke, I., et al. 2003, J. Mol. Spectrosc., 220, 234 [NASA ADS] [CrossRef] [Google Scholar]
  22. Gordy, W., & Cook, R.L. 1984, Microwave Molecular Spectra, 3rd edn. (New York: Wiley) [Google Scholar]
  23. Gratier, P., Majumdar, L., Ohishi, M., et al. 2016, ApJS, 225, 25 [Google Scholar]
  24. Groner, P., Winnewisser, M., Medvedev, I.R., et al. 2007, ApJS, 169, 28 [CrossRef] [Google Scholar]
  25. Halfen, D.T., & Ziurys, L.M. 2011, ApJ, 730, 107 [NASA ADS] [CrossRef] [Google Scholar]
  26. Hartwig, H., & Dreizler, H. 1996, Z. Naturfor. A, 51, 1099 [NASA ADS] [CrossRef] [Google Scholar]
  27. Hollis, J.M., Pedelty, J.A., Snyder, L.E., et al. 2003, ApJ, 588, 353 [NASA ADS] [CrossRef] [Google Scholar]
  28. Hollis, J.M., Lovas, F.J., Remijan, A.J., et al. 2006, ApJ, 643, L25 [NASA ADS] [CrossRef] [Google Scholar]
  29. Hoy, A.R., Mills, I.M., & Strey, G. 1972, Mol. Phys., 24, 1265 [NASA ADS] [CrossRef] [Google Scholar]
  30. Jiménez-Serra, I., Vasyunin, A.I., Caselli, P., et al. 2016, ApJ, 830, L6 [CrossRef] [Google Scholar]
  31. Jiménez-Serra, I., Martin-Pintado, J., Rivilla, V.M., et al. 2020, Astrobiology, 20, 1048 [CrossRef] [Google Scholar]
  32. Jimenez-Serra, I., Rodriguez-Almeida, L.F., Martin-Pintado, J., et al. 2022, A&A, 663, A181 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  33. Jones, P.A., Cunningham, M.R., Godfrey, P.D., & Cragg, D.M. 2007, MNRAS, 374, 579 [NASA ADS] [CrossRef] [Google Scholar]
  34. Kisiel, Z., Pszczolkowski, L., Bialkowska-Jaworska, E., & Charnley, S.B. 2007, J. Mol. Spectrosc., 241, 220 [NASA ADS] [CrossRef] [Google Scholar]
  35. Kisiel, Z., Kolesnikova, L., Belloche, A., et al. 2022, A&A, 657, A99 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  36. Kolesniková, L., Alonso, E.R., Mata, S., & Alonso, J.L. 2017, ApJS, 229, 26 [CrossRef] [Google Scholar]
  37. Kolesniková, L., Alonso, E.R., Tercero, B., Cernicharo, J., & Alonso, J.L. 2018, A&A, 616, A173 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  38. Kolesniková, L., Leon, I., Alonso, E.R., Mata, S., & Alonso, J.L. 2019, J. Phys. Chem. Lett., 10, 1325 [CrossRef] [Google Scholar]
  39. Kraśnicki, A., Pszczolkowski, L., & Kisiel, Z. 2010, J. Mol. Spectrosc., 260, 57 [CrossRef] [Google Scholar]
  40. Lee, K.L.K., Loomis, R.A., Xue, C., El-Abd, S., & McGuire, B.A. 2021, https://doi.org/10.5281/zenodo.5092150 [Google Scholar]
  41. León, I., Alonso, E.R., Mata, S., & Alonso, J.L. 2021, J. Phys. Chem. Lett., 12, 6983 [CrossRef] [Google Scholar]
  42. Ligterink, N.F.W., El-Abd, S.J., Brogan, C.L., et al. 2020, ApJ, 901, 37 [NASA ADS] [CrossRef] [Google Scholar]
  43. Ligterink, N.F.W., Ahmadi, A., Luitel, B., et al. 2022, ACS Earth Space Chem., 6, 455 [NASA ADS] [CrossRef] [Google Scholar]
  44. Marmion, C., Griffith, D., & Nolan, K. 2004, Eur. J. Inorg. Chem., 2004, 3003 [CrossRef] [Google Scholar]
  45. Martin, S., Requena-Torres, M.A., Martin-Pintado, J., & Mauersberger, R. 2008, ApJ, 678, 245 [CrossRef] [Google Scholar]
  46. Martin, S., Martin-Pintado, J., Blanco-Sanchez, C., et al. 2019, A&A, 631, A159 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  47. McCarthy, M.C., & McGuire, B.A. 2021, J. Phys. Chem. A, 125, 3231 [NASA ADS] [CrossRef] [Google Scholar]
  48. McGuire, B.A., Burkhardt, A.M., Loomis, R.A., et al. 2020, Astrophys. J. Lett., 900, L10 [NASA ADS] [CrossRef] [Google Scholar]
  49. McGuire, B.A., Loomis, R.A., Burkhardt, A.M., et al. 2021, Science, 371, 1265 [NASA ADS] [CrossRef] [Google Scholar]
  50. Miller, M.J. 1989, Chem. Rev., 89, 1563 [CrossRef] [Google Scholar]
  51. Mori, T., Suma, K., Sumiyoshi, Y., & Endo, Y. 2009, J. Chem. Phys., 130, 204308 [NASA ADS] [CrossRef] [Google Scholar]
  52. Muri, E.M., Nieto, J., & Sindelar, R.D. 2002, Curr. Med. Chem., 9, 1631 [CrossRef] [Google Scholar]
  53. Ozeki, H., Miyahara, R., Ihara, H., et al. 2017, A&A, 600, A44 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  54. Pal, D., & Saha, S. 2012, J. Adv. Pharmaceut. Technol. Res., 3, 92 [CrossRef] [Google Scholar]
  55. Peña, I., Mata, S., Martin, A., et al. 2013, Phy. Chem. Chem. Phys. (Incorp. Faraday Trans.), 15, 18243 [CrossRef] [Google Scholar]
  56. Pickett, H.M. 1991, J. Mol. Spectrosc., 148, 371 [NASA ADS] [CrossRef] [Google Scholar]
  57. Pickett, H.M., Poynter, R.L., Cohen, E.A., et al. 1998, J. Quant. Spectrosc. Radiative Transfer, 60, 883 [NASA ADS] [CrossRef] [Google Scholar]
  58. Requena-Torres, M.A., Martin-Pintado, J., Martin, S., & Morris, M.R. 2008, ApJ, 672, 352 [NASA ADS] [CrossRef] [Google Scholar]
  59. Rivilla, V.M., Martin-Pintado, J., Jiménez-Serra, I., et al. 2019, MNRAS, 483, L114 [NASA ADS] [CrossRef] [Google Scholar]
  60. Rivilla, V.M., Martin-Pintado, J., Jiménez-Serra, I., et al. 2020, ApJ, 899, L28 [NASA ADS] [CrossRef] [Google Scholar]
  61. Rivilla, V.M., Jiménez-Serra, I., Garcia de la Concepcion, J., et al. 2021a, MNRAS, 506, L79 [NASA ADS] [CrossRef] [Google Scholar]
  62. Rivilla, V.M., Jiménez-Serra, I., Martin-Pintado, J., et al. 2021b, Proc. Natl. Acad. Sci. U.S.A., 118, 2101314118 [NASA ADS] [CrossRef] [Google Scholar]
  63. Rivilla, V.M., Colzi, L., Jiménez-Serra, I., et al. 2022a, ApJ, 929, L11 [NASA ADS] [CrossRef] [Google Scholar]
  64. Rivilla, V.M., Garda de la Concepcion, J., Jiménez-Serra, I., et al. 2022b, Front. Astron. Space Sci., 9, 829288 [NASA ADS] [CrossRef] [Google Scholar]
  65. Robinson, G.W., & Cornwell, C.D. 1953, J. Chem. Phys., 21, 1436 [NASA ADS] [CrossRef] [Google Scholar]
  66. Rodríguez-Almeida, L.F., Jiménez-Serra, I., Rivilla, V.M., et al. 2021a, ApJ, 912, L11 [CrossRef] [Google Scholar]
  67. Rodríguez-Almeida, L.F., Rivilla, V.M., Jiménez-Serra, I., et al. 2021b, A&A, 654, A1 [Google Scholar]
  68. Rubin, R.H., Swenson, G.W.J., Benson, R.C., Tigelaar, H.L., & Flygare, W.H. 1971, ApJ, 169, L39 [NASA ADS] [CrossRef] [Google Scholar]
  69. Saldyka, M., & Mielke, Z. 2004, J. Mol. Struct., 692, 163 [NASA ADS] [CrossRef] [Google Scholar]
  70. Saldyka, M., & Mielke, Z. 2018, J. Phys. Chem. A, 122, 60 [NASA ADS] [CrossRef] [Google Scholar]
  71. Sanz-Novo, M., Largo, A., Redondo, P., & Barrientos, C. 2019, ACS Earth Space Chem., 3, 1170 [NASA ADS] [CrossRef] [Google Scholar]
  72. Sanz-Novo, M., Belloche, A., Alonso, J.L., et al. 2020, A&A, 639, A135 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  73. Snyder, L.E., Lovas, F.J., Hollis, J.M., et al. 2005, ApJ, 619, 914 [CrossRef] [Google Scholar]
  74. Turner, B.E. 1991, ApJS, 76, 617 [NASA ADS] [CrossRef] [Google Scholar]
  75. Watson, J.K.G. 1977, in Vibrational Spectra and Structure, ed. J.R. Durig, (Amsterdam: Elsevier), 6, 1 [Google Scholar]
  76. Xue, C., Willis, E.R., Loomis, R.A., et al. 2020, ApJ, 900, L9 [NASA ADS] [CrossRef] [Google Scholar]
  77. Zeng, S., Jiménez-Serra, I., Rivilla, V.M., et al. 2018, MNRAS, 478, 2962 [NASA ADS] [CrossRef] [Google Scholar]
  78. Zeng, S., Jiménez-Serra, I., Rivilla, V.M., et al. 2021, ApJ, 920, L27 [NASA ADS] [CrossRef] [Google Scholar]
1

Madrid Data Cube Analysis on ImageJ is a software developed at the Center of Astrobiology (CAB) in Madrid; http://cab.inta-csic.es/madcuba/

All Tables

Table 1

Theoretical and experimental spectroscopic parameters for the A-symmetry state of the observed conformers of AHA (A-Reduction, Ir-representation).

In the text
Table 2

Sample list of the measured transition frequencies for the A-symmetry state of AHA.

In the text
Table 3

Rotational and vibrational partition functions of Z-AHA.

In the text
Table A.1

Experimental spectroscopic parameters obtained from the XIAM A/E fit of Z- and E-AHA (A-Reduction, Ir-Representation for the Z-conformer).

In the text
Table A.2

Experimental spectroscopic parameters for the effective single-state fit of Z- and E-AHA (A-Reduction, Ir-Representation for the Z-conformer).

In the text
Table A.3

Sample list of the measured transition frequencies for the E-symmetry state of Z-AHA.

In the text
Table A.4

Sample list of the measured transition frequencies for the A- and E-symmetry states of E-AHA using the LA-CP-FTMW spectrometer.

In the text

All Figures

thumbnail Fig. 1

Structures of the AHA conformers, showing the presence of a 14N nucleus. V3 is the barrier height associated to the methyl internal rotation motion. Color-coding: carbon atoms are in grey; nitrogen atoms are in blue, oxygen atoms are in red, and hydrogen atoms are in white.
In the text
thumbnail Fig. 2

Rotational spectrum of AHA. (a) Broadband LA-CP-FTMW spectrum of AHA from 6.8 to 14.2 GHz. Strong a-type R-branch (J + 1)0,J+1 ← J1,j and (J + 1)1,J+1 ← J0,J transitions of rotamer I (Z-AHA, in blue) are shown, as well as some transitions of rotamer II (E-AHA, in red). (b) Zoom-in on the broadband spectrum showing the 10,1 ← 00,0 rotational transition for both acetohydroxamic acid species highlighting the A–E doublets due to the internal rotation of the methyl group. The intensity is given in arbitrary units.
In the text
thumbnail Fig. 3

Partially resolved hyperfine pattern for the 30,3 ← 21,2 transition of the A-symmetry ground state of Z-AHA (LA-CP-FTMW experiment) and completely resolved hyperfine structure using the cavity-based (LA-MB-FTMW) experiment, where each transition appears as Doppler doublets due to the sub-Doppler resolution of the spectrometer. The simulated spectrum is also depicted for comparison (in blue). The intensity is given in arbitrary units.
In the text
thumbnail Fig. 4

Simulation of acetohydroxamic acid emission at the 3σ upper limit column density derived toward TMC-1 using the parameters outlined in the text (in red) overlaid on the GOTHAM observations of TMC-1 (in black). The features shown arise from the 31,з–20,2 set of transitions. The spectra have been adjusted to the nominal source υlsr = 5.8 km s−1.
In the text
thumbnail Fig. 5

Simulation of acetohydroxamic acid emission at the 3σ upper limit column density derived toward G+0.693-0.027 using the parameters outlined in the text (in red) overlaid on the observations (black line and in gray histogram). The features shown arise from the 61,6–50,5 transition (left) and 70,7–61,6 transition (right).
In the text

Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.

Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.

Initial download of the metrics may take a while.