EDP Sciences logo
Subscriber Authentication Point
Search
Menu
Home All issues Volume 520 (September-October 2010) A&A, 520 (2010) L1 Full HTML
Free Access
Issue
A&A
Volume 520, September-October 2010
Article Number L1
Number of page(s) 5
Section Letters
DOI https://doi.org/10.1051/0004-6361/201015212
Published online 22 September 2010
A&A 520, L1 (2010)

LETTER TO THE EDITOR

Gamma ray emission from magnetized relativistic GRB outflows

A. Neronov - V. Savchenko

ISDC Data Center for Astrophysics, Chemin d'Écogia 16, 1290 Versoix, Switzerland and Geneva Observatory, 51 Ch. des Maillettes, 1290 Sauverny, Switzerland

Received 15 June 2010 / Accepted 19 July 2010

Abstract
We argue that small pitch angle synchrotron emission provides an important dissipation mechanism that has to be taken into account in models of the formation of relativistic magnetized $\gamma$-ray burst (GRB) outflows from newborn black holes and/or magnetars. We show that if the GRB outflow is proton loaded, the spectral energy distribution of this emission is expected to sharply peak in the 0.1-1 MeV energy band. If the small pitch-angle synchrotron emission efficiently cools relativistic particles of the outflow, the emission spectrum below the peak energy is a power law with spectral index $\alpha\simeq -1$, close to the typical spectral index of time-resolved GRB spectra. Otherwise, the low energy spectral index can be as hard as $\alpha\simeq 0$, as observed at the beginning of the GRB pulses. We conjecture that small pitch-angle synchrotron emission from proton-loaded magnetized GRB outflow could significantly contribute to the Band component of the prompt emission of GRBs, while electromagnetic cascade initiated by the protons may be responsible for the GeV component.

Key words: gamma-ray burst: general - radiation mechanisms: non-thermal

1 Introduction

A large amount of information about the time evolution of the spectral characteristics of prompt emission of $\gamma$-ray bursts (GRB) has been collected by CGRO/BATSE, Swift/BAT, INTEGRAL/ISGRI (see Mészáros 2006; and Piran 2004, for reviews) and, the Fermi/GBM (Band et al. 2009) telescopes. It is firmly established that the majority of the time-resolved GRB spectra could be well described by relatively simple spectral models, such as the Band model (Band et al. 1993), or a cut-off or broken power-law model determined by three main parameters: low and high energy photon indices $\alpha$ and $\beta$, and peak (or break or cut-off) energy $E_{\rm p}$ (Piran 2004; Mészáros 2006).

The observed distribution of the low-energy power-law indices $\alpha$ (of differential photon spectra given by ${\rm d}N_\gamma/{\rm d}E\sim E^{\alpha}$) of time-resolved GRB spectra is sharply peaked at the value $\alpha\simeq -1$ in the BATSE GRB (Band et al. 1993; Kaneko et al. 2006) and Swift/BAT (Savchenko & Neronov 2009) GRB samples. Deviations from this mean value toward $\alpha>-1$ (down to $\alpha\simeq 0$) are preferentially observed at the initial moments of onset of GRB sub-pulses, when the spectra could be as hard as $\alpha\ga 0$ (Preece et al. 2000; Savchenko & Neronov 2009; Kaneko et al. 2006). Evolution toward $\alpha<-1$ is commonly observed during the decay of individual sub-pulses and/or at the end of the prompt GRB emission phase. The characteristic value $\alpha\simeq -1$ is difficult to reconcile with the conventionally assumed synchrotron and/or inverse Compton mechanisms of prompt GRB emission. Alternative models, which attempt to resolve this problem, include ``jitter'' radiation (Medvedev 2006; Medvedev & Loeb 1999) (see Kirk & Reville 2009, for a discussion of the potential problems of the jitter radiation model) and ``quasi thermal comptonization'' (Ghisellini & Celotti 1999) models.

Spectra of at least some GRBs contain a separate GeV-band component (e.g., Abdo et al. 2009a,b). The GeV emission has a different (relative to the Band component) temporal evolution (Ghisellini et al. 2010). The absence of the high-energy cutoff in the spectrum of this GeV component due to the pair-production imposes a lower bound on the bulk Lorentz factors of the GRB outflows (about $\Gamma \ga 1000$, e.g. Baring & Harding 1997; Abdo et al. 2009a). Different models of the origin of the GeV emission have been proposed, such as high-energy extention of the conventional afterglow (Kumar & Duran 2009; Ghirlanda et al. 2009), afterglow emission from a fireball in the radiative regime (Ghisellini et al. 2010), and synchrotron (Razzaque et al. 2009) or cascade (Dermer & Atoyan 2006; Asano et al. 2009) emission from ultra high-energy ( ${\sim}10^{20}$ eV) protons.

Several models of the formation of relativistic GRB outflows from black holes or neutron stars, formed in result of core collapse of massive stars, assume that the outflow is highly magnetized (Komissarov & Barkov 2007; Bucciantini et al. 2009,2007; Komissarov et al 2007; Komissarov et al. 2009). In these models, the energy of the outflow is initially carried by the Poynting flux of electromagnetic energy, so that the ratio of electromagnetic to kinetic energy of the outflow, $\sigma$, is comparable to or much higher than unity. Numerical MHD calculations show that the electromagnetic energy could be converted in a dissipationless way to the kinetic energy of relativistic particle outflow. Dissipation is absent because the electromagnetic field forms a force-free configuration of electromagnetic field, ${\vec E}+[{\vec V}\times {\vec B}]=0$, where $\vec E,\vec B$ are the electric and magnetic fields and $\vec V$ is the flow velocity.

In what follows we show that even if electromagnetic field is force-free, the energy loss of relativistic particles in the outflow is non-zero, because the directions of the velocities of individual particles are scattered around the average direction of the flow within a certain angle $\Psi_0\sim \Gamma_0^{-1}$, where $\Gamma_0$ is the Lorentz factor of the outflow. This scatter in particle velocities leads to radiative dissipation of the outflow energy, the main dissipation mechanism being small pitch-angle synchrotron emission. We demonstrate that a significant fraction of the power of magnetized outflow could be dissipated by this mechanism even in the outflow acceleration region.

We derive the spectral characteristics of this emission and show that they are consistent with those of the observed GRB prompt emission spectra. The peak energy of the spectrum is expected to be in the 0.1-1 MeV range for the outflows with typical GRB parameters (bulk Lorentz factors, isotropic luminosities). The low-energy spectral index is expected to evolve from a very hard value $\alpha\simeq 0$ toward a ``steady state'' value $\alpha=-1$, which is expected to persist over the period of stable activity of GRB central engine and/or up to the moment when interactions of the high-energy protons with the synchrotron-cooled lower energy protons begin to contribute significantly to the energy loss. By comparing the spectral characteristics of proton synchrotron emission with those observed in the prompt emission $\gamma$-ray spectra of GRBs, we conjecture that emission from the pre-shock outflow dominates during the prompt emission phase. We discuss possible observational tests that would allow to test this conjecture.

2 Generic properties of pre-shock relativistic GRB outflow

If the observed isotropic luminosity of GRB is $L_{\rm iso}$, the energy flux carried by the GRB outflow ejected into a solid angle $\Omega$ is $L=(\Omega/4\pi)L_{\rm iso}\simeq 8$ $\times$ $10^{47}\left[L_{\rm iso}/10^{52}~{\rm erg~s^{-1}}\right] \left[\Theta/1^\circ\right]^2~{\rm erg~s^{-1}}$, where $\Theta$ is the opening angle of the outflow. The energy density of the outflow at a distance D is $U=L/(\Omega D^2)$[*]. It is convenient to parametrize the strength of the electromagnetic field in the outflow in terms of magnetization parameter $\sigma$, $B^2/(8\pi)\sim\sigma U/(1+\sigma)$. Using this a parametrization, the (electro)magnetic field strength at the distance D can be expressed as $B=\sqrt{2\sigma L_{\rm iso}/(1+ \sigma)}/D\simeq 10^{13}\left[\sigma/(1+\sigma)... ...rm iso}/10^{52}~{\rm erg~s^{-1}}\right]^{1/2} \left[D/10^8~{\rm cm}\right]^{-1}$ G.

The minimal possible angular scatter in the velocities of the particles is within a cone of opening angle $\Psi_0\simeq \kappa \Gamma_0^{-1}$ ($\kappa $ is a numerical factor of the order of 1). The non-zero angular scatter in the particle velocities around the bulk velocity $\vec V$ leads to the appearance of non-zero Lorentz force of the order of $F\sim eB_\bot$, where e is particle charge and $B_\bot\sim B\Psi_0=\kappa B\Gamma_0^{-1} \simeq 10^{9}\kappa\left[\sigma/(1\!\!... ...2}\!\left[D/\!10^8~\mbox{cm}\!\right]^{-1}\!\left[\Gamma_0/10^{4}\!\right]^{-1}$ G.

Particle motions due to this uncompensated Lorentz force lead to radiation. To calculate the properties of this radiation, it is convenient to go into the reference frame moving with velocity ${\cal V}=\left[{\vec E}\times {\vec B}\right]/\left\vert B\right\vert^2$. In this reference frame, the electric component of electromagnetic field is zero, the mean outflow velocity $\vec V'$ is aligned with the magnetic field $\vec B'$, and the radiation is synchrotron radiation of particles moving at small pitch angles in the magnetic field[*]. Unless $\vec V$ is orthogonal to $\vec B$, the velocity of the new frame ${\cal V}\ll c$. Taking this into account, we do not distinguish the quantities (such as photon energies) in different reference frames in the order-of-magnitude estimates presented below.

Properties of synchrotron emission from particles moving at small pitch angles with respect to the magnetic field significantly differ from those of synchrotron emission from an isotropic particle distribution (Epstein 1973; Lloyd & Petrosian 2000). The typical energy of the radiated photons, $\epsilon_{\rm s}\sim eB_\bot \Gamma_0^2/m_{\rm p}$

\begin{displaymath}% \epsilon_{\rm s} \!\simeq\! 6\times 10^{5}\kappa\left[\frac... ...}}\right]^{-1}\! \left[\frac{\Gamma_0}{10^4}\right]~\mbox{eV}, \end{displaymath} (1)

where $m_{\rm p}$ is the particle mass (proton mass in the above and in the following numerical estimates), scales proportionally to the particle energy, rather than to the square of it. The synchrotron energy loss rate $P_{\rm s}= 2e^4B_\bot^2\Gamma_0^2/(3m_{\rm p}^2)\sim \kappa^2B^2$ does not depend on the particle energies  $\Gamma_0m_{\rm p}$.

To estimate the importance of small pitch angle synchrotron cooling as a dissipation mechanism, one has to compare the synchrotron cooling distance $D_{\rm s}=\Gamma_0m_{\rm p}/P_{\rm s}\simeq 10^7\kappa^{-2} [\sigma/(1$ + $\sigma)]^{-1}\left[L_{\rm iso}/10^{52}~{\rm erg~s^{-1}}\right]^{-1} \left[D/10^8~{\rm cm}\right]^{2}\left[\Gamma_0/10^{4}\right]~{\rm cm}$ with the typical distance scales of formation and propagation of the GRB outflow. Equating $D_{\rm s}$ to D one can find that synchrotron emission can efficiently remove energy from relativistic protons out to the distance

\begin{displaymath}% {\cal D}_{\rm s}\simeq 1.3\times 10^{9}\kappa^2\left[\frac{... ...1}}}\right]\left[\frac{\Gamma_0}{10^{4}}\right]^{-1}~{\rm cm}. \end{displaymath} (2)

The typical distance scale of the GRB central engine $R_{\rm CE}\sim 10^6{-}10^7$ cm is given by the size of black hole formed in the collapse of a massive star, or the radius of the magnetar. The region of acceleration of the GRB outflow to its asymptotic Lorentz factor $\Gamma_0$ might be of a much larger size, e.g.  $D_{\Gamma}\sim \Gamma_0R_{\rm CE}$ in the ``fireball'' model (Mészáros 2006). A similar estimate of the size of the GRB outflow acceleration region is found in the ``magnetic acceleration'' type models (Komissarov et al. 2009; Komissarov et al 2007). Both  $R_{\rm CE}$ and $D_\Gamma$ might be ${\la}{\cal D}_{\rm s}$ implying that small pitch-angle synchrotron emission could be an efficient dissipation mechanism.

In the fireball model, it is commonly assumed that the bulk of the power of the GRB outflow is transferred from the region of the outflow formation to the internal shock region by relativistic protons, while the fraction of the power carried by electrons is smaller by a factor ${\sim}m_{\rm e}/m_{\rm p}\simeq 10^{-3}$ (Mészáros 2006). The power of the proton-loaded outflow is transferred efficiently to electrons only in the internal shock region, which is normally at large distances $D_{\rm shock}\sim \Gamma^2R_{\rm CE}\gg {\cal D}_{\rm s}$. In the unshocked outflow, electrons are affected, in a similar way to protons, by the energy loss related to the small pitch angle synchrotron emission. However, the energy losses of electrons do not contribute significantly to the energy dissipation in the distance range $D_\Gamma<D<D_{\rm shock}$.

Synchrotron photons may freely escape from the GRB outflow if they are produced at sufficiently large distances, where the GRB outflow is optically thin with respect to Compton scattering. The mean free path of photons with respect to the Compton scattering is $\lambda_{\rm C}\simeq \left[n\sigma_{\rm T}(1-\cos(\Psi_0))\right]^{-1}\simeq2\... ...o}/10^{52}~{\rm erg~s^{-1}}\right]^{-1} \left[\Gamma_0/10^4\right]^{3}~{\rm cm}$, where $\sigma_{\rm T}$ is Thomson cross-section and $n=\left.L_{\rm iso}\right/(4\pi D^2 m_{\rm p}\Gamma_0)$ is the electron density of the outflow (we assume that the number density of electrons is equal to the number density of protons). The outflow becomes optically thin at the distance at which $\lambda_{\rm C}\simeq D$ given by

\begin{displaymath}% {\cal D}_{\rm C}\simeq 5\times 10^{6}\left[\frac{L_{\rm iso... ...{-1}}}\right]\left[\frac{\Gamma_0}{10^4}\right]^{-3}~{\rm cm}. \end{displaymath} (3)

In GRBs with bulk Lorentz factors $\Gamma_0\ge 6\times 10^2$, ${\cal D}_{\rm C}<{\cal D}_{\rm s}$ and synchrotron photons freely escape if they are produced in the distance range ${\cal D}_{\rm C}<D<{\cal D}_{\rm s}$. In the distance range $D<{\cal D}_{\rm C}$, the spectrum of the proton synchrotron radiation is modified by the small angle Compton scattering on (energetically sub-dominant) electrons present in the outflow.

3 Spectrum of emission from pre-shock proton loaded GRB outflow

This type of spectrum could be calculated by summing the spectra emitted from the outflow components moving at different angles $\theta$ with respect to the line of sight

\begin{displaymath}% F_{\Gamma_0}(\nu)=\frac{1}{\Psi_0^2}\int \theta {\rm d}\theta \int_0^{\Psi_0}\Psi {\rm d}\Psi F_{\Gamma_0,\theta}(\nu,\Psi), \end{displaymath} (4)

where $F_{\Gamma_0,\theta}(\nu,\Psi)$ is emission from particles spiraling at pitch angle $\Psi$ within a beam with an opening angle $\Psi_0= \kappa\Gamma_0^{-1}$ viewed at an angle $\theta$ with respect to the direction of the beam. A non-negligible contribution to the integral over $\theta$ is given only by $\theta$ in the range $0<\theta<(\Psi_0+\Gamma^{-1})$. We note that we have assumed, for simplicity, that the parameters of the outflow do not depend on the angle with respect to the axis of the outflow, i.e., the Lorentz factor $\Gamma_0$ and particle density n are constant inside a cone of large enough opening angle $\Theta\gg \Gamma^{-1}$. The generalization of the calculations presented below to the case of non-trivial angular profile of the GRB outflow is straightforward.

The parameter $F_{\Gamma_0,\theta}(\nu,\Psi)$ in Eq. (4) could be expressed as (Epstein 1973; Ginzburg & Syrovatskii 1969)

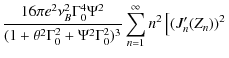
                      $\displaystyle F_{\Gamma_0,\theta}(\nu,\Psi)$ $\textstyle \simeq$ $\displaystyle \frac{16\pi e^2\nu_B^2\Gamma_0^4\Psi^2}{(1+\theta^2\Gamma_0^2+\Psi^2\Gamma_0^2)^3}\sum_{n=1}^\infty n^2\left[(J'_n(Z_n))^2\right.$  
    $\displaystyle \left.+~\left\{\frac{1-\theta^2\Gamma_0^2+\Psi^2\Gamma_0^2}{2\theta\Psi\Gamma_0^2}J_n(Z_n)\right\}^2\right] \delta(\nu-\nu_n),$  

where $\nu_B=eB/(2\pi m)$ is the cyclotron frequency, $\nu_n=2n\Gamma_0\nu_B/(1+\theta^2\Gamma_0^2+\Psi^2\Gamma_0^2)$, $Z_n=2n\Gamma_0^2\theta\Psi/(1+\theta^2\Gamma_0^2+\Psi^2\Gamma_0^2)$, and Jn(z) are Bessel functions. Substituting the above expression into Eq. (4), one finds

\begin{displaymath}% F_{\Gamma_0}(\nu)=\frac{4\pi e^2\nu_B }{\kappa^2\Gamma_0}{\cal F}(\tilde\nu,\kappa), \end{displaymath} (5)

where $\tilde\nu=\nu/(2\Gamma_0\nu_B)$ and ${\cal F}(\tilde \nu,\kappa)$ is a function that depends on neither the magnetic field strength nor the Lorentz factor. Numerically calculated functions  ${\cal F}(\tilde \nu,\kappa)$ are shown for several values of $\kappa $ in Fig. 1. At low frequencies,  $\tilde\nu\ll 1$, the function  ${\cal F}_\gamma(\tilde\nu,\kappa)$ has the form of a hard spectral index power-law, ${\cal F}_\gamma(\tilde\nu,\kappa)\sim \tilde\nu^{\alpha+1}$ with $\alpha=0$. The spectrum becomes even harder close to the Doppler shifted cyclotron frequency $\tilde\nu=1$. If $\kappa<1$, the function ${\cal F}(\tilde \nu,\kappa)$ has more-or-less strong higher harmonics of the cyclotron line, which can be seen as multiple ``bumps'' in the spectrum at $\tilde\nu\ge 1$.

\begin{figure} \par\includegraphics[width=8.8cm,clip]{15212fg1} \end{figure} Figure 1:

Spectra of small pitch-angle proton synchrotron emission from pre-shock GRB outflow for several values of $\kappa $. Thick solid line shows an emission spectrum from a proton spectrum formed by cooling of the outflow protons to $\Gamma =0.01\Gamma _0$. For comparison, we show Band model fits to the spectra of periods ``b'' (beginning) and ``d+e'' (end) of Fermi GRB 090902B (Abdo et al. 2009b) as lower and upper grew curves. Normalizations and peak energies of the Band model fits to GRB 090902B spectra are shifted to match the small pitch-angle synchrotron model curves.

Open with DEXTER

4 High-energy emission from the ``tail'' of angular distribution

The spectrum marked $\kappa=1$ in Fig. 1 is calculated based on the assumption that the angular distribution of particle velocities,  $N_{\rm p}(\Psi)$, is cut-off at the angle $\Psi_0\simeq \Gamma_0^{-1}$. In general, $N_{\rm p}(\Psi)$ may exhibit large angle ``tail'' extending beyond $\Psi\simeq \Gamma_0^{-1}$. The spectrum of emission from protons moving at large pitch angles $\kappa\gg 1$ extends up to the frequency $\tilde\nu\simeq \kappa$, while the frequency below which the spectrum is characterized by $\alpha=0$ moves down to $\tilde\nu\simeq \kappa^{-2}$ (see Fig. 1). In the range $\kappa^{-2}\tilde\nu<\kappa$, the spectrum is characterized by a photon index $\alpha=-2/3$ typical of synchrotron radiation from an isotropic particle distribution (Fig. 1). If the large pitch angle tail of  $N_{\rm p}(\Psi)$ has the form of a power-law, $N_{\rm p}(\kappa\Gamma_0^{-1})\sim \kappa^{-s}$, the spectrum of emission above the characteristic (peak) frequency $\nu=2\Gamma_0\nu_B$ has the form of a power-law with photon index $\beta=s-1$. In principle, the tail of the angular distribution could extend up to $\Psi\sim 1$, such that the energy of synchrotron photons emitted by the large angle tail extends up to $\tilde\nu\sim\Gamma_0$. If the spectrum of proton synchrotron emission from particles moving at the angle $\Psi\le \Gamma_0^{-1}$ peaks in the 100 keV-1 MeV energy band, the high-energy (large pitch angle) tail of the synchrotron spectrum could extend up to the 1-10 GeV band if $\Gamma_0\sim 10^4$. The presence of the large angle tails of proton angular distribution could, therefore, be responsible for the appearance of high-energy extensions of the prompt GRB spectra similar to that observed in GRB 080916C (Abdo et al. 2009a). Additional obvious possible sources of the GRB 080916C-like high-energy tails in the GRB spectra are protons with energies higher than  $\Gamma_0m_{\rm p}$ in the outflow.

5 Emission from a distribution of protons formed by the small pitch angle synchrotron cooling

The total proton energy-loss rate could be found by evaluating the integral of  $F_{\Gamma_0}(\nu)$:

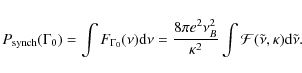
\begin{displaymath}% P_{\rm synch}(\Gamma_0)=\int F_{\Gamma_0}(\nu) {\rm d}\nu=\... ...B^2}{\kappa^2}\int {\cal F}(\tilde\nu,\kappa){\rm d}\tilde\nu. \end{displaymath} (6)

As expected, $P_{\rm synch}$ does not depend on the Lorentz factor $\Gamma_0$. Energy-independent small pitch angle synchrotron cooling leads to the generation of a low-energy tail of particle spectrum below the initial proton energy  $\Gamma_0m_{\rm p}$. During the time when the central engine of the GRB outflow continuously ejects relativistic outflow with rate $Q(\Gamma)=Q_0\delta (\Gamma-\Gamma_0)$, the spectrum of the synchrotron-cooled protons  $N_{\rm p}(\Gamma)$ is a stationary solution of continuity equation $\left.\partial\right/\partial \Gamma\left\{P_{\rm synch}(\Gamma)N_{\rm p}(\Gamma)\right\}=Q(\Gamma)$:

\begin{displaymath}% N_{\rm p}(\Gamma)=\frac{Q_0}{P_{\rm synch}(\Gamma)}\sim \Gamma^{-b},\ \ \ b=0, \end{displaymath} (7)

in the range of Lorentz factors $\Gamma\le \Gamma_0$. A spectrum of small pitch-angle synchrotron emission from such proton distribution is

\begin{displaymath}% F(\nu)=\int N_{\rm p}(\Gamma) F_\Gamma(\nu){\rm d}\Gamma\sim\nu^{\alpha+1}, \alpha=-(b+1)=-1. \end{displaymath} (8)

The overall spectral evolution of the pre-shock outflow emission is expected to be as follows. As soon as synchrotron cooling of the outflow particles becomes efficient, the spectrum of emission from the pre-shock outflow softens from $\alpha\simeq 0$ (or  $\alpha=-2/3$, depending on the initial value of $\kappa $) down to $\alpha\simeq -1$. The $\alpha\simeq -1$ spectrum persists over a period of stable activity of the GRB central engine as long as synchrotron cooling remains the dominant energy-loss channel. This value of $\alpha$ is close to the measured characteristic low-energy spectral index of CGRO/BATSE and Swift/BAT GRBs (Savchenko & Neronov 2009; Kaneko et al. 2006). Prompt emission spectra of some GRBs start from very low values of $\alpha>-2/3$ (Preece et al. 2000; Savchenko & Neronov 2009; Kaneko et al. 2006) and evolve toward $\alpha\simeq -1$ as expected if the very hard emission originates from the pre-shock GRB outflow. As an example, we show in Fig. 1 Band model fits to the spectra of the beginning and end of the prompt emission phase of the GeV-$\gamma$-ray-loud GRB 090902B, taken from (Abdo et al. 2009b). Comparing the Band model fit of the initial rising phase of GRB 090902B with the spectrum of emission from monochromatic proton distribution, one could conclude that GRB 090902B outflow is initially almost monoenergetic. Equation (1) enables us to derive initial $\Gamma_0\sim 10^4$, i.e. proton energy $\Gamma_0m_{\rm p}\sim 10^{13}$ eV, given the isotropic luminosity $E_{\rm iso}\sim 10^{53}$ erg s-1 and peak energy $E_{\rm peak}\sim \epsilon_{\rm s}\sim 10^6$ eV for this particular GRB.

Small pitch-angle synchrotron emission leads not only to cooling but also to scattering in the proton beam across a wider angle $\Psi$ $\sim$ $\Gamma^{-1}$ $\gg$ $\Gamma_0^{-1}$. The widening of the opening angle of particle distribution could lead to the ``switching on'' of interactions between the particles of the outflow. The mean free path of the highest energy protons with respect to the pp interactions with low energy protons is given by $\lambda_{pp}= \left\{\sigma_{pp}n(\Gamma) (1\!-\!\cos(\Gamma^{-1}))\right\}^{-1}$ $\simeq$ $10^{11}\left[L_{\rm iso}/10^{52}~{\rm erg~s^{-1}}\right]\left[D/10^8~{\rm cm}\right]\left[\Gamma_0/10^4\right]\left[\Gamma/10^4\right]$ cm, where $\sigma_{pp}\simeq 10^{-26}$ cm2 is the cross-section of pp interactions and $n(\Gamma)\sim \Gamma^{1-b}$ is the density of particles with gamma factor $\Gamma$. The parameter  $\lambda_{pp}$ becomes comparable or shorter than ${\cal D}_{\rm s}$ as soon as synchrotron cooling leads to the appearance of protons with energies $\Gamma\la 10^{-2}\Gamma_0$ if $\Gamma_0\sim 10^4$.

Development of proton initiated cascade leads to injection of secondary electrons/positrons (as $\gamma$-rays and well as neutrinos) which can largely outnumber the primary electrons present in the unshocked GRB outflow. In constrast to the primary electrons, synchrotron emission from these cascade electrons could provide a significant energy dissipation mechanism of the outflow. Although typical initial Lorentz factors of the secondary electrons are $\gamma_{\rm e}\sim (\Gamma m_{\rm p})/m_{\rm e}\gg \Gamma_0$, electrons are injected at the typical pitch angles $\psi_{\rm e}\sim \Gamma^{-1}\gg \gamma_{\rm e}^{-1}$, so that the synchrotron emission from the cascade electrons is not emitted in the small pitch angle regime.

The $\gamma$-rays with energies above the pair production threshold $E_{\gamma\gamma}=\sqrt{2m_{\rm e}^2c^4/(1-\cos(1/\Gamma))}\simeq 10^{10}\left[\Gamma/10^4\right]~{\rm eV}$ produce pairs in interactions among themselves and initiate the electromagnetic cascade. The highest energy $\gamma$-rays may also interact with protons via pion and pair production channels. The energy loss of protons due to the pp (and/or $p\gamma$) interactions is given by $P_{pp}(\Gamma_0)\sim f\Gamma_0/\lambda_{pp}$, where $f\sim 0.1{-}0.2$ is the typical inelasticity of pp collisions. As soon as the cooling of the highest energy protons by means of pp and $p\gamma$ interactions becomes more efficient than the synchrotron cooling, the spectrum of protons is expected to soften to b>0 (see Eq. (7)). This should lead to the softening of the small pitch angle synchrotron emission spectrum to $\alpha<-1$ and a decrease in its contribution to the overall GRB spectrum, compared to the contribution from the cascade emission component. The spectrum of emission from the proton-initiated cascade extends up to the energy of the threshold of the pair production,  $E_{\gamma\gamma}$. For the particular example of GRB 090902B, the electromagnetic cascade component is then clearly identified as the soft emission component extending up to the multi-GeV energies, which becomes dominant at the end of the prompt emission phase and persists for  ${\sim}10^3$ s after the end of the prompt emission (Abdo et al. 2009b).

Bursts that initiate with very hard spectra would be the most interesting candidates for testing the hypothesis of small pitch-angle proton synchrotron emission because they might possess the cyclotron line at the frequency $\nu\sim \Gamma_0\nu_B$. We search for this line at the energy close to the peak energy of very hard GRB spectra to test the proposed model. The cyclotron line feature may, however, have been ``washed out'' of the spectrum by a non-negligible spread in the particle energies in the outflow and by small angle Compton scattering if most of the small pitch-angle synchrotron emission is generated at the distances $D \la {\cal D}_{\rm C}$. In this case, the spectrum of emission from the pre-shock outflow would be indistinguishable from the generic Band model spectra. A complementary way of testing the small pitch angle proton synchrotron model is to search for the appearance of neutrino signal from pp interactions (Paczynski & Xu 1994) at the moment of softening of the spectrum of prompt emission to $\alpha\le 1$ in GeV $\gamma$-ray-loud GRBs. The neutrino signal is expected to be sharply peaked at energies $E_\nu\sim f\Gamma_0m_{\rm p}\simeq 1\left[\Gamma_0/10^4\right]$ TeV and its flux is expected to be comparable to the luminosity of the cascade (GeV) component of the GRB spectrum. The peak energy of neutrino signal can be predicted if the bulk Lorentz factor $\Gamma_0$ is estimated from the measurement of the peak energy of the proton synchrotron component using Eq. (1). A search for the neutrino counterparts of the GeV $\gamma$-ray loud GRBs becomes possible after a cross-correlation of the signal of Fermi/LAT-detected GRBs with the TeV neutrino signal detected bu the km3 scale neutrino telescope IceCube, which will become possible in the nearest future (Abbasi et al. 2009).

6 Summary

We have explored the possibility that small pitch-angle proton synchrotron emission from the magnetized GRB outflow gives significant contribution to the GRB spectrum. This emission provides an important dissipation mechanism in the region of acceleration of GRB outflows with high magnetization parameter $\sigma$. We have shown that the steady-state spectrum of this emission is expected to have photon index $\alpha=-1$, close to the characteristic photon index of the time-resolved GRB spectra. A small pitch-angle proton synchrotron emission component could also explain the extremely hard spectra $\alpha\ga 0$ observed at the beginning of some GRBs. The possibility that small pitch angle proton synchrotron emission from the region of acceleration of GRB outflow could be identified in the observed GRB spectra implies that the models of formation of magnetized relativistic outflow by newly born stellar mass black holes or magnetars could be observationally tested. Whether a small pitch angle synchrotron emission component exists in the GRB spectra could be verified by searching for the cyclotron line features in spectra of (some of the) hardest GRBs and/or searching for prompt TeV neutrino emission from GeV $\gamma$-ray-loud GRBs.

Acknowledgements
We would like to thank A.Taylor for discussions of the subject. The work of AN is supported by the Swiss National Science Foundation grant PP00P2_123426.

References

  1. Abdo, A. A., Ackermann, M., Arimoto, M., et al. (Fermi collaboration) 2009a, Science, 323, 1688 [Google Scholar]
  2. Abdo, A. A., Ackermann, M., Ajello, M., et al. 2009b, ApJ, 706, L138 [NASA ADS] [CrossRef] [Google Scholar]
  3. Abbasi, R., Abdou, Y., Ackermann, M., et al. (IceCube collaboration) 2009, ApJ, 701, L47 [Google Scholar]
  4. Asano, K., Guiriec, S., & Meszaros, P. 2009, ApJ, 705, L191 [NASA ADS] [CrossRef] [Google Scholar]
  5. Baring, M. G., & Harding, A. K. 1997, ApJ, 491, L663 [NASA ADS] [CrossRef] [Google Scholar]
  6. Band, D., Matteson, J., Ford, L., et al. 1993, ApJ, 413, 281 [NASA ADS] [CrossRef] [Google Scholar]
  7. Band, D. L., Axelsson, M., Baldini, L., et al. 2009, ApJ, 701, 1673 [NASA ADS] [CrossRef] [Google Scholar]
  8. Bucciantini, N., Quataert, E., Arons, J., Metzger, B. D., & Thompson, T. A. 2007, MNRAS, 380, 1541 [NASA ADS] [CrossRef] [Google Scholar]
  9. Bucciantini, N., Quataert, E., Metzger, B. D., et al. 2009, MNRAS, 396, 2038 [NASA ADS] [CrossRef] [Google Scholar]
  10. Dermer, C. D., & Atoyan, A. 2006, N. J. Ph., 8, 122D [Google Scholar]
  11. Epstein, R. J. 1973, ApJ, 183, 593 [NASA ADS] [CrossRef] [Google Scholar]
  12. Ghisellini, G., & Celotti, A. 1999, ApJ, 511, L93 [NASA ADS] [CrossRef] [Google Scholar]
  13. Ghisellini, G., Ghirlanda, G., Nava, L., & Celotti., A. 2010, MNRAS, 403, 926 [NASA ADS] [CrossRef] [Google Scholar]
  14. Ghirlanda, G., Ghisellini, G., & Nava, L. 2010, A&A, 510, 7 [Google Scholar]
  15. Ginzburg, V. L., & Syrovatskii, S. I. 1969, ARA&A, 7, 375 [NASA ADS] [CrossRef] [Google Scholar]
  16. Kaneko, Y., Preece, R. D., Briggs, M. S., et al. 2006, ApJS, 166, 298 [NASA ADS] [CrossRef] [Google Scholar]
  17. Komissarov, S. S., & Barkov, M. V. 2007, MNRAS, 320, 1029 [NASA ADS] [CrossRef] [Google Scholar]
  18. Komissarov, S. S., Barkov, M. V., Vlahakis, N., & Königl, A. 2007, MNRAS, 380, 51 [NASA ADS] [CrossRef] [Google Scholar]
  19. Komissarov, S. S., Vlahakis, N., Königl, A., & Barkov, M. V. 2010, MNRAS, 394, 1182 [Google Scholar]
  20. Kumar, P., & Duran, R. B. 2009, MNRAS, 400, 75 [Google Scholar]
  21. Kirk, J. G., & Reville, B. 2010, ApJ, 710, L16 [NASA ADS] [CrossRef] [Google Scholar]
  22. Lloyd, N. M., & Petrosian, V. 2000, ApJ, 543, 722 [NASA ADS] [CrossRef] [Google Scholar]
  23. Medvedev, M. 2006, ApJ, 637, 869 [NASA ADS] [CrossRef] [Google Scholar]
  24. Medvedev, M., & Loeb, S. 1999, ApJ, 526, 697 [NASA ADS] [CrossRef] [Google Scholar]
  25. Mészáros, P. 2006, Rep. Prog. Phys., 69, 2259 [NASA ADS] [CrossRef] [Google Scholar]
  26. Paczynski, B., & Xu, G. 1994, ApJ, 427, 708 [NASA ADS] [CrossRef] [Google Scholar]
  27. Piran, T. 2004, Rev. Mod. Phys., 76, 1143 [Google Scholar]
  28. Preece, R. D., et al. 2000, ApJ, 506, L23 [Google Scholar]
  29. Razzaque, S., Dermer, C. D., & Finke, J. D. 2009 [arXiv:0908.0513] [Google Scholar]
  30. Savchenko, V., & Neronov, A. 2009, MNRAS, 396, 935 [NASA ADS] [CrossRef] [Google Scholar]

Footnotes

...$U=L/(\Omega D^2)$[*]
We use natural system of units c=1.
... field[*]
In a special case 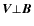$\vec V\bot\vec B$ the reference frame moving with velocity 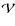${\cal V}$ is comoving with the outflow and the radiation is cyclotron radiation.

All Figures

  \begin{figure} \par\includegraphics[width=8.8cm,clip]{15212fg1} \end{figure} Figure 1:

Spectra of small pitch-angle proton synchrotron emission from pre-shock GRB outflow for several values of $\kappa $. Thick solid line shows an emission spectrum from a proton spectrum formed by cooling of the outflow protons to $\Gamma =0.01\Gamma _0$. For comparison, we show Band model fits to the spectra of periods ``b'' (beginning) and ``d+e'' (end) of Fermi GRB 090902B (Abdo et al. 2009b) as lower and upper grew curves. Normalizations and peak energies of the Band model fits to GRB 090902B spectra are shifted to match the small pitch-angle synchrotron model curves.

Open with DEXTER
In the text
Copyright ESO 2010

Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.

Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.

Initial download of the metrics may take a while.