| Issue |
A&A
Volume 553, May 2013
|
|
|---|---|---|
| Article Number | A66 | |
| Number of page(s) | 14 | |
| Section | Stellar atmospheres | |
| DOI | https://doi.org/10.1051/0004-6361/201220518 | |
| Published online | 07 May 2013 | |
Online material
Appendix A: Influence of the adopted model parameters on the spot maps
Appendix A.1: Time resolution (Δtf) and amount of regularization (β)
In Sect. 3.3 we discussed the method applied to determining the maximum time interval Δtf that our model can accurately fit when considering a fixed distribution of three active regions (Lanza et al. 2003). This corresponds to the time resolution of our model, since we assume that active regions do not evolve on timescales shorter than Δtf. The total interval T was divided to Nf segments so that Δtf = T/Nf. The optimal Nf was found to be 58 from the best fit of the three-spot model, as measured from the χ2 statistics.
Three cases corresponding to different lengths of the time interval Δtf are presented in Fig. A.1, where the ratio 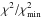 is plotted vs. Q, the facular-to-spotted area ratio; χ2 is the chi square of the composite best fit to the total time series; and
is plotted vs. Q, the facular-to-spotted area ratio; χ2 is the chi square of the composite best fit to the total time series; and 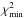 is the minimum χ2 obtained for Nf = 58 and Q = 8.0. The corresponding spot model is discussed in the text. Here we explore the case with Nf = 50 and perform an ME analysis with the corresponding time interval, i.e., Δtf = 10.1719 days, to investigate the effect of a different Δtf on our results. The same approach as described in Sect. 4.1 was considered, and regularized ME models were iteratively adjusted until β = 2. The isocontour map of the distribution of the spot filling factor vs. time and longitude is presented in Fig. A.2. It is remarkably similar to the one presented in Fig. 8 and the phased activity enhancement around ~350° is still visible, although the migration of the starspots and their changes on short timescales are less evident. Therefore, a longer time interval limits the possibility of accurately measuring spots’ migration and estimate their lifetimes.
is the minimum χ2 obtained for Nf = 58 and Q = 8.0. The corresponding spot model is discussed in the text. Here we explore the case with Nf = 50 and perform an ME analysis with the corresponding time interval, i.e., Δtf = 10.1719 days, to investigate the effect of a different Δtf on our results. The same approach as described in Sect. 4.1 was considered, and regularized ME models were iteratively adjusted until β = 2. The isocontour map of the distribution of the spot filling factor vs. time and longitude is presented in Fig. A.2. It is remarkably similar to the one presented in Fig. 8 and the phased activity enhancement around ~350° is still visible, although the migration of the starspots and their changes on short timescales are less evident. Therefore, a longer time interval limits the possibility of accurately measuring spots’ migration and estimate their lifetimes.
In a second test, we chose the same time interval as in Sect. 4, i.e., Nf = 58, to explore the effects of a different amount of regularization on the ME spot maps. In Fig. A.3, we present a spot map obtained with a lower regularization (β = 1). At that point, the large starspots appearing in Fig. 8 are resolved into several smaller spots. This fine structure changes vs. the time without any clear regularity, as expected for an artefact due to overfitting the noise present in the data.
Finally, we plot the distribution of the filling factor obtained with β = 3 in Fig. A.4. The effects of the overegularization described in Sect. 4.1 can be seen as a tendency for the appearance of larger and smoother spot groups that include previously resolved starspots. Three main groups separated by approximately 120° in longitude generally appear, showing the same backwards migration as with β = 2, the optimal value adopted for the regularization. The residuals of the composite best fit to the light curve (not shown) show larger systematic deviations than in the cases with β = 1 or β = 2, again a sign of overegularization.
 |
Fig. A.1
Ratio of the χ2 of the three-spot model fit to the minimum value for Nf = 58 ( |
| Open with DEXTER | |
 |
Fig. A.2
As in Fig. 8, but adopting a longer time interval Δtf = 10.1719 days. |
| Open with DEXTER | |
 |
Fig. A.3
As in Fig. 8, but performing an ME regularization with β = 1. |
| Open with DEXTER | |
 |
Fig. A.4
As in Fig. 8, but performing an ME regularization with β = 3. |
| Open with DEXTER | |
Appendix A.2: Inclination of the stellar spin axis
The inclination of the stellar spin is assumed to be equal to that of the orbit of the BD companion in our analysis, i.e. . An isotropic orientation of the stellar spin axis has its mode at i = 90° (Herrero et al. 2012), so it is reasonable to choose a value close to this, given that there is no a priori information to constrain the parameter.
The tidal timescale for the alignment of the stellar spin and the orbital angular momentum are comparable, in the case of LHS 6343 A, to the synchronization timescale that is estimated to be ≈7.5 Gyr. Given a probable age of the system between 1 and 5 Gyr, we cannot be sure that the obliquity has been damped by tides, unless we assume that most of the damping occurred during the pre-main-sequence phase when the tidal interaction was stronger due to a larger RA (cf. Sect. 5). Therefore, we adopt a prudential approach and explore different cases in which the system is out of spin-orbit alignment.
We adopt the same parameters as in Sect. 4.1 and compute a regularized ME best fit of the light curve with β = 2, fixing the stellar inclination to i = 60° and i = 45°. The resulting spot maps are plotted in Figs. A.5 and A.6, respectively. We may expect that the latitudinal distribution of the spots are remarkably different, especially for i = 45°. On the other hand, the plotted distributions of the filling factor vs. longitude and time are similar to what is computed with . Also the evolution and migration of the active regions are similar to that case. Only some details appear to be critically dependent on the adopted inclination.
The results of the tests described in this Appendix confirms that by averaging the ME spot maps over latitude, we can effectively remove most of the degeneracy present in the light curve inversion process.
 |
Fig. A.5
As in Fig. 8, but adopting a stellar inclination of i = 60°. |
| Open with DEXTER | |
 |
Fig. A.6
As in Fig. 8, but adopting a stellar inclination of i = 45°. |
| Open with DEXTER | |
© ESO, 2013
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


