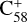EDP Sciences logo
Subscriber Authentication Point
Search
Menu
Home All issues Volume 662 (June 2022) A&A, 662 (2022) A21 Full HTML
Open Access
Issue
A&A
Volume 662, June 2022
Article Number A21
Number of page(s) 8
Section Atomic, molecular, and nuclear data
DOI https://doi.org/10.1051/0004-6361/202243087
Published online 06 June 2022

© C. Zhang et al. 2022

Licence Creative CommonsOpen Access article, published by EDP Sciences, under the terms of the Creative Commons Attribution License (https://creativecommons.org/licenses/by/4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

This article is published in open access under the Subscribe-to-Open model. Subscribe to A&A to support open access publication.

1 Introduction

The buckminsterfullerene (C60) molecule was first ‘discovered’ in the laboratory in 1985 (Kroto et al. 1985). In 2010, the existence of buckminsterfullerene (C60) in space was discovered through the infrared (IR) spectra of circumstellar and interstellar sources (Cami et al. 2010; Sellgren et al. 2010). Importantly, for the first time, several far-red diffuse interstellar bands were linked to the electronic transitions of buckminster-fullerene monocations (C60+) (Campbell et al. 2015; Walker et al. 2015; Cordiner et al. 2017). Hence, understanding the processes that regulate the origin and evolution of these fullerene species and their relationships with the organic inventory in space has become a focus of astrochemistry research (Tielens 2013, and references therein).

Fullerenes are exceptionally stable carbon cages that are difficult to destroy or modify (Handschuh et al. 1995; Candian et al. 2019). However, minor modifications that preserve the cage size and composition are much easier to accomplish, such as ionization, isomerization, and exohedral atom addition, as well as other chemical reactions, including association with PAH molecules (Jäger et al. 2009, 2011; 2019b). Therefore, when the total abundance of a fullerene species, such as C60, in an interstellar region is considered, its different ionization or chemical states should be considered instead of its abundance in a given state in space (Omont 2016; Cordiner et al. 2019, and references therein).

As C60 is an important component of the interstellar organic inventory, various studies have been performed on the formation mechanism of C60, and the formation of fullerenes has been shown to be chemically linked to large PAHs in the interstellar medium (ISM; Berné & Tielens 2012; Zhen et al. 2014; Andrews et al. 2015). Given the presence of C60 and PAHs in photodissociation regions (PDRs), such as NGC 7023 (Sellgren et al. 2010; Berné & Tielens 2012), studies on the chemical evolution network of C60 and PAH species have piqued the interest of researchers (for example, see Bohme 2016; Candian et al. 2019; Zhen et al. 2019a,b).

The adduction reactions of large molecular clusters and the charge transfer reactions of fullerene and PAHs have been extensively studied by Bohme and colleagues using the selected ion flow tube technique. The reactions of singly, doubly, and triply charged C60 with a wide range of collision partners have been studied (Bohme 1992, 2016; Petrie & Bohme 1993). The adduction reactions compete with the charge transfer channels, and doubly and triply charged fullerene (C60) cations exhibit considerably different behaviours relative to singly charged fullerene (C60) cations. The main reason for this is that charge transfer is endothermic for reactions with singly charged cations and exothermic for reactions with doubly and triply charged cations. In our previous studies, we investigated the formation and photo-dissociation of fullerene/anthracene, fullerene/9-vinylanthracene, and fullerene/9-methylanthracene cluster monocations (Zhen et al. 2019a,b), and molecular adduct formation was found to be the dominant channel.

A critical issue is understanding the influence of the collision reactions of fullerenes with PAH species or other coexisting molecules in the ISM (Omont 2016). Recently, for the first time, two nitrile-functionalized PAHs, 1- and 2-cyanonaphthalene, were detected in the ISM. The bicyclic ring PAH molecules with functional groups were observed in the Taurus molecular cloud 1 (TMC-1; McGuire et al. 2021).

In this work, to understand how monocationic fullerenes aggregate with functional PAH species (specifically, with -CH2 functional groups) in the gas phase and to illustrate the competition between charge transfer and molecular cluster formation channels, as well as the importance of charge exchanges, an experimental and theoretical study on ion–molecule collision reactions between monocationic fullerene (e.g. C60/70, C56/58 and C66/68) and neutral 2,3-benzofluorene is presented. We selected 2,3-benzofluorene (C17H12, 28 atoms, m/z = 216) as the large neutral molecule due to the unique behaviour of its gas-phase reactions with fullerene cations.

2 Experimental results

The experiments were performed on an apparatus equipped with a quadrupole ion trap and reflection time-of-flight mass spectrometer, and the experimental details are provided in the Appendix A (Zhen et al. 2019a,b). During the experiments, the high energy of the impacting electrons (~82 eV) causes fullerene (C60 and C70) monocations to form, and the fragmentation of the original fullerene cations through C2 losses leads to the formation of smaller fullerenes (C54/56/58 or C64/66/68) monocations (Lifshitz 2000; Zhen et al. 2014). The resulting mass spectra are presented in Figs. 1 and 2.

The mass spectra of the fullerene (C54/56/58 and C60) monocation collision reactions with (red line) and without (blue line) neutral 2,3-benzofluorene are shown in Fig. 1A. Clearly, during the reaction with 2,3-benzofluorene, a strong mass peak associated with the 2,3-benzofluorene cations 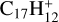(m/z = 216) is observed, as well as a series of weak mass peaks associated with the fullerene/2,3-benzofluorene cluster cations. In Fig. 1B, we label the newly formed fullerene (C54, C56, C58, and C60)/2,3-benzofluorene cluster cations as [(C17H12)C54]+ (m/z = 864), [(C17H12)C56]+ (m/z = 888), [(C17H12)C58]+ (m/z = 912), and [(C17H12)C60]+ (m/z = 936), respectively.

Importantly, the intensity of the species I(, without 2,3-benzofluorene) ≃ I(, with 2,3-benzofluorene) + I([(C17H12)C58]+), and I(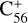, without 2,3-benzofluorene) ≃ I(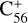, with 2,3-benzofluorene) + I([(C17H12)C56]+). This result suggests that for fullerene (C56/58) monocations, the molecular cluster formation channel is the dominant channel. Furthermore, the intensity of the species I(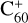, without 2,3-benzofluorene) ≃ I(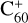, with 2,3-benzofluorene) + , and the intensity ratio of , which suggests that, for fullerene (C60) monocations, the charge transfer channel is the dominant channel. The intensity of the newly formed 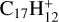is similar to the intensity of I(, without 2,3-benzofluorene) − I(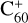, with 2,3-benzofluorene) as a result of the ion-molecular charge transfer channel. We discuss this result later in combination with the theoretical results.

Based on the above results, for the gas-phase reaction of fullerene (C54/56/58 and C60) monocations with neutral 2,3-benzofluorene, the reaction pathways are as follows. The fullerene (C60) monocation reaction with neutral 2,3-benzofluorene is

The fullerene (C54/56/58) monocation reaction with neutral 2,3-benzofluorene is

Similar to the reaction behaviour of fullerene (C60 and C54/56/58) monocation and 2,3-benzofluorene systems, the mass spectra of the fullerene (C64/66/68 and C70) monocation collision reaction with (red line) and without (blue line) neutral 2,3-benzofluorene are shown in Fig. 2A. Clearly, when 2,3-benzofluorene is present, a strong mass peak associated with the 2,3-benzofluorene cations 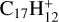(m/z = 216) is observed, as well as a series of weak mass peaks associated with the fullerene/2,3-benzofluorene cluster cations. As shown in Fig. 2B, we label the newly formed fullerene (C64, C66, C68, and C70)/2,3-benzofluorene cluster cations as [(C17H12)C64]+ (m/z = 984), [(C17H12)C66]+ (m/z = 1008), [(C17H12)C64 (m/z = 1032), and [(C17H12)C70]+ (m/z = 1056), respectively.

Importantly, the intensity of the species I(, without 2,3-benzofluorene) ≃ I(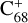, with 2,3-benzofluorene) + I([(C17H12)C68]+) and I(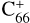, without 2,3-benzofluorene) ≃ I(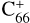, with 2,3-benzofluorene) + I([(C17H12)C56]+). This result suggests that for fullerene (C66/68) monocations, the molecular cluster formation channel is the dominant channel. Furthermore, the intensity of the species I(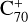, without 2,3-benzofluorene) ≃ I(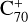, with 2,3-benzofluorene) + , and the intensity ratio of , which suggests that, for fullerene (C70) monocations, the charge transfer channel is the dominant channel. The intensity of the newly formed 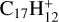is similar to the intensity of I(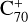, without 2,3-benzofluorene) − I(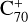, with 2,3-benzofluorene) due to the ion-molecular charge transfer channel. We discuss this result later in combination with the theoretical results.

Based on the above results, for the gas-phase reaction of fullerene (C64/66/68 and C70) monocations with neutral 2,3-benzofluorene, we propose the following reaction pathways. The fullerene (C70) monocation reaction with neutral 2,3-benzofluorene is

The fullerene (C64/66/68) monocation reaction with neutral 2,3-benzofluorene is

In addition, in Figs. 1 and 2, we observe additional mass features when 2,3-benzofluorene is not present. We suspect that these mass peaks, which occur at approximately m/z = 200 in Figs. 1 and 2, form either due to previous similar experiments or as a side product due to contamination in the vacuum chamber. The mass peaks at approximately m/z = 360 in Fig. 1 are the dication species of C58/60; the mass peaks at approximately m/z = 420 in Fig. 2 are the dication species of C68/70; and the mass peaks at approximately m/z = 600 in Fig. 2 are the cation species of previous experiments (dicoronylene cations, 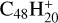). We do not believe that these species affect the interpretation of our measurements due to their relatively small magnitudes.

thumbnail Fig. 1

Mass spectrum of the products resulting from the fullerene (C54/56/58 and C60) monocation collision reaction with and without neutral 2,3-benzofluorene. Panel A: mass spectrum of fullerene (C54/56/58 and C60) monocations with and without 2,3-benzofluorene. Panel B: presence of [C54/56/58/60]+ and the newly formed [(C17H12)C54/56/58/60]+ cluster cations.
thumbnail Fig. 2

Mass spectrum of the products resulting from the fullerene (C64/66/68 and C70) monocation collision reaction with and without neutral 2,3-benzofluorene. Panel A: mass spectrum of fullerene (C64/66/68 and C70) monocations with and without 2,3-benzofluorene. Panel B: presence of [C64/66/68/70]+ and the newly formed [(C17H12)C64/66/68/70]+ cluster cations.

3 Theoretical calculation results

To understand the details of the reaction process of the fullerene monocations with neutral 2,3-benzofluorene, we use 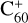+ 2,3-benzofluorene and (7 C-ring or 6 C-ring) + 2,3-benzofluorene as examples and study their theoretical adduct reactions and charge transfer processes. We follow the minimum energy pathway. At each step, from the van der Waals cluster to the covalently bonded clusters, the energy and optimized structures are calculated. The details of the theoretical calculation are provided in the Appendix A.

The energies and optimized structures for the reactant, transition states (TS, or TS1 and TS2), intermediate states, and product of the adduct reaction pathway between 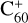and 2,3-benzofluorene, 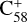(7 C-ring/6 C-ring) and 2,3-benzofluorene are shown in Figs. 3–5, as well as the charge transfer channels. At the beginning of the calculations, we consider the reaction process between the fullerene cations and the 2,3-benzofluorene cluster cations in two types of channels. The I channel (the blue line) is the charge transfer channel, where charge exchange occurs during the collision reaction between the fullerene cations and 2,3-benzofluorene. The II channel (the red line) is the adduct formation channel, where clusters (van der Waals clusters or covalently bonded clusters) form during the collision reaction between the fullerene cations and 2,3-benzofluorene. These two channels compete throughout the reaction process, experiencing relatively similar intermediate processes or sharing the same initial precursors (fullerene cation + 2,3-benzofluorene, which is defined as 0eV).

3.1 The optimized geometric structure of the fullerene (C58) monocations and neutral 2,3-benzofluorene

We assume that the fullerene (C58) monocations do not have a carbon skeleton rearrangement (except for the C2 loss at a local position) during the electron impact ionization and fragmentation process. The 7 C-ring isomer structure is selected for the fullerene (C58) cations, and we focus on this isomer in the following calculations (Lee & Han 2004; Candian et al. 2019; Zhen et al. 2019b). has an odd number of electrons, resulting in an open-shell doublet ground state with a spin multiplicity of 2 in the electronic ground state.

Neutral 2,3-benzofluorene is a relatively complex and asymmetrical molecule. Unlike the anthracene (C14H10) reaction with fullerene cations (Zhen et al. 2019b), due to the asymmetric structure of 2,3-benzofluorene, two adduct pathways need to be considered in accordance with the CH2 unit binding effect: a-C17H12 (the CH unit without the effect of the CH2 unit, where the CH2 and CH units are not adjacent) and b-C17H12 (the CH unit with the effect of the CH2 unit, where the CH2, and CH units are adjacent).

3.2 Reaction process of 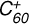with C17H12

As shown in Fig. 3, at the beginning of the adduction process (red line), 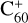and 2,3-benzofluorene form a van der Waals molecular complex ([C60-(C17H12)]+) with an exothermic energy of approximately 1.00 eV. For the covalently bonded product, in the 2,3-benzofluorene ‘landing’ during the 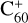process, two carbon atoms from C60 are removed from the cage surface, and the 2,3-benzofluorene structure is modified to allow the 9, 10 C-atoms to bond to the fullerene C-atoms (Sato et al. 2013; Zhen et al. 2019b). Due to the asymmetric structure of 2,3-benzofluorene, two transition states are obtained based on the CH2 unit, as presented in Figs. 3A and B.

As shown in Fig. 3A, the CH unit first bonds to the fullerene cage, then enters a transition state ([C60-(a-C17H12)]+, TS, −0.06 eV) and passes the first activation barrier (0.93 eV). Then, the final product (product, [C60-(C17H12)]+, −0.25 eV) is formed. Considering the energy loss of the van der Waals molecules, including IR emissions and collisions, a small number of covalently bonded molecules may form during the transition state ([C60-(a-C17H12)]+, TS, −0.06eV).

As presented in Fig. 3B, the CH unit (the same side as the CH2 unit) first bonds to the fullerene cage, then enters a transition state ([C60-(b-C17H12)]+, TS, +0.06eV) and passes the first activation barrier (1.05 eV). Then, the final product (product, [C60-(C17H12)]+, −0.25 eV) is formed. However, considering the energy loss of the van der Waals molecules, such as IR emissions and collisions, no covalently bonded molecules form during the transition state ([C60-(b-C17H12)]+, TS, +0.06eV).

During the adduct reaction process, charge transfer (blue line) easily occurs between the C60 monocation and neutral 2,3-benzofluorene. As presented in Fig. 3, the charge exchange reaction of 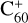with 2,3-benzofluorene is exothermic with an energy of 0.27 eV, which is thermodynamically favourable.

thumbnail Fig. 3

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.

3.3 Reaction of 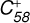(6 C-ring) with C17H12

For the reaction of C58 monocations with neutral 2,3 benzofluorene, due to the structure of 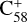, two typical reaction pathways are considered (Zhen et al. 2019b). The first pathway is in the ‘6 C-ring’, and the other pathway is in the ‘7 C-ring’. The calculation results are presented in Figs. 4 and 5, respectively.

The theoretical results of the reaction pathway between 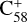(6 C-ring) and 2,3-benzofluorene are presented in Fig. 4. At the beginning of the adduction process (red line), 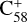(6 C-ring) and 2,3-benzofluorene form a van der Waals molecular complex ([C58 (6 C-ring)-(C17H12)]+) with an exothermic energy of approximately 0.78 eV. For the covalently bonded product, in the 2,3-benzofluorene Ίanding’in the 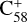process, two carbon atoms from C58 are removed from the cage surface, and the 2,3-benzofluorene structure is modified to allow the 9, 10 C-atoms to bond to the C-atoms from (6 C-ring) (Sato et al. 2013; Zhen et al. 2019b). Due to the asymmetric structure of 2,3-benzofluorene, two transition states are obtained based on the CH2 unit of 2,3-benzofluorene, as presented in Figs. 4A and B.

Figure 4A shows that the CH unit first bonds to the fullerene cage. Then, the CH unit goes through a transition state ([C58-(a-C17H12)]+, TS, +0.22 eV) and passes the first activation barrier (1.00 eV). Next, the final product (product, [C58-(C17H12)]+, −0.01 eV) is formed. As presented in Fig. 4B, the CH unit (the same side as the CH2 unit) first bonds to the fullerene cage, then enters a transition state ([C58-(b-C17H12)]+, TS, +0.33 eV) and passes the first activation barrier (1.11 eV). After that, the final product (product, [C58-(C17H12)]+, −0.01 eV) is formed. However, considering the energy loss of van der Waals molecules, such as IR emissions and collisions, no covalently bonded molecules form during the transition (TS, [C58-(a-C17H12)]+ and TS, [C58-(b-C17H12)]+).

During the adduct reaction process, charge transfer (blue line) cannot occur between the C58 cation and 2,3-benzofluorene. As presented in Fig. 4, the charge exchange reaction of 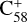with 2,3-benzofluorene is endothermic with an energy of 0.32 eV, which is thermodynamically unfavourable.

3.4 Reaction of 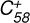(7 C-ring) with C17H12

The theoretical results of the reaction pathway between 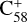(7 C-ring) and 2,3-benzofluorene are presented in Fig. 5. As shown in Fig. 5, initially (red line) 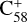(7 C-ring) and 2,3-benzofluorene form a van der Waals molecular complex ([C58 (7 C-ring)-(C17H12)]+, with an exothermic energy of approximately 0.93 eV). For the covalently bonded product, in the 2,3-benzofluorene ‘landing’ during the 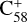process, two carbon atoms from C58 are removed from the cage surface, and the 2,3-benzofluorene structure is modified to allow the 9, 10 C-atoms to bond to the C-atoms from 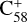(7 C-ring) (Sato et al. 2013; Zhen et al. 2019b). Due to the asymmetric structure of 2,3-benzofluorene, multiple transition pathways are obtained based on the effect of the CH2 unit of 2,3-benzofluorene, as presented in Figs. 5A and B.

As presented in Fig. 5A, the CH unit first bonds to the fullerene cage (7 C-ring) first; then, the CH unit enters an intermediary state (Inter, [C58 (7 C-ring)-(a-C17H12)]+, −1.02eV) before entering the first transition state (TS1, [C58 (7 C-ring)-(a-C17H12)]+, −0.91 eV) and passing the first activation barrier (0.02eV). After that, the product (product, [C58 (7 C-ring)-(C17H12)]+) is formed with an exothermic energy of approximately 0.31 eV in the second transition state (TS2, [C58 (7 C-ring)-(a-C17H12)]+, −0.28 eV), which passes the second activation barrier (0.74eV).

As presented in Fig. 5B, the CH unit (the same side as the CH2 unit) first bonds to the fullerene cage (7 C-ring); then, the CH unit enters an intermediary state (Inter, [C58 (7 C-ring)-(b-C17H12)]+, −0.66 eV) before entering the first transition state (TS1, [C58 (7 C-ring)-(b-C17H12)]+, −0.67 eV) and passing the first activation barrier (0.26 eV). After that, the product (product, [C58 (7 C-ring)-(C17H12)]+ is formed with an exothermic energy of approximately 0.31 eV in the second transition state (TS2, [C58 (7 C-ring)-(b-C17H12)]+, −0.21 eV), which passes the second activation barrier (0.46 eV). Considering the energy loss of the van der Waals molecules, such as IR emissions and collisions, a large number of covalently bonded molecules are formed during the transition states.

During the adduct reaction process, charge transfer (blue line) cannot occur between the C58 cation and 2,3-benzofluorene. As presented in Fig. 5, the charge exchange reaction of 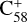with 2,3-benzofluorene is endothermic with an energy of 0.32 eV, which is thermodynamically unfavourable.

The charge transfer channel between the C56 cation and 2,3-benzofluorene was also calculated. The charge exchange reaction of 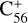with 2,3-benzofluorene is endothermic with an energy of 0.64 eV, which is thermodynamically unfavourable. We note that we simplified the charge transfer channel in the ion-molecular collision reaction process, and our conclusions were mainly based on the DFT calculation results. A molecular dynamic simulation that treats the fullerene cations as metallic spheres and 2,3-benzofluorene as thin circular conducting discs is needed to understand more details of the collision process (Zettergren et al. 2012; Forsberg et al. 2013).

thumbnail Fig. 4

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of (6 C-ring) with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.

4 Discussion

Overall, the obtained theoretical calculation results are consistent with the experimental results: the charge transfer process is the dominant channel for the reaction system of 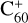and 2,3-benzofluorene, while the adduct formation channel has little influence. In contrast, the adduct formation channel is the dominant channel for the reaction system of 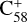(7 C-ring) and 2,3-benzofluorene. Thus, the charge transfer process is likely unfavourable for the system of 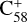and 2,3-benzofluorene.

In the theoretical calculations, we separate the reaction process between the fullerene cations and 2,3-benzofluorene into two types of channels (Zhen et al. 2019b). The first type of channel is the adduct formation channel, in which two processes occur: the first process is between the fullerene cation and 2,3-benzofluorene to form the van der Waals cluster (Initial), which is energetically unfavourable with the reactants; the second process is the transition from the van der Waals cluster (Initial) to the covalently bonded cluster (Product).

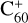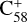There is a substantially higher energy barrier during the transition from the van der Waals cluster to the covalently bonded cluster for and (6 C-ring) than for 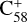(7 C-ring). Thus, if clusters form, fullerene ( 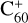and 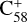(6 C-ring))/2,3-benzofluorene cluster cations are trapped in the van der Waals form and do not have sufficient energy to overcome the energy barrier of the covalently bonded species. In contrast, if they are trapped in van der Waals form, fullerene ( 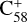(7 C-ring))/2,3-benzofluorene cluster cations can form, overcoming the energy barrier as covalently bonded species. The second type of channel is the charge transfer channel; charge transfer easily occurs between the C60 cation and 2,3-benzofluorene, which is thermodynamically favourable, while charge transfer cannot occur between the C58 cation and 2,3-benzofluorene, which is thermodynamically unfavourable.

According to the obtained theoretical results, we can infer that the formation of van der Waals species is energetically favourable and that all species have relatively strong van der Waals bonding abilities. However, according to the experimental results, almost no clusters based on the fullerene (C60) cations form, and we speculate that van der Waals bond cluster molecules do not play an important role in the adduct process. Molecular cluster formation driven by covalent bond forces makes up the overall ion-molecular reaction process. We further surmise that the van der Waals cluster does not survive in our experimental conditions, while the covalently bonded species do.

When reacted with fullerene (C60 and C70) cations, compared with some PAH molecules (e.g. anthracene, C14H10) (Zhen et al. 2019b), 2,3-benzofluorene has a weak covalent bond ability due to the effect of the CH2 unit. For smaller fullerene molecules, the bonding patterns with anthracene and 2,3-benzofluorene are similar; the cluster is formed mainly due to the higher bonding ability of the smaller fullerene cations.

thumbnail Fig. 5

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of (7 C-ring) with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.

5 Astronomical implications and conclusions

The total abundance of fullerene (C60 and C70) in interstellar environments, such as the PDRs, is found through several given states as opposed to one (Omont 2016). The abundance of each given state is affected by the surrounding physical and chemical environmental conditions (Berné & Tielens 2012; Omont 2016; Candian et al. 2019). According to previous works, for fullerene species, the cluster formation channels compete with the charge transfer channels. The cluster formation channels are the dominant channels for reactions with singly charged cations, since the charge transfer channel is endothermic for these reactions, while the charge transfer channel are the dominant channels for reactions with doubly and triply charged cations, since the charge transfer channel is exothermic for these reactions (Bohme 2016).

However, based on our current results, charge transfer channels are also the dominant reaction channels for singly charged fullerenes (C60/70) when they react with natural 2,3-benzofluorene. Based on this result, for interstellar chemical-evolution models for the molecular spatial evolution of fullerenes and PAH species in space, especially when the concentration ratio of the neutral fullerene molecules to the fullerene monocations is considered, differences in the reaction of fullerene monocations with their coexisting interstellar neutral molecules should be taken into account, such as anthracene (C14H10)-type molecules, which have higher covalent bond abilities that lead to the formation of clusters (Zhen et al. 2019b), or 2,3-benzofluorene (C17H12)-type molecules, which have weak covalent bond abilities that lead to charge exchanges.

(Bernard-Salas et al. 2012) was unable to satisfactorily explain the relative intensities of the IR emission bands attributed to C60 in planetary nebulae. They suggested that other substances, such as C70, contributed to some of the bands, resulting in the inconsistencies observed in their results. Based on our results, fullerenes (C60/70) increase the abundance of their monocation forms through collision reactions with other neutral molecules, which may better explain their evolution and charge distribution characteristics in the ISM. However, further observational and experimental studies are required to address these issues.

In addition, it has been suggested that PAH clusters play an important role in extended red emissions, which are prominent in various interstellar and circumstellar environments (Rhee et al. 2007; Montillaud et al. 2013). The fullerene/PAH adducts formed in our experiments may be relevant to these extended red emissions. Similarly, these types of clusters may play an important role in the IR spectral complexity of circumstellar environments, where C60 is prominent (Cami et al. 2010; Sloan et al. 2014; Otsuka et al. 2014). Furthermore, the covalent fullerene/PAH clusters obtained in this work may be an important step in the formation of larger carbon grains (Dunk et al. 2013). 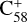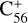Our present results indicate that smaller fullerene cations, such as and ( and 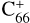), may form adducted clusters with PAH species even though these PAHs have weak covalent bond abilities (e.g. 2,3-benzofluorene, C17H12). Hence, if these smaller fullerenes are present in space, the formation of covalently bonded fullerene-based clusters could produce an extended family of large molecules (Candian et al. 2018).

In conclusion, the experimental and theoretical results of the gas-phase reaction of fullerene cations with 2,3-benzofluorene are presented, demonstrating the importance of charge transfer channels and illustrating the competition between charge transfer and molecular cluster formation channels. The ion-molecule collision reaction between fullerene cations and 2,3-benzofluorene offers a good model for understanding the physical-chemical processes of the charge transfer channel and cluster formation channels. Thus, fullerenes (C60/70) increase the abundance of their monocations forms through collision reactions with other neutral molecules in the ISM. Further observational studies are warranted, especially in consideration of the spatial evolution of fullerenes and PAH species in space.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (NSFC, Grant Nos. 41625013, 12073027, and 21827804), the Pre-research Project on Civil Aerospace Technologies (D020202) of the Chinese National Space Administration, and the Fundamental Research Funds for the Central Universities of China (WK3410000019). The theoretical calculations were performed at the Supercomputing Center of China’s University of Science and Technology.

Appendix A Experimental and theoretical calculation methods

The experiment was performed on our apparatus, which was equipped with a quadrupole mass filter, a quadrupole ion trap, and a time-of-flight mass spectrometer, which was described in detail in (Zhen et al. 2019a,b). Briefly, gas-phase fullerene (C60 and C70) was produced by heating fullerene powder to ~ 573 and 593 K and then ionizing the power with an electron gun. These fullerene ions were then transported into the ion trap for accumulation via an ion gate and the quadrupole mass filter. Due to the collision between the buffer gas helium and fullerene cations in the ion trap, we assume that the fullerene cations are all in their ground state and have the same temperature as He (room temperature, 298 K). The gas-phase 2,3-benzofluorene (C17H12) molecules were produced by heating its powder to ~ 323 K, then continuously effusing its product towards the centre of the trap for a chemical reaction with the fullerene cations. After some amount of time after the reaction, the ions were detected by reflection time-of-flight mass spectrometry. A highly precise digital generator was used to control the time sequences, including the ion gate, the beam shutter, and the measurement of the trapped ions. The experiments were run at 0.1 Hz, with a measurement period lasting 10 s. The following time sequence schemes were employed in the experiments: the ion gate remained open from 0-9.88 s, allowing fullerene cations to accumulate and react with 2,3-benzofluorene. Then, at 9.88 s, all trapped cation species were removed from the ion trap and detected by time-of-flight mass spectrometry.

The calculations were based on density functional theory (DFT) at the B3LYP level (Becke 1992). A basis set of 6-31+g(d, p), including the dispersion correction (D3), was chosen to describe the clusters with van der Waals bonds (Grimme et al. 2011; Sato et al. 2013; Frisch et al. 2016). The geometries of all species were optimized at the local minimum of their potential energy surface in the calculation. For the fullerene cations, 2,3-benzofluorene, and their adducts, frequency calculations were also performed based on their optimized geometries. Furthermore, the frequency calculation determined the zero-point energy and the thermal corrections to the molecular energy. We emphasize that the basis set-superposition error correction is not included in the calculation because of its slight influence on the binding energy.

References

  1. Andrews, H., Boersma, C., Werner, M. W., et al. 2015, ApJ, 807, 99 [NASA ADS] [CrossRef] [Google Scholar]
  2. Becke, A. D. 1992, J. Chem. Phys., 96, 2155 [NASA ADS] [CrossRef] [Google Scholar]
  3. Bernard-Salas, J., Cami, J., Peeters, E., et al. 2012, ApJ, 757, 41 [NASA ADS] [CrossRef] [Google Scholar]
  4. Berné, O., & Tielens, A. G. G. M. 2012, Proc. Natl. Acad. Sci. U.S.A., 109, 401 [CrossRef] [Google Scholar]
  5. Bohme, D. K. 1992, Chem. Rev., 92, 1487 [CrossRef] [Google Scholar]
  6. Bohme, D. K. 2016, Phil. Trans. A Math. Phys. Eng. Sci., 374, 20150321 [Google Scholar]
  7. Cami, J., Bernard-Salas, J., Peeters, E., & Malek, S. E. 2010, Science, 329, 1180 [NASA ADS] [CrossRef] [Google Scholar]
  8. Campbell, E. K., Holz, M., Gerlich, D., & Maier, J. P. 2015, Nature, 523, 322 [NASA ADS] [CrossRef] [Google Scholar]
  9. Candian, A., Zhen, J., & Tielens, A. G. G. M. 2018, Phys. Today, 71, 38 [NASA ADS] [CrossRef] [Google Scholar]
  10. Candian, A., Rachid, M. G., MacIsaac, H., et al. 2019, MNRAS, 485, 1137 [CrossRef] [Google Scholar]
  11. Cordiner, M. A., Cox, N. L. J., Lallement, R., et al. 2017, ApJ, 843, L2 [NASA ADS] [CrossRef] [Google Scholar]
  12. Cordiner, M. A., Linnartz, H., Cox, N. L. J., et al. 2019, ApJ, 875, L28 [NASA ADS] [CrossRef] [Google Scholar]
  13. Dunk, P. W., Adjizian, J. J., Kaiser, N. K., et al. 2013, Proc. Natl. Acad. Sci. USA, 110, 18081 [NASA ADS] [CrossRef] [Google Scholar]
  14. Forsberg, B. O., Alexander, J. D., Chen, T., et al. 2013, J. Chem. Phys., 138, 054306 [NASA ADS] [CrossRef] [Google Scholar]
  15. Frisch, M. J., Trucks, G. W., Schlegel, H. B., et al. 2016, Gaussian 16, Revision E. 01 (Wallingford, CT: Gaussian, Inc.) [Google Scholar]
  16. Grimme, S., Ehrlich, S., & Goerigk, L. 2011, J. Comp. Chem., 32, 1456 [CrossRef] [Google Scholar]
  17. Handschuh, H., Gantefor, G., Kessler, B., Bechthold, P. S., & Eberhardt, W. 1995, Phys. Rev. Lett., 74, 1095 [NASA ADS] [CrossRef] [Google Scholar]
  18. Jäger, C., Huisken, F., Mutschke, H., Llamas Jansa, I., & Henning, T., 2009, ApJ, 696, 706 [CrossRef] [Google Scholar]
  19. Jäger, C., Mutschke, H., Henning, T., & Huisken, F. 2011, in EAS Pub. Ser., 46, eds. C. Joblin, & A.G.G.M. Tielens, 293 [CrossRef] [EDP Sciences] [Google Scholar]
  20. Kroto, H. W., Heath, J. R., Obrien, S. C., Curl, R. F., & Smalley, R. E. 1985, Nature, 318, 162 [NASA ADS] [CrossRef] [Google Scholar]
  21. Lee, S. U., & Han, Y. 2004, J. Chem. Phys., 121, 3941 [NASA ADS] [CrossRef] [Google Scholar]
  22. Lee, C., Yang, W., & Parr, R. G. 1988, Phys. Rev. B, 37, 785 [Google Scholar]
  23. Lifshitz, C. 2000, Int. J. Mass Spectrom., 200, 423 [NASA ADS] [CrossRef] [Google Scholar]
  24. McGuire, B. A., Loomis, R. A., Burkhardt, A. M., et al. 2021, Science, 371, 1265 [Google Scholar]
  25. Montillaud, J., Joblin, C., & Toublanc, D. 2013, A&A, 552, A15 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  26. Omont, A. 2016, A&A, 590, A52 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
  27. Otsuka, M., Kemper, F., Cami, J., Peeters, E., & Bernard-Salas, J. 2014, MNRAS, 437, 2577 [NASA ADS] [CrossRef] [Google Scholar]
  28. Petrie, S., & Bohme, D. K. 1993, Nature, 365, 426 [NASA ADS] [CrossRef] [Google Scholar]
  29. Rhee, Y. M., Lee, T. J., Gudipati, M. S., Allamandola, L. J., & Head-Gordon, M. 2007, Proc. Natl. Acad. Sci. USA, 104, 5274 [NASA ADS] [CrossRef] [Google Scholar]
  30. Sato, S., Maeda, Y., Guo, J., et al. 2013, J. Am. Chem. Soc., 135, 5582 [CrossRef] [Google Scholar]
  31. Sellgren, K., Wener, M. W., Ingalls, J. G., et al. 2010, ApJ, 722, 54 [Google Scholar]
  32. Sloan, G. C., Lagadec, E., Zijlstra, A. A., et al. 2014, ApJ, 791, 28 [NASA ADS] [CrossRef] [Google Scholar]
  33. Tielens, A. G. G. M. 2013, Rev. Mod. Phys., 85, 1021 [NASA ADS] [CrossRef] [Google Scholar]
  34. Walker, G. A. H., Bohlender, D. A., Maier, J. P., & Campbell, E. K. 2015, ApJ, 812, L8 [NASA ADS] [CrossRef] [Google Scholar]
  35. Zettergren, H., Forsberg, B. O., & Cederquist, H. 2012, Phys. Chem. Chem. Phys., 14, 16360 [NASA ADS] [CrossRef] [Google Scholar]
  36. Zhen, J., Castellanos, P., Paardekooper, D. M., Linnartz, H., & Tielens, Agg M. 2014, ApJ, 797, L30 [CrossRef] [Google Scholar]
  37. Zhen, J., Zhang, W., Yang, Y., & Zhu, Q. 2019a, MNRAS, 486, 3259 [NASA ADS] [CrossRef] [Google Scholar]
  38. Zhen, J., Zhang, W., Yang, Y., Zhu, Q., & Tielens, A. G. G. M. 2019b, ApJ, 887, 70 [NASA ADS] [CrossRef] [Google Scholar]

All Figures

thumbnail Fig. 1

Mass spectrum of the products resulting from the fullerene (C54/56/58 and C60) monocation collision reaction with and without neutral 2,3-benzofluorene. Panel A: mass spectrum of fullerene (C54/56/58 and C60) monocations with and without 2,3-benzofluorene. Panel B: presence of [C54/56/58/60]+ and the newly formed [(C17H12)C54/56/58/60]+ cluster cations.
In the text
thumbnail Fig. 2

Mass spectrum of the products resulting from the fullerene (C64/66/68 and C70) monocation collision reaction with and without neutral 2,3-benzofluorene. Panel A: mass spectrum of fullerene (C64/66/68 and C70) monocations with and without 2,3-benzofluorene. Panel B: presence of [C64/66/68/70]+ and the newly formed [(C17H12)C64/66/68/70]+ cluster cations.
In the text
thumbnail Fig. 3

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.
In the text
thumbnail Fig. 4

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of (6 C-ring) with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.
In the text
thumbnail Fig. 5

Reactants, transition states, intermediate states, products, and corresponding energies of the reaction pathways of (7 C-ring) with 2,3-benzofluorene: the I channel (the blue line) is the charge transfer channel, and the II channel (the red line) is the adduct formation channel. These two channels originate from the same starting point, which is defined as 0 eV.
In the text

Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.

Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.

Initial download of the metrics may take a while.