| Issue |
A&A
Volume 543, July 2012
|
|
|---|---|---|
| Article Number | A17 | |
| Number of page(s) | 4 | |
| Section | Planets and planetary systems | |
| DOI | https://doi.org/10.1051/0004-6361/201117346 | |
| Published online | 21 June 2012 | |
Research Note
The role of Mab as a source for the μ ring of Uranus
UNESP – Univ Estadual Paulista, Campus de Guaratinguetá, Brazil
e-mail: rsfair@feg.unesp.br
Received: 25 May 2011
Accepted: 24 May 2012
Context. We previously analysed how the solar radiation force combined with the planetary oblateness changes the orbital evolution of a sample of dust particles located at the secondary ring system of Uranus. Both effects combined with the gravitational perturbations of the close satellites lead to the depletion of these dust particles through collisions on the surfaces of these satellites on a timescale of hundreds of years.
Aims. In this work we investigate if the impacts of interplanetary dust particles (IDPs) onto Mab’s surface can produce sufficient particles to replenish the μ ring population.
Methods. We first analysed through numerical simulations the evolution of a sample of particles ejected from the surface of Mab and computed the lifetime of the grains when the effects of the solar radiation pressure and the planetary oblateness are taken into account. Then we estimated the mass production rate due to the impacts of IDPs following a previously established algorithm, and used this value to determine the time necessary to accumulate an amount of particles comparable with the mass of the μ ring.
Results. Based on an estimate of the flux of interplanetary particles and on the surface properties of Mab it is expected that the satellite supplies material to the ring at a rate of ~3 g/s. Meanwhile, our numerical model showed that the ejected particles are removed from the system through collisions with the satellite, and the mean lifetime of the grains may vary from 320 to 1500 years, depending on the radius of the particle.
Conclusions. The time necessary to accumulate the mass of the μ ring via ejection from Mab is much shorter than the mean lifetime of the particles, and a stationary regime is not reached. If the ring is kept in a steady state, other effects such as the electromagnetic force and/or the existence of additional bodies may play a significant role in the dust balance, but the current lack of information about the environment renders modelling these effects unfeasible.
Key words: planets and satellites: rings / methods: numerical / planets and satellites: individual: Uranus
© ESO, 2012
1. Introduction
Our knowledge of the outer limit of the Uranus ring system was extended with the discovery of the tenuous μ ring (Showalter & Lissauer (2006) – SL06 hereafter). The ring presents a clear triangular profile and extends 17 000 km radially from the orbit of the satellite Puck, encompassing the orbit of the satellite Mab, which matches the peak of the ring.
The exact particle size distribution of the μ ring is unknown, but the strong backscattering component suggests that the ring population is dominated by tiny particles. Moreover, near-infrared observations show that the μ ring is very blue, which is indicative of a steep size distribution dominated by smaller grains (de Pater et al. 2006).
The alignment between the orbit of Mab and the ring peak renders the satellite a natural candidate to be the source of dust particles. Because Mab is quite small, about 12 km in radius, geological activity as found in Enceladus is unlikely (Spahn et al. 2006). Therefore an alternative dust delivery method is required to explain the existence of the μ ring. A plausible mechanism is the generation of particles through impacts of micrometeorites onto the surface of the satellite, which could eject new debris to populate the ring.
Here we investigate the efficiency of the hypervelocity impacts of interplanetary dust particles (IDPs) onto Mab’s surface to provide material for the μ ring, and the possibility of keeping the ring in a steady state when perturbative forces are taken into account.
Initially we examine the effects of disturbing forces that can act upon dust grains, and through numerical simulations we compute the lifetime of a particle after its ejection from Mab’s surface (Sect. 2). In Sect. 3 we present a method to determine the mass production rate given the IDPs flux and the characteristics of the satellite. An estimate of the ring mass is presented in Sect. 4, along with the time necessary to populate the ring. Finally, Sect. 5 contains a summary and discussion of our results.
2. Numerical simulations
2.1. Disturbing forces
Circumplanetary dust grains can be influenced in their evolution and fate by disturbing forces, each one with a distinctive effect on the orbit of the particles (Burns et al. 2001): the planetary oblateness and electromagnetic forces manifest their effects in the precession of the orbital pericentre, while the solar radiation pressure and the solar tide act mainly in the excitation of the eccentricity of the particle. On the other hand, the orbital energy can be altered by the atmospheric drag, the Poynting-Robertson component of the solar radiation force, and the plasma drag, which leads to a change in the semimajor axis.
For the μ ring environment not all these forces play a significant role in the dynamics of the particles. The atmospheric drag can be safely ignored, since the ring is far away from the exosphere of Uranus, and the solar tide affects only particles located much farther out than the outer edge of the μ ring. Slowly acting forces, such as the Poynting-Robertson drag and the plasma drag, are not determinant for the fate of the particles, and therefore contribute only negligibly to the timescale we are going to analyse.
The equations of motion of a charged particle orbiting through a planetary magnetic field B require knowing the spherical harmonic coefficients of the multipolar expansion of B. A meaningful representation of the shifted and inclined magnetic field of Uranus requires an expansion up to octupole terms, which is not well determined (Connerney 1993). Therefore, we are unable to take the effects of this force into account in our model.
The effects of the other two remaining perturbations, the planetary oblateness and the solar radiation pressure, were addressed in a previous paper (Sfair & Giuliatti Winter 2009). Using numerical simulations, we analysed the combined effect of both forces on the orbital evolution of an ensemble of micrometic particles evenly spaced across the radial extension of the μ ring, taking into account the gravitational perturbation of Puck and Mab. Our results showed that the likely outcome for the ring particles is the collision with nearby satellites on a timescale of hundreds of years.
2.2. Initial conditions and numerical model
To mimic the scenario in which Mab is the source the of ring dust particles, we studied the dynamics of grains initially ejected from the satellite, which is different from the initial conditions of Sfair & Giuliatti Winter (2009).
If we assume that the dust particles have no memory of their previous history, their evolution after the ejection can be treated as an independent process. In this section we analyse how the orbit of the particles evolves under a combination of the gravity force from the planet and the closest satellites, as well as the solar radiation force.
Voyager data indicate that the μ ring is brighter for high phase angles (SL06), a strong evidence that the ring is composed of a population of micrometric-sized particles. Since no information regarding the size distribution of the grains has been published, we adopted in our numerical simulations spherical particles of pure ice with 1, 3, 5, and 10 μm in radius as a representative set.
Another important parameter that has to be determined is the velocity vej at which the dust was ejected after the impact of the IDP. In contrast to the continuous distribution that have been proposed (Krüger et al. 2000; Krivov et al. 2003), we chose the discrete values of 10, 50, and 100 m/s for vej, which correspond to approximately 1, 5, and 10 times the escape velocity vesc of Mab, respectively. The lower cut-off is the escape velocity, since any dust particle ejected with v < vesc would not be able to leave the vicinity of the parent body. The upper limit was chosen in such a way that most of the significant values were covered when compared to the continuous distributions.
For each combination of particle size and ejection velocity, a sample of 360 particles are uniformly ejected from the whole surface of Mab. There is no dependence between the ejecta velocity and the size of the grain throughout (Nakamura & Fujiwara 1991), and the trajectories are straight lines normal to the surface of the satellites. These assumptions simplify the problem by avoiding the introduction of additional parameters without losing any information regarding the general picture of the dust generation process.
All bodies are orbiting an oblate Uranus, and once the dust is released from the surface of the satellite, they are subjected to the gravitational influence of Mab and Puck. The physical radius of the satellites, as well their orbital elements, were derived from SL06. The density of both satellites is unknown, therefore we assumed the value of 1.3 g/cm3, the same as Miranda. For the planet, the parameters (mass, radius and gravity coefficients) were extracted from Murray & Dermott (1999).
Our model also includes the components of the solar radiation force (SRF) as described in Sfair & Giuliatti Winter (2009), assuming particles of ideal material. Effects of planetary shadow and of the light reflected from the planet were not taken into account, since their contribution to the evolution of the particles is secondary when compared to the effects of direct illumination (Hamilton 1993). To integrate the equations of motion we used the Burlish-Stöer algorithm available in the Mercury package (Chambers 1999), and for each particle the integration was carried out until a collision was detected.
2.3. Results
After the ejection, the initial orbit of the particles follows the orbit of Mab. Several orbital periods later, the ejecta forms a narrow tube around the source satellite, which increases in spread with increasing ejection velocity.
The next stage of the orbital evolution is determined by the effects of the radiation pressure component of the SRF, which leads to an increase in the eccentricity of the particles and has only a negligible effect on the orbital inclination. Even when the oblateness of the planet is taken into account, the maximum eccentricity can reach values as high as 0.13 for the 1 μm particle with an initial velocity equal to the escape velocity of Mab. For comparison, with the same velocity the eccentricity of a particle of 10 μm can reach the value of 0.01.
With increasing eccentricity, the particles are scattered within a wide radial extension and the width of the region a particle orbit covers is determined by the amplitude of e. The 1 μm grains, which are more affected by the solar radiation, are spread along a ~24 000 km wide region, which is comparable to the width of the μ ring, while larger grains are confined to narrower regions and remain in the vicinity of Mab.
Owing to the variation of the orbital radius of the particles, their orbits can cross the orbit of Mab or Puck, which may result in a collision with the satellites. Table 1 summarises the fate of the particles of different sizes for the three values of vej assumed, and the mean time 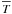 when the collisions occur.
when the collisions occur.
Fate of the particles.
The fate of the ejected particles is the collision with a satellite, and for all but the 1 μm sized particles the only sink is Mab. This is a consequence of the amplitude the eccentricity can reach: only the smaller particles reach sufficient high values of e for the pericentre to cross the orbit of the inner satellite, Puck. For those particles that reach the region of Puck, the larger number of collisions with the satellite is expected since it is almost seven times larger than Mab in radius.
Even with the oscillation of the eccentricity caused by the radiation pressure and the eventual close encounters with Mab, which may cause “jumps” of the semimajor axis, the 3, 5 and 10 μm particles remain in the vicinity of Mab. This behaviour explains the similarity of collision times for all values of vesc, since for all these sizes of particle the orbit constantly intercepts the orbit of Mab.
The same argument can explain the similarity of the collision times for all particle sizes, independently of the velocity of ejection. It is worth to point out that for all cases 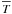 is not shorter than hundreds of years, even for smaller particles.
is not shorter than hundreds of years, even for smaller particles.
3. Dust production by hypervelocity impacts
In this section we present an algorithm that can be used to estimate how much mass can be supplied to the ring through the impact of IDPs onto the surface of a satellite.
To determine the amount of dust that could be created it is necessary to characterise the impactors and the targets. Some difficulties arise from the lack of information about the satellites and the IDP environment at the outer solar system, therefore the derived quantities should be taken as an estimate.
3.1. Flux
Krivov et al. (2003), based on previous works of Divine (1993) and Grun et al. (1985), determined the flux of IDPs at the Jovian and Saturnian systems. Following a similar procedure and including the contribution of a population of objects from the Kuiper belt, Porter et al. (2010) settled the value of 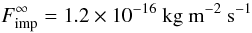 (1)for the mass flux of impactors at the Uranus heliocentric distance, because it is measured far from the planet (hence the ∞ superscript). This is the value we adopted in our calculations, but it is important to recall that this is merely an estimate, since there is not any direct measurement of the flux beyond Saturn.
(1)for the mass flux of impactors at the Uranus heliocentric distance, because it is measured far from the planet (hence the ∞ superscript). This is the value we adopted in our calculations, but it is important to recall that this is merely an estimate, since there is not any direct measurement of the flux beyond Saturn.
As the IDPs come close to the planet, their velocity 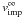 is enhanced due to the gravitational focusing, thus the mean velocity of the particles vimp with respect to the planet at the satellite distance a is given by
is enhanced due to the gravitational focusing, thus the mean velocity of the particles vimp with respect to the planet at the satellite distance a is given by  (2)where G is the gravitational constant and Mp is the planet mass. For the velocity of the IDPs far from Uranus we have assumed
(2)where G is the gravitational constant and Mp is the planet mass. For the velocity of the IDPs far from Uranus we have assumed 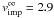 km s-1, given by the planet’s orbital velocity times
km s-1, given by the planet’s orbital velocity times  , where e ≈ i ≈ 0.3, the same formula as adopted by Porter et al. (2010). This equation, which differs from the model of Divine (1993), relies on the hypothesis that the IDP flux at Uranus comes from the Edgeworth-Kuiper belt. A more detailed discussion can be found in Liou & Zook (1999).
, where e ≈ i ≈ 0.3, the same formula as adopted by Porter et al. (2010). This equation, which differs from the model of Divine (1993), relies on the hypothesis that the IDP flux at Uranus comes from the Edgeworth-Kuiper belt. A more detailed discussion can be found in Liou & Zook (1999).
The spatial density of the dust nimp exceeds the density far from the planet, 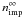 . Both values are related by (Colombo et al. 1966)1
. Both values are related by (Colombo et al. 1966)1![\begin{eqnarray} \frac{n_{\rm imp}}{n_{\rm imp}^\infty} =\frac{1}{2} \frac{v_{\rm imp}}{v_{\rm imp}^\infty} + \frac{1}{2} \left[ \left( \frac{v_{\rm imp}}{v_{\rm imp}^\infty} \right)^2 - \left( \frac{R_{\rm p}}{a}\right)^2 \left(1+\frac{2GM_{\rm p}}{R_{\rm p}\left(v_{\rm imp}^\infty\right)^2}\right) \right]^{1/2}, \label{E-nratio} \end{eqnarray}](/articles/aa/full_html/2012/07/aa17346-11/aa17346-11-eq38.png) (3)where Rp is the radius of the planet.
(3)where Rp is the radius of the planet.
To determine the mass flux Fimp at the satellite distance, one can combine the previous equations resulting in 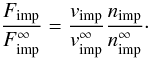 (4)All the above assumptions imply an isotropic flux of projectiles and do not take into account the motion of the satellite. This motion periodically changes the relative velocity among the satellite and the IDPs, and results in a time-dependent process, which is beyond the scope of this work.
(4)All the above assumptions imply an isotropic flux of projectiles and do not take into account the motion of the satellite. This motion periodically changes the relative velocity among the satellite and the IDPs, and results in a time-dependent process, which is beyond the scope of this work.
3.2. Target characterisation and mass production
The efficiency of dust generation depends on the characteristics of the surface of the satellite. It can be measured by the ejecta yield Y, defined as the ratio of the total ejecta mass to the mass of the impactor.
Koschny & Grün (2001) performed several experimental tests and derived an expression to compute the value of Y for targets with different ice-silicate mixtures as a function of the speed of the impactors vimp and their typical mass mimp. When extrapolated to a satellite that is covered by pure ice, the expression for the yield can be written as 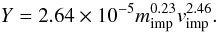 (5)This value is calculated assuming a typical impactor with mass of 10-8 kg, corresponding to a grain of ~100 μm in radius, and the velocity given by Eq. (2). With this information, along with the IDPs flux (Eq. (4)), the mass production rate for a satellite with radius r and cross section area S = πr2 can be expressed as
(5)This value is calculated assuming a typical impactor with mass of 10-8 kg, corresponding to a grain of ~100 μm in radius, and the velocity given by Eq. (2). With this information, along with the IDPs flux (Eq. (4)), the mass production rate for a satellite with radius r and cross section area S = πr2 can be expressed as 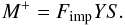 (6)Using the physical parameters of Mab described in Sect. 2, along with the parameters mentioned previously, the evaluation of the Eqs. (2)–(6) is straightforward. The values obtained are summarised in Table 2.
(6)Using the physical parameters of Mab described in Sect. 2, along with the parameters mentioned previously, the evaluation of the Eqs. (2)–(6) is straightforward. The values obtained are summarised in Table 2.
Parameters.
4. Comparative analysis
In Sect. 2 we analysed the orbital evolution of the particles ejected from the surface of Mab, and in Sect. 3 we presented a simplified model to estimate the mass production rate by hypervelocity impacts onto the satellite. Combining both results, we can determine if the production process is efficient enough to keep the ring in a steady state.
First, it is necessary to estimate the mass of the μ ring. Because the ring presents a distinguished triangular profile, it is reasonable to consider that most of the mass is concentrated within a region around the peak of the profile. The distribution of the dust grains was assumed to follow a power-law in the form n(s)ds = Cs − q, with n(s)ds being the number of particles in the size range [s,s + ds] . The constant C must be determined using the optical depth of the ring, and the index is taken as q = 3.5, the same as used by Colwell & Esposito (1993) for the dust region within the main ring system of Uranus.
For an optical depth of τ = 8.5 × 10-6 (SL06), the mass of a dust sheet 300 km wide at the orbit of Mab is m = 6.6 × 106 kg, assuming the particle size distribution extending from 1 μm up to 10 μm. The value of m is quite sensitive to the size range and the ring width, so it should be taken with a uncertainty of at least one magnitude.
To produce this amount of material would take roughly 80 years, assuming the mass rate production found in Table 2. When we compare the time necessary to accumulate material produced by ejecta and the lifetime of the particles (Table 1), we see that the grains remain within the ring region for a time much longer than the necessary to populate the ring.
The total mass of the ring and the accumulation time carry a uncertainty mainly from the flux determination and from the ring width assumed, but even if these values are underestimated, they are at least one magnitude lower than the time necessary to remove the grains through collisions. This becomes clearer when we recall that the time in Table 1 represents only an average value, and it can easily take up to 6.300 and 13.000 years to remove all particles of 1 and 10 μm in radius, respectively.
We also performed a similar analysis for the production rate of ejecta from the inner satellite Puck, which is considerably larger than Mab. Our results showed that 150 g of material per second can be generated by the impacts of IDPs on its surface, however, just a very small fraction of the total mass is ejected with a sufficiently high velocity to surpass the escape velocity of the satellite. Thus the ring around Puck would be fed at a slower rate than the value found at Mab. Furthermore, given the higher initial velocity, the particles ejected from Puck are broadly spread, resulting in an optical depth at least two orders of magnitude lower than the peak of the μ ring.
5. Discussion
Impacts of micrometeorites on Mab’s surface release dust grains that escape from the satellite. After the ejection the particles are under the influence of gravitational effects of the nearby satellites and the solar radiation pressure. Since the strength of this dissipative force depends on the grain size, there is a segregation according to the particle radius: larger particles remain in the vicinity of the satellite, while the smaller grains are spread within the radial extension of the μ ring. This mechanism can explain the blue colour of the ring observed by de Pater et al. (2006).
Beyond acting as the source for the ring, Mab plays the role of a sink for the particles once most of them cross the orbit of the satellite continuously and eventually collide with it. The main responsible mechanism for this behaviour is the excitation of the eccentricity caused by the radiation pressure.
To keep the ring population in a steady state, an equilibrium between the dust delivery process and the removal of particles due to the collisions with the satellites is necessary. Although our model can reproduce a ring with a triangular profile and radial extent comparable to the μ ring, we are not able to reach a steady state that matches the current optical depth of the ring derived by SL06. In our scenario the number of particles continuously increases, and therefore the ring would become gradually brighter. If one seeks for a ring with a constant particle population, a wide range of hypotheses emerges, for instance: i) the initial assumption for the flux of interplanetary meteoroids can be overrated; ii) the effects of electromagnetic forces within the μ ring environment were not taken into account; iii) the existence of other sinks (and eventually sources) of the particles.
The current knowledge of the flux of impactors at Uranus is merely an estimate based on the extrapolation of measurements up to the orbit of Saturn, and any change in the IDP flux can modify the dust production rate. It is also possible, if unlikely, that the flux is not continuous and the ring is a transient structure generated by high-energy impacts.
Concerning the electromagnetic force, depending on the charge of the grains and their motion relative to the magnetic field, this force could diminish the pericentre precession induced by the planetary oblateness, leading to an increase of the particle eccentricity. With high values of e the lifetime of the dust grains is expected to be shorter, since the orbit of the particles intercepts the orbit of the neighbour satellites more frequently.
The possible existence of additional and yet unseen moonlets could be an additional source and/or sink of particles. However, this possibility was not considered for two reasons: (a) the observational threshold discards any moonlet larger than 5 km in radius, which means that very many bodies would be necessary to produce an amount of ejecta comparable to Mab’s; and (b) a sample of numerical simulations showed that the moonlets can be located in a wide region between Mab and Puck, but to reproduce the triangular ring profile, these satellites should be close to the orbit of Mab, which raises the question about their stability. We are currently investigating this question to provide the more detailed analysis that is required here.
To compute the exact dust balance within the μ ring we need a deeper understanding of the ring environment. A more accurate model of the IDPs population at Uranus is expected from NASA’s New Horizons spacecraft data. Future observations can improve our knowledge of the satellite’s surface composition. All these new data will provide better constraints to refine our model.
This equation is given by Spahn et al. (2006), who corrected a misprint in the original paper.
Acknowledgments
The authors thank B. Sicardy for helpful discussions, and the anonymous referee for improving the presentation and content of the paper. This work was supported by FAPESP (Proc. 2006/04997-6), and INCT – Estudos do Espaço.
References
- Burns, J., Hamilton, D., & Showalter, M. 2001, in Interplanetary Dust, ed. E. Grün et al. (Berlin: Springer Verlag), 641 [Google Scholar]
- Chambers, J. E. 1999, MNRAS, 304, 793 [NASA ADS] [CrossRef] [MathSciNet] [Google Scholar]
- Colombo, G., Shapiro, I. I., & Lautman, D. A. 1966, J. Geophys. Res., 71, 5719 [NASA ADS] [CrossRef] [Google Scholar]
- Colwell, J. E., & Esposito, L. W. 1993, J. Geophys. Res., 98, 7387 [NASA ADS] [CrossRef] [Google Scholar]
- Connerney, J. E. P. 1993, J. Geophys. Res., 98, 18659 [NASA ADS] [CrossRef] [Google Scholar]
- de Pater, I., Hammel, H. B., Gibbard, S. G., & Showalter, M. R. 2006, Science, 312, 92 [Google Scholar]
- Divine, N. 1993, J. Geophys. Res., 98, 17029 [NASA ADS] [CrossRef] [Google Scholar]
- Grun, E., Zook, H. A., Fechtig, H., & Giese, R. H. 1985, Icarus, 62, 244 [NASA ADS] [CrossRef] [Google Scholar]
- Hamilton, D. P. 1993, Icarus, 101, 244 [NASA ADS] [CrossRef] [Google Scholar]
- Koschny, D., & Grün, E. 2001, Icarus, 154, 402 [NASA ADS] [CrossRef] [Google Scholar]
- Krivov, A. V., Sremčević, M., Spahn, F., Dikarev, V. V., & Kholshevnikov, K. V. 2003, Planet. Space Sci., 51, 251 [NASA ADS] [CrossRef] [Google Scholar]
- Krüger, H., Krivov, A. V., & Grün, E. 2000, Planet. Space Sci., 48, 1457 [NASA ADS] [CrossRef] [Google Scholar]
- Liou, J. C., & Zook, H. A. 1999, AJ, 118, 580 [NASA ADS] [CrossRef] [Google Scholar]
- Murray, C. D., & Dermott, S. F. 1999, Solar system dynamics (Cambridge University Press) [Google Scholar]
- Nakamura, A., & Fujiwara, A. 1991, Icarus, 92, 132 [NASA ADS] [CrossRef] [Google Scholar]
- Porter, S. B., Desch, S. J., & Cook, J. C. 2010, Icarus, 208, 492 [NASA ADS] [CrossRef] [Google Scholar]
- Sfair, R., & Giuliatti Winter, S. M. 2009, A&A, 505, 845 [NASA ADS] [CrossRef] [EDP Sciences] [Google Scholar]
- Showalter, M. R., & Lissauer, J. J. 2006, Science, 311, 973 [Google Scholar]
- Spahn, F., Albers, N., Hörning, M., et al. 2006, Planet. Space Sci., 54, 1024 [NASA ADS] [CrossRef] [Google Scholar]
All Tables
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


