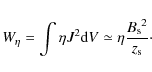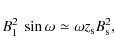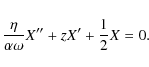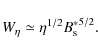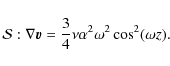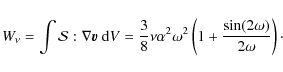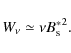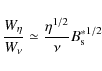| Issue |
A&A
Volume 501, Number 2, July II 2009
|
|
|---|---|---|
| Page(s) | 755 - 760 | |
| Section | The Sun | |
| DOI | https://doi.org/10.1051/0004-6361/200911784 | |
| Published online | 13 May 2009 | |
Anisotropic viscous dissipation in three-dimensional magnetic merging solutions
I. J. D. Craig - Y. E. Litvinenko
Department of Mathematics, University of Waikato, PB 3105, Hamilton, New Zealand
Received 4 February 2009 / Accepted 23 April 2009
Abstract
Aims. We consider viscous and resistive energy dissipation in the flaring solar corona.
Methods. We compute energy dissipation rates, associated with magnetic merging in three dimensions. We examine an exact 3D solution for steady magnetic merging in a viscous resistive incompressible plasma. We use the Braginskii stress tensor to model viscous effects and derive scalings for the resistive and viscous dissipation rates. We evaluate the dissipation rates for typical parameters of solar active regions.
Results. Large-scale advective flows required to sustain resistive current sheets are shown to be associated with significant viscous losses. For plausible dimensionless resistivities (inverse Lundquist numbers), whether classical
![]() or anomalous
or anomalous
![]() ,
viscous loss rates greatly exceed the resistive loss rates of the current layer and can approach flare-like rates of 1028 erg s-1.
,
viscous loss rates greatly exceed the resistive loss rates of the current layer and can approach flare-like rates of 1028 erg s-1.
Key words: magnetohydrodynamics (MHD) - sun: flares - sun: magnetic fields
1 Introduction
One of the outstanding problems in astrophysics is explaining the explosive energy release of solar and stellar flares. Although magnetic reconnection is the accepted release mechanism, the weak electrical resistivity in the solar corona leads to energy-loss rates that are generally too slow to account for flare observations (Priest & Forbes 2000). To what extent the presence of other physical ingredients - such as Hall effects - can increase the reconnection rate is currently the focus of intense theoretical investigation (e.g., Birn et al. 2001; Knoll & Chacon 2006; Litvinenko 2009 and references therein).
A common element of virtually all reconnection models is the presence of strong Alfvénic exhaust flows. Observational studies (e.g., McKenzie & Hudson 1999; Asai et al. 2004) indeed confirm that strong outflows are correlated with impulsive hard X-ray bursts in flares. Since typical merging models also require strong advective inflows to carry magnetic field lines into the reconnection region, it is natural to ask: How significant are the viscous energy losses that derive from velocity fields associated with magnetic merging? It is this question that motivates the present study.
As background, we note that Hollweg (1985, 1986) has already argued that viscous damping is likely to dominate resistive dissipation under a wide range of coronal conditions. In particular, because coronal plasmas are only weakly resistive, huge gradients in the magnetic field - typically requiring near-singular current sheets - are required to provide appreciable Ohmic dissipation rates. By contrast, viscous dissipation can achieve significant rates with relatively modest gradients in the velocity field of the plasma. This point has recently been emphasized by two planar reconnection studies, namely, the incompressible steady merging model of Litvinenko (2005) and the compressible X-point collapse analysis of Craig (2008).
Consider, for instance, a coronal velocity field of global scale ![]() .
In the case of Alfvénic speeds the vorticity will scale as
.
In the case of Alfvénic speeds the vorticity will scale as
![]() and therefore the global viscous
losses can be estimated as
and therefore the global viscous
losses can be estimated as
Taking a classical shear viscosity
It would be a major deception, however, to apply an estimate based on Eq. (1) to magnetic merging in the solar corona. This is because an accurate treatment of viscous dissipation in a magnetically stratified plasma requires a tensor description that effectively suppresses shear viscosity components (Braginskii 1965). In the case of planar merging driven by a stagnation point flow, the bulk viscosity provides dissipation rates that are comparable to those based on the classical shear viscosity (Litvinenko 2005). But if an axial field, rather than a planar field, were advected by the flow, the viscous dissipation would be entirely negligible (see Sect. 2.2).
These considerations suggest that dissipation due to bulk viscosity may depend in a complicated way on the details of the field geometry. In the present paper we extend recent studies that incorporate bulk viscosity within planar magnetic geometries (Litvinenko 2005; Craig 2008). Specifically we analyze the resistive and viscous dissipation provided by an exact steady three-dimensional (3D) magnetohydrodynamic (MHD) solution for incompressible magnetic merging (Craig & Watson 2000). We also generalize our previous calculation (Litvinenko 2005) by determining the effect of the magnetic field pile-up at the entrance to the reconnecting current sheet on the resistive and viscous dissipation rates.
The incompressible MHD equations are introduced in Sect. 2 where we discuss energy losses due to resistive and viscous effects under typical coronal conditions. Our main results are derived in Sect. 3 where we introduce a 3D ``fan'' merging solution, based on an axisymmetric velocity field. This model is used to provide concrete scaling laws for the resistive and viscous losses. In Sect. 4 we present our conclusions.
2 Viscous and resistive dissipation in magnetized coronal plasmas
2.1 Introduction
Our aim is to construct a three-dimensional magnetic merging solution
that allows us to evaluate the global viscous and resistive losses. To do this we work with the incompressible MHD equations, scaled with respect to typical solar coronal values for field strength
![]() G, size scale
G, size scale
![]() cm, and number density
cm, and number density
![]() cm-3. Times are measured in units of
cm-3. Times are measured in units of
![]() where
where
![]() cm s-1 is the Alfvén speed. The global energy loss rate has the units
cm s-1 is the Alfvén speed. The global energy loss rate has the units
![]() erg s-1.
erg s-1.
Energy losses from the source volume are controlled by two small parameters,
the dimensionless resistivity
![]() and the dimensionless plasma viscosity
and the dimensionless plasma viscosity
![]() .
For a collisional plasma of temperature T = 106 K
with conductivity
.
For a collisional plasma of temperature T = 106 K
with conductivity
![]() (Spitzer 1962),
(Spitzer 1962),
![]() is
an inverse Lundquist number of magnitude
is
an inverse Lundquist number of magnitude
Viscous losses are determined by an inverse of the traditional Reynolds number. For a plasma of mass density
From now on, we work only with dimensionless quantities, so we drop the superscripts and use simply
The fact that
![]() suggests that viscous dissipation is
likely to dominate resistive damping for typical coronal plasmas.
This dominance can be expected to increase in active region plasmas where
plasma temperatures can exceed several million degrees.
We should remember, however, that energy that derives
from the topological simplification of the magnetic field can only be
released resistively via magnetic reconnection. A
further complication is that viscous effects become
highly anisotropic in magnetized coronal plasmas (Braginskii 1965; Hollweg 1986). Isotropy breaks down when the proton mean free path exceeds the gyroradius:
suggests that viscous dissipation is
likely to dominate resistive damping for typical coronal plasmas.
This dominance can be expected to increase in active region plasmas where
plasma temperatures can exceed several million degrees.
We should remember, however, that energy that derives
from the topological simplification of the magnetic field can only be
released resistively via magnetic reconnection. A
further complication is that viscous effects become
highly anisotropic in magnetized coronal plasmas (Braginskii 1965; Hollweg 1986). Isotropy breaks down when the proton mean free path exceeds the gyroradius:
![]() ,
where
,
where
![]() is the proton cyclotron frequency and
is the proton cyclotron frequency and
![]() is the mean time (in seconds) between momentum-changing collisions. Typical coronal parameters lead to
is the mean time (in seconds) between momentum-changing collisions. Typical coronal parameters lead to
![]() ,
which confirms that anisotropic viscosity must be used in almost all coronal applications.
,
which confirms that anisotropic viscosity must be used in almost all coronal applications.
2.2 Incompressible MHD equations
We adopt the 3D incompressible MHD equations for the veloc- ity field
![]() and the magnetic field
and the magnetic field
![]() .
These comprise Maxwell's equations and the momentum and continuity equations:
.
These comprise Maxwell's equations and the momentum and continuity equations:
Here P is the plasma pressure,
As already mentioned, the classical expression for the viscous tensor ![]() in an incompressible fluid, namely
in an incompressible fluid, namely
is not accurate for a magnetized collisional plasma in which the proton mean free path greatly exceeds the proton gyroradius. In such cases the strong field form (Braginskii 1965) is appropriate:
Here
For completeness, we should mention that the stress tensor ![]() generally contains an extra term
generally contains an extra term ![]()
![]() that accounts for the
finite compressibility of the plasma. This term provides an avenue for bulk viscous dissipation (Sect. 3.5) that is not represented in the present incompressible analysis.
that accounts for the
finite compressibility of the plasma. This term provides an avenue for bulk viscous dissipation (Sect. 3.5) that is not represented in the present incompressible analysis.
2.3 Global energy losses
Before considering explicit solutions, it is instructive to obtain a general expression for the global energy losses, valid for all forms of viscosity.
To do this we dot the primitive momentum Eq. (4) with ![]() and permute
and permute
![]() ,
making use of the identity
,
making use of the identity
| (9) |
Using the induction equation to re-express the final term we find that
where
In what follows we are interested in steady state solutions
(
![]() )
in a volume V, sustained by advective
flows through the boundary. In this case the resistive and
viscous losses (the right-hand side terms in Eq. (10))
can be integrated over V and the divergence term
evaluated as a surface contribution. We see that
energy dissipation in a steady solution must be balanced by
the Poynting flux and the work done by viscous stresses.
)
in a volume V, sustained by advective
flows through the boundary. In this case the resistive and
viscous losses (the right-hand side terms in Eq. (10))
can be integrated over V and the divergence term
evaluated as a surface contribution. We see that
energy dissipation in a steady solution must be balanced by
the Poynting flux and the work done by viscous stresses.
3 The visco-resistive merging solution
3.1 Merging solutions in 3D
It is now recognised that 3D magnetic merging at an isolated null requires the presence of highly localized current structures in the form of current sheets or quasi-cylindrical current tubes. Such structures account for resistive dissipation in so-called
``fan'' and ``spine'' reconnection models. More specifically, fan solutions can be derived using a form
that defines a current sheet in the fan plane z = 0. Spine solutions, in which
3.2 Velocity field for fan merging
We now ask: what kind of flows can support a steady fan merging solution?
Note that the fan current structure
![]() leads to a Lorentz force that is irrotational. This implies that any velocity field
that satisfies the curled form of the momentum Eq. (4), namely
leads to a Lorentz force that is irrotational. This implies that any velocity field
that satisfies the curled form of the momentum Eq. (4), namely
provides a possible solution.
Now, from Craig & Watson (2000), we know that
provides an exact, axisymmetric solution in the absence of viscous effects. Here the parameter
The Braginskii tensor retains only a z-dependence and a simple check shows that
The solution is completed by solving Eq. (5) for the magnetic field components:
This system, first derived by Craig & Watson (2000), describes the merging of magnetic field lines, washed in through the planes
3.3 Computed merging solutions
The solution outlined above is exact, but to obtain plausible
dissipation rates we must ensure
that magnetic field is coupled to the flow in a physically realistic
manner. In practice, this can be achieved by matching
the magnetic pressure in the current sheet to the dynamic pressure of
the plasma exhaust-a prescription that provides, for a specified
peak field in the current layer ![]() ,
a well defined merging
rate (Litvinenko & Craig 1999, 2000; Craig & Watson 2000). In the results below the peak field
,
a well defined merging
rate (Litvinenko & Craig 1999, 2000; Craig & Watson 2000). In the results below the peak field ![]() is identified with
the exhaust speed in the fan (
is identified with
the exhaust speed in the fan (
![]() )
at unit radial distance
from the origin. The solution is obtained by ``shooting'' using
Eqs. (15) and (16), adjusting gradients at the
origin until the required field amplitude is achieved over
the inflow region.
)
at unit radial distance
from the origin. The solution is obtained by ``shooting'' using
Eqs. (15) and (16), adjusting gradients at the
origin until the required field amplitude is achieved over
the inflow region.
Figure 1 shows a typical solution plotted along the inflow z-axis
for the case
![]() ,
,
![]() ,
,
![]() .
The
solution is dominated by a large scale advective region but there is a narrow current layer
overlying the origin that accounts for the bulk of the Ohmic
dissipation. Here we have modeled the current layer by taking
Y'(0) = 0 and varying X'(0) in the numerical integration of
Eqs. (15) and (16).
(Note for clarity that the fact Y'(0) = 0
is obscured in Fig. 1 by the strong variation in the solution
close to the origin). The X-field component is therefore dominant in the
region
.
The
solution is dominated by a large scale advective region but there is a narrow current layer
overlying the origin that accounts for the bulk of the Ohmic
dissipation. Here we have modeled the current layer by taking
Y'(0) = 0 and varying X'(0) in the numerical integration of
Eqs. (15) and (16).
(Note for clarity that the fact Y'(0) = 0
is obscured in Fig. 1 by the strong variation in the solution
close to the origin). The X-field component is therefore dominant in the
region
![]() ,
but this dominance is lost in regions approaching the outer boundary. It is also possible to
tune the wavenumber so that
,
but this dominance is lost in regions approaching the outer boundary. It is also possible to
tune the wavenumber so that
![]() .
This adjustment is minor (a few percent) and does not impact
significantly on the computed dissipation rates.
.
This adjustment is minor (a few percent) and does not impact
significantly on the computed dissipation rates.
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig1.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg84.png) |
Figure 1:
Inflow magnetic field and flow speed against z for the parameters
|
| Open with DEXTER | |
In view of the smallness of the coronal resistivity, in practice
it is important to understand how the solution behaves over
an extended range of ![]() .
In Fig. 2 we plot the global resistive
and viscous dissipation rates over the resistive range
.
In Fig. 2 we plot the global resistive
and viscous dissipation rates over the resistive range
![]() for the flow strength
for the flow strength
![]() .
We obtain the global dissipation rates by integrating the volumetric rates,
defined by Eq. (10):
.
We obtain the global dissipation rates by integrating the volumetric rates,
defined by Eq. (10):
![]() and
and
![]() .
The dimensionless Braginskii viscosity coefficient was fixed at
.
The dimensionless Braginskii viscosity coefficient was fixed at
![]() ,
which corresponds to an active region temperature
of around
,
which corresponds to an active region temperature
of around
![]() K. With these parameters we see that the viscous losses
exceed the resistive losses in the physically relevant regime
K. With these parameters we see that the viscous losses
exceed the resistive losses in the physically relevant regime
![]() .
Note that, although
.
Note that, although ![]() approximates an
approximates an
![]() scaling,
scaling, ![]() is effectively independent of the resistivity. The calculated rate
is effectively independent of the resistivity. The calculated rate
![]() corresponds to an energy loss rate exceeding 1028 erg s-1 - and such rates can legitimately be multiplied by four since only one quadrant, say
x>0, y>0, of the full merging geometry has been considered for the integration.
corresponds to an energy loss rate exceeding 1028 erg s-1 - and such rates can legitimately be multiplied by four since only one quadrant, say
x>0, y>0, of the full merging geometry has been considered for the integration.
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig2.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg94.png) |
Figure 2:
Scaling of resistive and viscous dissipation rates over the resistive range
|
| Open with DEXTER | |
As we argued previously (Litvinenko & Craig 1999, 2000),
although any solution is specified by four input parameters - ![]() ,
,
![]() ,
,
![]() and
and ![]() - two physically based restrictions should be imposed on the model:
first, the exhaust speed of the flow should be comparable to the Alfvén speed based on the peak magnetic field
- two physically based restrictions should be imposed on the model:
first, the exhaust speed of the flow should be comparable to the Alfvén speed based on the peak magnetic field ![]() ;
second, the field
;
second, the field ![]() in the current sheet must saturate at some level
in the current sheet must saturate at some level
![]() ,
independent of the values of small
dissipation parameters
,
independent of the values of small
dissipation parameters ![]() and
and ![]() .
We refer to these as the equalization (i) and saturation (ii) conditions. These conditions lead to the maximum achievable
dissipation rates and thus allow us to test the viability of the model in application to flare-like energy release.
.
We refer to these as the equalization (i) and saturation (ii) conditions. These conditions lead to the maximum achievable
dissipation rates and thus allow us to test the viability of the model in application to flare-like energy release.
Although condition (i) is built into the numerical results,
the absence of condition (ii) suggests that dissipation rates
could be increased indefinitely by allowing larger values of the sheet field
![]() .
By taking an upper limit for
.
By taking an upper limit for ![]() we can restrict
both the merging rate and the exhaust flow to physically plausible
levels. More generally, by applying conditions (i) and (ii)
systematically, the dependence of the dissipation rates
on
we can restrict
both the merging rate and the exhaust flow to physically plausible
levels. More generally, by applying conditions (i) and (ii)
systematically, the dependence of the dissipation rates
on ![]() and
and ![]() can be effectively eliminated: in this
case the scalings are specified by
can be effectively eliminated: in this
case the scalings are specified by ![]() and
and ![]() and the saturated peak field
strength
and the saturated peak field
strength
![]() .
.
3.4 Resistive and viscous scalings
Consider first the Ohmic dissipation rate ![]() .
Since the merging solution always
comprises an outer advection region
.
Since the merging solution always
comprises an outer advection region
![]() and a narrow resistive current layer
and a narrow resistive current layer
![]() we can write
we can write
In order to estimate the resistive layer thickness
assuming
The properties of the current layer can be specified using
the equalization and saturation conditions, introduced in the
previous section. The equalization condition (i) follows from
the form of the solution (13) for the plasma flow:
| (19) |
In the resistive layer we can assume-by a suitable orientation of the magnetic field on the inflow boundary-that the X component of the field is dominant. Since the inflow is approximately linear in the region
The important point is the scaling of the current sheet thickness
Eliminating
If necessary, Eqs. (18) and (21) can be solved to give the values of
Now consider the bulk viscosity dissipation ![]() .
Using Eqs. (13) and (14), we find that
.
Using Eqs. (13) and (14), we find that
For
Since the bracketed term is of order unity in the interval
The dependence of
We see that
is likely to be 10-1 or smaller for any plausible resistivity
Finally we comment on the accuracy of the analytical scalings above. Figure 3 shows a comparison of ![]() ,
defined by the position of the peak field
,
defined by the position of the peak field ![]() ,
against
the approximate formula
,
against
the approximate formula
![]() of Eq. (18). The relative agreement is better than ten percent over a range of resistivities
of Eq. (18). The relative agreement is better than ten percent over a range of resistivities
![]() .
Thus the boundary layer arguments used to derive the scaling laws outlined above are remarkably well supported by the numerics.
.
Thus the boundary layer arguments used to derive the scaling laws outlined above are remarkably well supported by the numerics.
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig3.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg128.png) |
Figure 3:
Current sheet thickness based on measured position of the peak field (circles) against location (solid line with crosses) estimated using formula (18). Results are computed for
|
| Open with DEXTER | |
3.5 Discussion
The present results suggest that viscous losses are likely to dominate
resistive losses in magnetic merging solutions for all plausible
plasma resistivities. The possibility that an enhanced ``anomalous'' resistivity
can nullify this conclusion seems remote given that
enhancements of order 106 are the most that can be expected
(e.g., Litvinenko & Craig 2000 and references therein).
Even for ![]() as large as 10-8,
as large as 10-8, ![]() can exceed
can exceed
![]() only if
only if
![]() lies at the lower end of
its plausible range in the solar corona.
lies at the lower end of
its plausible range in the solar corona.
Possibly the main limitation of the present analysis is
the requirement of a global vortical flow to
drive the merging. Clearly, on physical grounds, the velocity
field should fall off substantially outside the solution domain
![]() .
Were this not the case then unphysically
large hydro-magnetic pressures would be required to
sustain the merging. Whether large-scale velocity fields
with the required properties are likely
to develop in the active solar corona - say, as a result
of flux emergence or filament eruption - is far from assured.
Yet it should be kept in mind that the present model represents
only one solution in a range of theoretical possibilities.
.
Were this not the case then unphysically
large hydro-magnetic pressures would be required to
sustain the merging. Whether large-scale velocity fields
with the required properties are likely
to develop in the active solar corona - say, as a result
of flux emergence or filament eruption - is far from assured.
Yet it should be kept in mind that the present model represents
only one solution in a range of theoretical possibilities.
Consider, for example, an X-point implosion within a closed
planar geometry. Reconnection
is driven by a rapid localization in both the ![]() and
and
![]() fields, and viscous dissipation
is enhanced by strong compressive effects that accompany the
collapse (Craig 2008). This physical picture is quite different
from that of the present model. Specifically, the present solution
does not rely on small length scales in the velocity field
in order to achieve significant bulk viscous losses.
Taking both models together, however, strongly suggests
that anisotropic viscous effects can lead to flare-like energy release
rates in a variety of magnetic merging geometries.
fields, and viscous dissipation
is enhanced by strong compressive effects that accompany the
collapse (Craig 2008). This physical picture is quite different
from that of the present model. Specifically, the present solution
does not rely on small length scales in the velocity field
in order to achieve significant bulk viscous losses.
Taking both models together, however, strongly suggests
that anisotropic viscous effects can lead to flare-like energy release
rates in a variety of magnetic merging geometries.
4 Conclusions
The present study has addressed the viscous and resistive
energy losses associated with an exact incompressible
magnetic-merging solution.
We have shown that large-scale advective flows, required to sustain
3D ``fan'' merging at an isolated neutral point, are subject to
significant viscous energy losses based on the bulk viscosity terms in the Braginskii viscous stress tensor. Specifically, for typical coronal parameters, the viscous dissipation rate can reach flare-like levels of 1028 erg s-1.
This rate dominates resistive losses for all plausible resistivities, whether classical
![]() or turbulent
or turbulent
![]() .
.
One curious feature of the present analysis is that the global viscous
losses seem almost independent of the rate of magnetic merging.
Certainly, when the peak magnetic field strength is fixed, the viscous loss rate is independent of the resistivity (Fig. 2). However,
for stronger field strengths ![]() ,
both the dynamic pressure of the plasma flow and the viscous loss rate are enhanced,
,
both the dynamic pressure of the plasma flow and the viscous loss rate are enhanced,
![]() .
.
Notably absent in the present solution is a small length
scale controlled by the plasma viscosity. For example, a hybrid
length scale
![]() is known to emerge in other
reconnection solutions, for example, those based on X-point
collapse, for both classical and anisotropic viscosities
(Craig et al. 2005; Craig 2008).
Although these compressible solutions are very
different from the models considered here (see Sect. 3.5),
they are consistent with fast viscous dissipation,
driven by strong localizations in the velocity field,
at least in the regime
is known to emerge in other
reconnection solutions, for example, those based on X-point
collapse, for both classical and anisotropic viscosities
(Craig et al. 2005; Craig 2008).
Although these compressible solutions are very
different from the models considered here (see Sect. 3.5),
they are consistent with fast viscous dissipation,
driven by strong localizations in the velocity field,
at least in the regime
![]() .
What the
present analysis confirms is that alternative solutions are possible in
which strong bulk viscous losses are not dependent on the development
of small length scales in the velocity field that drives the merging.
.
What the
present analysis confirms is that alternative solutions are possible in
which strong bulk viscous losses are not dependent on the development
of small length scales in the velocity field that drives the merging.
Our approach is limited in two respects, justifying future research.
First, we explored the viability of the 3D magnetic merging model
by calculating the maximum energy release rate, consistent with
physical requirements of optimization and saturation of the solution.
Flare observations should be used to investigate whether
the large-scale vortical flows, required to sustain the maximum
viscous dissipation rate, are indeed present in the flaring
solar corona. Second, we have used the leading bulk viscosity
term of the Braginskii viscous stress tensor in our analysis.
Because the expression is valid in a strong field limit,
our analysis cannot be extended to the neighborhood of a magnetic
null. This is not critical in the present analysis because the
viscous stress tensor is valid everywhere except for a thin strip
![]() of negligible measure,
which resides well inside the current sheet. However,
a more general form of the viscous stress tensor,
valid for arbitrary magnetic field strengths, should be employed
to generalize our results. One possible choice would be
the so-called Liley form of the stress tensor (e.g., Hosking & Marinoff 1973), although it is not clear whether analytical treatment would be possible in that case.
of negligible measure,
which resides well inside the current sheet. However,
a more general form of the viscous stress tensor,
valid for arbitrary magnetic field strengths, should be employed
to generalize our results. One possible choice would be
the so-called Liley form of the stress tensor (e.g., Hosking & Marinoff 1973), although it is not clear whether analytical treatment would be possible in that case.
Acknowledgements
This work was supported by NASA grant NNX08AG44G, NSF grants ATM-0734032 and ATM-0837915, and by a research fellowship from the Alexander von Humboldt Foundation. Discussions with Prof. Roger Hosking and a detailed, constructive report by an anonymous referee have been much appreciated. Joli Adams' encouragement to personalize the writing is acknowledged.
References
- Asai, A., Yoshimoto, T., Shimojo, M., & Shibata, K. 2004, ApJ, 605, L77 [NASA ADS] [CrossRef] (In the text)
- Birn, J., Drake, J. F., Shay, M. A., et al. 2001, J. Geophys. Res., 106, 3715 [NASA ADS] [CrossRef] (In the text)
- Braginskii, S. I. 1965, Rev. Plasma Phys. 1, 205 (In the text)
- Craig, I. J. D. 2008, A&A, 487, 1155 [NASA ADS] [CrossRef] [EDP Sciences] (In the text)
- Craig, I. J. D., & Fabling, R. B. 1996, ApJ, 462, 969 [NASA ADS] [CrossRef] (In the text)
- Craig, I. J. D., & Watson, P. G. 2000, Solar Phys., 194, 251 [NASA ADS] [CrossRef] (In the text)
- Craig, I. J. D., Litvinenko, Y. E., & Senanayake, T. 2005, A&A, 433, 1139 [NASA ADS] [CrossRef] [EDP Sciences] (In the text)
- Hollweg, J. V. 1985, J. Geophys. Res., 90, 7620 [NASA ADS] [CrossRef] (In the text)
- Hollweg, J. V. 1986, ApJ, 306, 730 [NASA ADS] [CrossRef] (In the text)
- Hosking, R. J., & Marinoff, G. M. 1973, Plasma Phys., 15, 327 [NASA ADS] [CrossRef] (In the text)
- Knoll, D. A., & Chacon, L. 2006, Phys. Rev. Lett., 96, 135001 [NASA ADS] [CrossRef] (In the text)
- Litvinenko, Y. E. 2005, Solar Phys., 229, 203 [NASA ADS] [CrossRef] (In the text)
- Litvinenko, Y. E. 2009, ApJ, 694, 1464 [NASA ADS] [CrossRef] (In the text)
- Litvinenko, Y. E., & Craig, I. J. D. 1999, Solar Phys., 189, 315 [NASA ADS] [CrossRef] (In the text)
- Litvinenko, Y. E., & Craig, I. J. D. 2000, ApJ, 544, 1101 [NASA ADS] [CrossRef] (In the text)
- McKenzie, D. E., & Hudson, H. S. 1999, ApJ, 519, L93 [NASA ADS] [CrossRef] (In the text)
- Priest, E. R., & Forbes, T. 2000, Magnetic reconnection: MHD theory and applications (Cambridge Univ. Press) (In the text)
- Spitzer, L. 1962, Physics of fully ionized gases (John Wiley & Sons) (In the text)
All Figures
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig1.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg84.png) |
Figure 1:
Inflow magnetic field and flow speed against z for the parameters
|
| Open with DEXTER | |
| In the text | |
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig2.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg94.png) |
Figure 2:
Scaling of resistive and viscous dissipation rates over the resistive range
|
| Open with DEXTER | |
| In the text | |
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{1784fig3.eps}
\end{figure}](/articles/aa/full_html/2009/26/aa11784-09/Timg128.png) |
Figure 3:
Current sheet thickness based on measured position of the peak field (circles) against location (solid line with crosses) estimated using formula (18). Results are computed for
|
| Open with DEXTER | |
| In the text | |
Copyright ESO 2009
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.



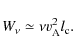
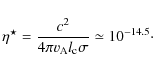
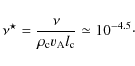
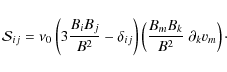

![\begin{displaymath}\eta X'' + \alpha \sin(\omega z) X' + {\alpha \omega \over 2}[
\cos(\omega z) X + \sin(\omega z) Y] = 0,
\end{displaymath}](/articles/aa/full_html/2009/26/aa11784-09/img76.png)
![\begin{displaymath}\eta Y'' + \alpha \sin(\omega z) Y' + {\alpha \omega \over 2}[
\cos(\omega z) Y - \sin(\omega z) X] = 0.
\end{displaymath}](/articles/aa/full_html/2009/26/aa11784-09/img77.png)