A&A 411, L473-L476 (2003)
DOI: 10.1051/0004-6361:20031299

A new model for brown dwarf spectra including accurate unified line shape theory for the Na I and K I resonance line profiles

N. F. Allard1,2 - F. Allard3 - P. H. Hauschildt4 - J. F. Kielkopf5 - L. Machin1,3


1 - Institut d'Astrophysique de Paris, CNRS, 98bis boulevard Arago, 75014 Paris, France
2 - Observatoire de Paris-Meudon, LERMA, 92195 Meudon Principal Cedex, France
3 - Centre de Recherche Astronomique de Lyon, École Normale Supérieure, 69364 Lyon Cedex 07, France
4 - Hamburger Sternwarte, Gojenbergsweg 112, 21029 Hamburg, Germany
5 - Department of Physics, University of Louisville, Louisville, KY 40292, USA

Received 28 July 2003 / Accepted 26 August 2003

Abstract
We present the first brown dwarf atmosphere models based on theoretical calculations of absorption profiles of sodium and potassium perturbed by helium and molecular hydrogen. The synthetic spectra have been compared to previous calculations with Lorentz profiles and the classic van der Waals approximation, and to the observed spectrum of the T dwarf SDSS 1624. The new profiles provide increased opacities in the optical spectra of methane brown dwarfs. However, the potassium and sodium far wings alone cannot explain the missing opacity in the 0.85 to 1.1 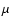$\mu$m range.

Key words: brown dwarfs - line: profiles - radiation mechanisms: general - stars: atmospheres

1 Introduction

Evolved methane brown dwarfs are hydrogen-rich substellar mass objects which show methane bands in their spectra. Their atmosphere is depleted of refractory elements due to grain sedimentation. Resonance lines of alkali elements can form in deep regions of the atmosphere, and are the rare remaining sensitive spectral type and atmospheric parameter indicators. The importance of the far wings of the potassium doublet, centered at 0.77 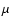$\mu$m in spectra of methane brown dwarfs, has been demonstrated by Burrows et al. (2000). With these wings defining a pseudo-continuum out to more than 2500 Å from the line center, it became clear that the classical approximations that were widely used in main sequence star and brown dwarf model atmospheres (see e.g. Allard et al. 2001) - Lorentz profiles using van der Waals damping constants, such as described by Schweitzer et al. (1996) for example - were not appropriate to handle this situation. This motivated Burrows et al. (2002) to modify Lorentz profiles, introducing cutoffs and parameters.

Fortunately, the broadening of alkali lines by rare gases is a problem which has been extensively investigated in experimental and theoretical work (Allard & Kielkopf 1982). Interatomic interactions are the main physical quantities needed for a good understanding of collisional processes. The theoretical potentials for the binary interactions of alkali atoms perturbed by He or H2 were computed for the lower states to an accuracy suitable for line shape calculations by Pascale (1983, 2003) and Rossi & Pascale (1985). This allows us to solve the radiative collision problem given an appropriate theoretical framework for the line shape. In a recent paper, Burrows & Volobuyev (2003, hereafter BV), using their evaluations of multiconfiguration self-consistent field Hartree-Fock potentials, have calculated the far wing line profiles of the 0.589 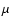$\mu$m sodium D and 0.77 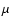$\mu$m potassium doublets using the uniform approximation to the wing profile (Szudy & Baylis 1975; 1996). This is a major improvement compared to the unrealistic use of a Lorentzian so far in the wings. Also, in contrast to a simple quasistatic model, this formula has no singularity and should in principle predict the satellite shapes. However, profiles in the satellite regions were poorly determined by BV, and no applications to brown dwarfs model atmospheres and synthetic spectra have been published to this day.

In this paper, we present the first application of accurate alkali profiles of sodium and potassium doublets to the model atmospheres and synthetic spectra of brown dwarfs, and we compare the results and the depth of satellite bands to the observed spectrum of the T dwarf SDSS 1624.

2 Theoretical line profiles

A theory of spectral line broadening has been developed to calculate neutral atom spectra given the interaction and transition moments for relevant states of the radiating atom with other atoms in its environment. Within this framework it is possible to compute the complete spectrum with a unified approach. Unlike impact theories of line broadening which predict a lorentzian line, the classical static theories that may apply in regions of the near wing, or the approximation methods of Szudy & Baylis (1975, 1996) used by BV, our unified theory provides an accurate spectrum from the line center to the extreme wing. Complete details and the derivation of the theory are given by Allard et al. (1999). Our approach is based on quantum theory of spectral line shapes by Baranger (1958a,b) with an adiabatic representation to include the degeneracy of atomic levels.


  \begin{figure} \par {\resizebox{8.8cm}{!}{ \includegraphics[clip]{letter_allard_fig1.eps}} }\end{figure} Figure 1: Variation of the Na and K line profiles perturbed by He with density $n_{{\rm He}} =10^{19}$ cm-3. The temperatures (top to bottom) are 3000, 2000, 1000, and 500 K.
Open with DEXTER

The spectrum can be written as the Fourier transform of the autocorrelation function of the dipole moment in the Heisenberg representation (Allard & Kielkopf 1982)

\begin{displaymath}F_\nu(\Delta \nu) = {\bf FT} \left[\exp\left(ng(s)\right)\right]. \end{displaymath} (1)

For a transition 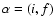$\alpha =(i,f)$ from initial state i to final state f, we have
                                            $\displaystyle g_{\alpha}(s)$ = $\displaystyle \frac{1} {\sum_{e,e'} ~ \! ^{(\alpha)} ~ \vert d_{ee'}\vert^2 } \sum_{e,e'} ~ \! ^{(\alpha)} \; \;$  
    $\displaystyle \times \int^{+\infty}_{0}\!\!2\pi\rho {\rm d}\rho \int^{+\infty}_{-\infty}\!\! {\rm d}x \; \tilde{d}_{ee'}[ ~ R(0) ~ ] ~$  
    $\displaystyle \times \left[ ~ {\rm e}^{\frac{i}{\hbar}\int^s_0 ~ {\rm d}t \; V_... ...ilde{d^{*}}_{ee'}[ ~ R(s) ~ ] ~ - ~ \tilde{d}_{ee'}[ ~ R(0) ~ ] \right] \; . \;$ (2)

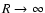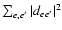The e and e' label the energy surfaces on which the interacting atoms approach the initial and final atomic states of the transition as  $R \rightarrow \infty$ (here R denotes the internuclear distance between the radiator and the perturber). The total line strength of the transition is  $\sum_{e,e'} \vert d_{ee'}\vert^2$. The radiative dipole transition moment of each component of the line depends on R, and changes during the collision. At time t from the point of closest approach for a rectilinear classical path

\begin{displaymath}R(t) = \left[\rho^2 + (x + vt)^2 \right]^{1/2} \; , \; \end{displaymath} (3)

where $\rho$ is the impact parameter of the perturber trajectory, and x is the position of the perturber along its trajectory. We define $\tilde{d}_{ee'}(R(t))$ as a modulated dipole (Allard et al. 1999)

\begin{displaymath}\tilde{d}_{ee'}[R(t)] = d_{ee'}[R(t)]{\rm e}^{-\frac{\beta}{2}V_{e}[R(t)] } \; , \; \end{displaymath} (4)

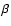where $\beta $ is the inverse temperature (1/kT).

The alkali line profiles and satellites are calculated for physical conditions encountered in the atmospheres of brown dwarfs. Although our theory takes into account the effects of multiple close collisions, for use here we computed the line profile in the low density limit as described by Allard et al. (1994). This method uses the expansion of the autocorrelation function in powers of density. We assume that interactions of an absorbing atom with more than one perturber do not contribute to the far line wing profile under conditions in brown dwarf atmospheres.

3 Interpretation of the absorption profiles

We used the molecular potentials and dipole moments of Pascale (1983) for the alkalis perturbed by He. The shape of the line wing is sensitive to the difference between the ground and excited state interaction potentials, and satellites appear corresponding to extrema in 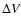$\Delta V$. The difference potential energy surfaces as shown in Figs. 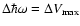2a and 2b give rise to satellites in the far wings positioned at $\Delta \hbar\omega = \Delta V_{\rm max}$ (Allard 1978). Another important factor is the variation of the radiative dipole moment during the collision once modulated by the Boltzmann factor e -Ve (r)/2kT (Figs. 2a and 2b).

The maxima in the difference potentials 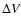$\Delta V$ lead to far blue satellites. Their presence is very sensitive to the temperature due to the fast variation of the modulated dipole moment with temperature in the internuclear region where the line satellite is formed (Figs. 2a and 2b). We present in Fig. 1 the absorption cross section for the resonance lines of Na and K for a He density of 1019 cm-3 and temperatures from 500 to 3000 K. The strength of the satellites increases with temperature. For Na, the satellite due to interactions with He is at 0.52 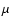$\mu$m with oscillatory structure between the satellite and the line center. For K, the He satellite is at 0.69 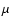$\mu$m. At lower temperatures the satellites are manifested as a distinct break in the line wing continuum, but at 1000 K and above, there is a broad line-like maximum, often referred to as a "satellite band.''

The alkali-H2 molecular potentials have been calculated by Rossi & Pascale (1985), but only for the C2v and C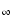$_\infty $symmetries. We also had to assume that the dipole moment for the transitions is constant and equal to its asymptotic value, but modulated by the Boltzmann factor to take into account temperature effects.

We note the presence of far blue satellites of Na-H2 between 0.49 and 0.508 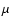$\mu$m shown in Fig. 3a. The satellites arising from potentials with C2v symmetry are more sharply defined and intense at a given temperature, and closer to the resonance line center. In Fig. 3b, the blue satellites of K-H2 appear from 0.67 to 0.69 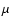$\mu$m. These satellite features increase in strength with temperature over the range of our calculations from 500 to 3000 K. An extended red wing with no satellite structure also increases with temperature. This is due to contributions from the difference potentials which do not present extrema.

  \begin{figure} \par\mbox{\subfigure[K-He]{\resizebox{8cm}{!}{\includegraphics[cl... ...izebox{8cm}{!}{\includegraphics[clip]{letter_allard_fig2b.eps}} }}\end{figure} Figure 2: Variation with temperatures of the K-He and K-H2modulated dipole at 3000, 2000, 1000, and 500 K (top to bottom), and the difference of upper and lower state potentials for the resonance lines. 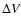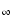For K-H2, difference potentials $\Delta V$ are shown for the C2v (--) and C$_\infty $ (- - -) symmetries.
Open with DEXTER


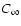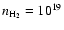   \begin{figure} \par\mbox{\subfigure[Na-H$_2$\space profiles.]{\resizebox{8cm}{!}... ...zebox{8cm}{!}{ \includegraphics[clip]{letter_allard_fig3b.eps}} }}\end{figure} Figure 3: Variation with temperature of the Na and K line profiles perturbed by H2 for the C2v (--) and  $C_{\infty }$ (- - -) symmetries. The density of perturbers is  $n_{{\rm H}_2} =10^{19}$ cm-3. The temperatures (top to bottom) are 3000, 2000, 1000, and 500 K.
Open with DEXTER

4 Application to brown dwarf synthetic spectra


  \begin{figure} \par {\resizebox{8.8cm}{!}{ \includegraphics[clip]{letter_allard_fig4.eps}} }\end{figure} Figure 4: Synthetic spectra obtained with the van der Waals approximation (dot-dashed line), and with the detailed profiles of Fig. 3 (dotted line) are compared for an effective temperature of 1000 K, surface gravity of $\log g = 5.5$, and solar composition. The observed spectrum of SDSS 1624 (Strauss et al. 1999, full line) is also shown. The synthetic spectra have been degraded to a 10 Å resolution thoughout, and the models are converged.
Open with DEXTER

We have computed model atmospheres and synthetic spectra using the Allard et al. (2001) atmosphere program PHOENIX and the new theoretical Na I D and K I profiles. The results are compared to previous models in Fig. 4, and demonstrate that these improvements are of fundamental importance for obtaining a better quantitative interpretation of the spectra. The new profiles carry significantly more opacity within the first 1800 Å from the line centers, while providing less opacity further in the red wings. The optical-to-red pseudo-continuum is therefore depressed, while raised at the flux maximum near 1.1 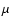$\mu$m, compared to models based upon the van der Waals approximation. Figure 4 also shows the observed spectrum of the T6 methane brown dwarf SDSS 1624. The changes provided by the new profiles correspond more closely to the strongly depressed red spectrum of the T dwarf.

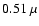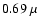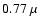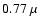BV stated that satellites would provide only negligible additional opacity for these two transitions. Indeed, these features, located around $0.51~\mu$m and $0.69~\mu$m for the Na I D and  K I $0.77~\mu$m doublets respectively, occur in a regime of low emitted flux for such cool brown dwarfs, and do not affect significantly the balance of energy and the thermal profile. However, the K I $0.77~\mu$m satellite forms a broad but weak feature in the vicinity of the Li I transition at 6708 Å, which could be observable with a resolution better than 10 Å and low noise. 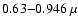This is not the case of the Keck LRIS $0.63{-}0.946~\mu$m spectra of SDSS1624 shown in Fig. 4. We have therefore removed the observed spectrum in Fig. 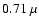4 for wavelengths below $0.71~\mu$m for clarity.

However we find that the far wings of the potassium and sodium doublets cannot alone explain the missing opacities between 0.85 and 1.1 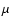$\mu$m. Perhaps allowing for contributions of other alkalis such as Rb I and Cs I can resolve this problem. For the systems Rb-He and Cs-He the line satellites are much closer to the line core, and their effect is important in the near wing. However, the relative abundances of these elements are small, and their effect may be negligible.

Another important point is the use of the low-density limit for the wing. We have restricted our expansion of the autocorrelation function to the first order, assuming that interactions with more than one perturber do not contribute to the profile. This is acceptable if the density of perturbers at the depth of formation of the wings is less than 1019 cm-3 for Na I and K I,but it is not correct for higher densities, or when the line satellites of the alkali-perturber system are closer to the main line (Allard et al. 2003). This cannot be taken into account in the uniform approximation of the Frank-Condon theory, and will be included in future work.

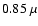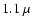In conclusion we have presented the first application of detailed alkali profiles in the construction of synthetic spectra for brown dwarf atmospheres. While the results are encouraging and account for the observed opacity in the visible spectrum, the source of additional opacity in the $0.85~\mu$m to $1.1~\mu$m region remains a mystery.

Acknowledgements
We are grateful to J. Pascale for kindly providing the molecular potentials and dipole moments, and to S. K. Leggett for providing an up-to-date observed spectrum of the T6 brown dwarf SDSS 1624.

References


Copyright ESO 2003