A&A 386, 187-203 (2002)
DOI: 10.1051/0004-6361:20020183
S. Meibom 1,2 - J. Andersen 1 - B. Nordström 1,3
1 - Astronomical Observatory, Niels Bohr Institute for Astronomy,
Physics, and Geophysics,
Juliane Maries Vej 30, 2100 Copenhagen, Denmark
2 -
Astronomy Department, University of Wisconsin, Madison, WI 53706, USA
3 -
Lund Observatory, PO Box 43, 221 00 Lund, Sweden
Received 28 November 2001 / Accepted 30 January 2002
Abstract
We present multiple-epoch radial-velocity observations for 104 stars
in a
![]() field of the intermediate-age open
cluster IC4651 to
field of the intermediate-age open
cluster IC4651 to ![]() .
Only 13 stars (13%) of the full sample
are field stars. From the 44 single member stars we find a mean radial
velocity of
.
Only 13 stars (13%) of the full sample
are field stars. From the 44 single member stars we find a mean radial
velocity of
![]() ,
and
the 12 single red-giant members yield a true radial-velocity dispersion
of
,
and
the 12 single red-giant members yield a true radial-velocity dispersion
of
![]() .
Of the 19 giant members, 7 (37%) are
spectroscopic binaries with periods up to 5000 days, while 35 (52%) of
the 67 main-sequence and turnoff members are binaries with periods less
than
.
Of the 19 giant members, 7 (37%) are
spectroscopic binaries with periods up to 5000 days, while 35 (52%) of
the 67 main-sequence and turnoff members are binaries with periods less
than ![]() 1000 days. Combined with our deep, accurate CCD Strömgren
photometry in a
1000 days. Combined with our deep, accurate CCD Strömgren
photometry in a
![]() field of IC 4651
(Meibom 2000), these data substantially improve the definition of the
cluster locus in the colour-magnitude diagram and the spatial structure
of the cluster, although the photometry shows that IC 4651 contains at
least twice as many stars on the upper main sequence as was believed when
the radial-velocity survey was initiated.
The single cluster members define a very tight sequence in the CMD, and two
sets of isochrones from stellar models with convective overshooting (
field of IC 4651
(Meibom 2000), these data substantially improve the definition of the
cluster locus in the colour-magnitude diagram and the spatial structure
of the cluster, although the photometry shows that IC 4651 contains at
least twice as many stars on the upper main sequence as was believed when
the radial-velocity survey was initiated.
The single cluster members define a very tight sequence in the CMD, and two
sets of isochrones from stellar models with convective overshooting (
![]() = 0.2) have been fit to it. Our best estimate for the age of IC 4651 is
= 0.2) have been fit to it. Our best estimate for the age of IC 4651 is
![]() Gyr, assuming
Gyr, assuming
![]() (Hyades) and
E(b-y) = 0.071.
Including the
(Hyades) and
E(b-y) = 0.071.
Including the ![]() 650 stars newly discovered from the photometry,
we estimate the present total mass of IC4651 to be
650 stars newly discovered from the photometry,
we estimate the present total mass of IC4651 to be
![]() ,
excluding any undetected stellar remnants. The corresponding tidal cutoff
radius is
,
excluding any undetected stellar remnants. The corresponding tidal cutoff
radius is
![]() .
IC4651 shows evidence of moderate mass
segregation: Most of the turn-off stars and nearly all the red giants are
located at radii smaller than
.
IC4651 shows evidence of moderate mass
segregation: Most of the turn-off stars and nearly all the red giants are
located at radii smaller than
![]() ,
while the lower main-sequence
stars are less centrally concentrated. The spatial distributions of cluster
and field stars indicate that additional cluster stars are probably still
to be found outside the fields studied so far.
Comparison of the present mass function of IC 4651 with plausible initial
mass functions indicates that the cluster initially contained at least
,
while the lower main-sequence
stars are less centrally concentrated. The spatial distributions of cluster
and field stars indicate that additional cluster stars are probably still
to be found outside the fields studied so far.
Comparison of the present mass function of IC 4651 with plausible initial
mass functions indicates that the cluster initially contained at least
![]() 8300 stars with a total mass of
8300 stars with a total mass of
![]() .
Thus, of the
original cluster stars only
.
Thus, of the
original cluster stars only ![]() 7%, containing
7%, containing ![]() 12% of the initial
mass, remain today. Of the initial cluster mass,
12% of the initial
mass, remain today. Of the initial cluster mass, ![]() 35% has been lost due
to evolution of the most massive stars into white dwarfs or other remnants
while the remaining
35% has been lost due
to evolution of the most massive stars into white dwarfs or other remnants
while the remaining ![]() 53%, comprising
53%, comprising ![]() 93% of the original
low-mass stars, appear to have migrated out of the observed field or been
lost from the cluster altogether. IC4651 is currently 1 kpc closer to the
Galactic center than its "sister'' cluster NGC3680 (Nordström et al. 1997),
but their Galactic orbital parameters indicate that the mean orbital radius
of IC 4651 is in fact larger by 0.7 kpc, providing a plausible reason why
it is much less advanced in its dynamical evolution than the coeval cluster
NGC3680.
93% of the original
low-mass stars, appear to have migrated out of the observed field or been
lost from the cluster altogether. IC4651 is currently 1 kpc closer to the
Galactic center than its "sister'' cluster NGC3680 (Nordström et al. 1997),
but their Galactic orbital parameters indicate that the mean orbital radius
of IC 4651 is in fact larger by 0.7 kpc, providing a plausible reason why
it is much less advanced in its dynamical evolution than the coeval cluster
NGC3680.
Key words: open clusters and associations: IC 4651 - stars: HR diagram - stars: evolution - stars: kinematics - stars: fundamental parameters
Open star clusters are a rich source of information on several key topics in stellar and galactic astrophysics. First, stars seem to form primarily in clusters. Second, as the stars evolve, the cluster sequence in the colour-magnitude diagram (CMD) traces the form of progressively older isochrones in considerable detail, and thus allows to check the predictions of stellar evolution models. Third, rich clusters can be observed to large distances from the Sun and, therefore, serve as probes of the age and abundance structure of the galactic disk. And fourth, as clusters evolve through dynamical interactions, internally and with the Galactic tidal field, their structure also changes and the cluster stars are gradually lost to the field. The many uses of (especially older) open clusters in stellar and Galactic astrophysics were comprehensively reviewed by Friel (1995) and are illustrated, e.g., by Friel & Janes (1993) and Janes & Phelps (1994).
The key observational tool in the study of a star cluster is an accurate CMD in a well-calibrated colour system. However, any determination of distance, reddening, chemical composition, or age, and any detailed isochrone fit, assumes that one can distinguish cluster members from field stars and identify binary and multiple stars among the cluster members. Further, in studies of the dynamical evolution of clusters one also needs information on the velocities and masses of the individual (single or binary) cluster members. Detailed membership information can be provided by proper motion and radial velocity data, the latter also allowing to detect spectroscopic binaries if multiple observations are obtained over long intervals.
The depth of analysis that can be achieved with complete, high-quality
photometric and kinematic data is perhaps best illustrated in the classic
study of the Hyades by Perryman et al. (1998), while the observational history of
NGC 3680 demonstrates the difficulty in reaching firm conclusions
without such information (Nordström et al. 1997). Yet, besides these two
clusters, the Pleiades (Raboud & Mermilliod 1998a), Praesepe (Raboud & Mermilliod 1998b),
NGC 752 (Daniel et al. 1994), and M67 (Nissen et al. 1987; Mathieu et al. 1986) are still
the only clusters to have reasonably complete kinematic data. The present
paper adds the intermediate-age cluster IC 4651 (age ![]() 1.7 Gyr)
to that select company.
1.7 Gyr)
to that select company.
Our study of IC 4651 and its "sister'' cluster NGC 3680 (Nordström et al. 1997), of nearly identical chemical composition and age, was initiated in 1988 to provide tighter observational constraints on stellar models and on ages derived from them. Indeed, previously published ages for the relatively nearby IC 4651 ranged from 1.3 Gyr (Mazzei & Pigatto 1988) to 4.0 Gyr (Maeder 1990), even when based on the same photometry. Moreover, both clusters were used as evidence both for and against models with extended convective cores ("overshooting'' models). Since then, evidence from NGC 3680 (see Nordström et al. 1997) and many other sources has proved the reality of "overshooting'' beyond doubt; the debate now concerns its physical nature and quantitative description.
However, our study of NGC 3680 not only ruled out the use of standard models
for stars above
![]() ,
it also showed NGC 3680 to be
an extreme case of dynamical evolution: Virtually all cluster stars more than
two magnitudes below the turnoff have been lost from the area studied so far,
and of the remainder some 60% are spectroscopic binaries. Clearly, neither
stellar evolution nor, especially, Initial Mass Functions (IMF's) can be
studied in open clusters without explicit consideration of their dynamical
evolution, as emphasized also in recent theoretical studies (de la Fuente Marcos 1997; Portegies Zwart et al. 2001, and references therein).
,
it also showed NGC 3680 to be
an extreme case of dynamical evolution: Virtually all cluster stars more than
two magnitudes below the turnoff have been lost from the area studied so far,
and of the remainder some 60% are spectroscopic binaries. Clearly, neither
stellar evolution nor, especially, Initial Mass Functions (IMF's) can be
studied in open clusters without explicit consideration of their dynamical
evolution, as emphasized also in recent theoretical studies (de la Fuente Marcos 1997; Portegies Zwart et al. 2001, and references therein).
The present study of IC 4651 underscores this point again, based on the
recent, accurate Strömgren uvby photometry for over 17000 stars in
a
![]() field around IC 4651 by Meibom (2000);
references to previous work on the cluster can be found in that paper.
Meibom (2000) succeeded in delineating the main sequence down to six magnitudes below the turnoff; even more importantly, it was shown that the
cluster covers at least twice the area and contains twice as many stars
as previously believed. Thus, the radial-velocity survey presented in the
present paper turns out in fact to only cover the central, classical
cluster area, but it remains adequate to address the fundamental dynamical
properties of IC 4651. We note that a radial-velocity study of the red
giant stars in IC 4651 (and NGC 3680) was published already by
Mermilliod et al. (1995), but these stars are included again here, because
additional observations and an improved zero-point calibration have become
available after that time.
field around IC 4651 by Meibom (2000);
references to previous work on the cluster can be found in that paper.
Meibom (2000) succeeded in delineating the main sequence down to six magnitudes below the turnoff; even more importantly, it was shown that the
cluster covers at least twice the area and contains twice as many stars
as previously believed. Thus, the radial-velocity survey presented in the
present paper turns out in fact to only cover the central, classical
cluster area, but it remains adequate to address the fundamental dynamical
properties of IC 4651. We note that a radial-velocity study of the red
giant stars in IC 4651 (and NGC 3680) was published already by
Mermilliod et al. (1995), but these stars are included again here, because
additional observations and an improved zero-point calibration have become
available after that time.
In the following, we describe the new observational data for IC 4651 in Sect. 2 and analyze them in Sect. 3 in terms of cluster membership, binary star detection, and field star contamination. Section 4 is devoted to a comparison of theoretical isochrones with the cluster sequence, while Sect. 5 discusses the current structure and dynamical state of the cluster and compares with theoretical computations. Finally, Sect. 6 summarizes our findings and discusses the outlook for further studies.
The new CMD for IC 4651 by Meibom (2000), based on CCD uvby
photometry for 17640 stars to
![]() in a
in a
![]() field supersedes all previous photometry
for the main-sequence region of the cluster. All details of the
observations and reduction procedures are given in the paper,
which also summarizes the observational history of IC 4651.
Typical standard errors of the mean magnitudes are
field supersedes all previous photometry
for the main-sequence region of the cluster. All details of the
observations and reduction procedures are given in the paper,
which also summarizes the observational history of IC 4651.
Typical standard errors of the mean magnitudes are
![]() in V,
in V,
![]() in b,
in b,
![]() in v, and
in v, and
![]() in u.
in u.
The salient findings from the photometry were: (i): a greatly superior definition of the main sequence in the (v-y) - V CMD, as compared to the traditional combination (b-y) - V; (ii): an effective doubling of the known size of the cluster in terms of both area and number of stars; and (iii): a clear tendency for the faint main-sequence stars to prefer the outskirts of the cluster. All three topics are further discussed below.
While the Meibom (2000) paper was in press, a new CMD comprising
uvby-
![]() photometry of 387 stars in a
photometry of 387 stars in a
![]() field to
field to
![]() was published by
Anthony-Twarog & Twarog (2000). Comparison with our data shows a similar colour
discrepancy for the red giants (
was published by
Anthony-Twarog & Twarog (2000). Comparison with our data shows a similar colour
discrepancy for the red giants (
![]() ,
(b-y) > 0.6) as found by Meibom (2000, Fig. 2) relative to the earlier
data by Anthony-Twarog Twarog (1987), our photometry being about 0.03 mag
brighter in V and bluer in b-y than theirs. This is readily
understandable because our observations were calibrated primarily
for the main-sequence region; also, the uvby colour calibrations
are less reliable for red giants, both theoretically and observationally.
For the 252 stars in the colour range covered by our standard stars,
i.e.,
(b-y) < 0.6, the average differences are
,
(b-y) > 0.6) as found by Meibom (2000, Fig. 2) relative to the earlier
data by Anthony-Twarog Twarog (1987), our photometry being about 0.03 mag
brighter in V and bluer in b-y than theirs. This is readily
understandable because our observations were calibrated primarily
for the main-sequence region; also, the uvby colour calibrations
are less reliable for red giants, both theoretically and observationally.
For the 252 stars in the colour range covered by our standard stars,
i.e.,
(b-y) < 0.6, the average differences are
![]() (s.d.) in V and
(s.d.) in V and
![]() (s.d.) in (b-y), respectively,
a quite satisfactory result.
(s.d.) in (b-y), respectively,
a quite satisfactory result.
Given the close agreement between the photometry by Anthony-Twarog & Twarog (2000) and Meibom (2000), but also the larger area and greater depth of the latter, we will use the Meibom photometry in the remainder of this paper.
In order to distinguish between field and cluster stars and detect the
spectroscopic binaries in the cluster, radial-velocity observations were
made during the years 1989-1997 with the photoelectric scanner CORAVEL
(Mayor 1985) on the Danish 1.54-m telescope at ESO, La Silla. The
observations are referred to the accurate velocity zero-point determined
from a large number of observations of minor planets and standard stars by
Udry et al. (1999). The observing list comprised all known red giants in
and near the cluster, plus all candidate main-sequence and turnoff stars
brighter than the limiting magnitude of CORAVEL (![]() )
from the then
largest known photometric surveys of the cluster, by Eggen (1971) and
Anthony-Twarog et al. (1988, AMCT).
)
from the then
largest known photometric surveys of the cluster, by Eggen (1971) and
Anthony-Twarog et al. (1988, AMCT).
With the aim to obtain three or more observations per star in IC4651, a total of 520 observations were made of the 117 programme stars, including about 220 measurements of the 20 red giants and 300 measurements of 97 turnoff and main-sequence stars. Many of the stars in the latter group rotate rapidly, so high signal levels were required and typical integration times were of the order of an hour; for the red giants integration times were 5-10 min. Only 13 stars, mainly in the turn-off region, rotated too fast for a proper determination of their radial velocity; in the system of Meibom (2000), these stars are MEI5489, 7386, 7628, 10059, 10232, 11035, 11305, 11852, 11888, 12035, 12089, 13015, and 14069.
If a first observation of a photometric non-member gave a velocity far from the cluster mean, or a second observation immediately established a star as an obvious spectroscopic binary, observations were normally stopped. Otherwise, three or more observations were obtained over a time interval of typically 1000-2000 days for the main-sequence stars, and 5000 days for the red giants (observed since 1983). The red giant data were analyzed by Mermilliod et al. (1995), but are rediscussed here because additional observations have been obtained.
The results of the radial-velocity observations are summarized in Table 1. Star numbers are given in the systems of Meibom (2000, MEI), Eggen (1971, E, numbers less than 200)
and Anthony-Twarog et al. (1988, AT, numbers larger than 1000). A few outlying red cluster
giants have numbers from either Lindoff (1972, L, Nos. 241 and 244) or Mermilliod et al. (1995)
(M, Nos. 811, 812, and 817).
Following the mean velocity <RV> and its error,
![]() ,
n is the
number of observations and
,
n is the
number of observations and ![]() the standard deviation of a single
observation; for double- or triple-lined binaries, we give the
systemic velocity and
the standard deviation of a single
observation; for double- or triple-lined binaries, we give the
systemic velocity and
![]() as computed with the method of
Wilson (1941). O/C is the
ratio
as computed with the method of
Wilson (1941). O/C is the
ratio ![]() /
/
![]() ,
where
,
where
![]() is the average value of the
error estimated for each observation of the star from the number of counts
and the shape of the profile. The algorithm for computing
is the average value of the
error estimated for each observation of the star from the number of counts
and the shape of the profile. The algorithm for computing
![]() has been
improved since the study of NGC 3680 by Nordström et al. 1996, and no
dependence of O/C on stellar rotation is seen in IC4651.
Consequently, no corrections have been applied to the present data.
If
has been
improved since the study of NGC 3680 by Nordström et al. 1996, and no
dependence of O/C on stellar rotation is seen in IC4651.
Consequently, no corrections have been applied to the present data.
If
![]() ,
,
![]() =
=
![]() is given as a more
realistic error estimate than
is given as a more
realistic error estimate than
![]() .
Finally,
.
Finally, ![]() is the time span (days) covered by the observations;
the median value of
is the time span (days) covered by the observations;
the median value of ![]() is 1845 days.
is 1845 days.
In order to facilitate future studies of the velocity variables, the
individual radial velocity observations of all stars are provided in Table A1 (in electronic form only).
Figure 1 shows the CMD of IC 4651, with the stars
with radial-velocity data identified by squares. The small points indicate
stars not included in the radial-velocity survey, either because they were
too faint or because they lie outside the inner field believed to
contain the cluster when the radial-velocity survey was conducted.
The many new outlying and/or faint cluster members would form ideal targets
for a new survey with a multi-fiber spectrometer on a large telescope.
The distribution of the observed mean radial velocities is shown in Fig. 2a; as seen, the velocities of the (relatively few)
field stars are distributed in the range -80 to +40 km s-1.
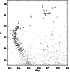 |
Figure 1: The (b-y, V) CMD of IC 4651 (Meibom 2000). Stars with radial-velocity data are framed with squares. |
| Open with DEXTER | |
 |
Figure 2:
Radial-velocity histograms for a) all observed stars; b) radial velocity members (line) and red giant members only (filled curve).
The vertical lines show the mean cluster velocity and its mean error
|
| Open with DEXTER | |
A few stars require separate comment. First, by mistake, Table 2 of Meibom (2000 MEI) identified MEI6726 with both E73 and AT1230; as indicated in our Table 1, E73 is in fact the star MEI7036. Moreover, correction of a previously misidentified observation shows that MEI6333 is in fact a single star, contrary to the assignment of variability by Mermilliod et al. (1995).
More substantially, additional radial velocities have been obtained for most of the red giant stars since Mermilliod et al. (1995), extending the time basis of the data. These new data led to the detection of two new spectroscopic binaries amongst the red giants (MEI11218 and 14527). We have also recomputed the spectroscopic binary orbits given previously by Mermilliod et al. (1995). The resulting orbital elements are given in Table 2 and are significantly improved in accuracy over those by Mermilliod et al. (1995).
For double-lined binaries with insufficient data for an actual orbit
determination, the Wilson (1941) method also provides an estimate of the
mass ratio
![]() .
For the stars labeled "sb2" in Table 1,
we find the following results: MEI6726:
.
For the stars labeled "sb2" in Table 1,
we find the following results: MEI6726:
![]() ;
MEI6733:
;
MEI6733:
![]() ;
MEI8302:
;
MEI8302:
![]() ;
MEI9278:
;
MEI9278:
![]() ;
and MEI10029:
;
and MEI10029:
![]() .
MEI10019 and MEI11504 show double lines,
but only one component varies in velocity; thus, these objects are likely to
be triple systems and no mass ratio can be determined.
.
MEI10019 and MEI11504 show double lines,
but only one component varies in velocity; thus, these objects are likely to
be triple systems and no mass ratio can be determined.
Rotational velocities were determined for the stars in IC 4651 as described
by Benz & Mayor (1981; Benz & Mayor 1984) and are included, with their errors, in Table 1. The mean rotational velocity of the 31 measurable single
main-sequence stars is
![]() kms-1 (s.e.m.), with a
standard deviation of 13.1 kms-1. For comparison, the single
main-sequence stars in NGC3680 have a mean rotational velocity of
kms-1 (s.e.m.), with a
standard deviation of 13.1 kms-1. For comparison, the single
main-sequence stars in NGC3680 have a mean rotational velocity of
![]() kms-1 (s.e.m.) with a standard deviation of
13.3 kms-1, essentially the same as in IC 4651. The modest
sample sizes do not allow further detailed study of, e.g., the orientation
of rotational axes, etc.
kms-1 (s.e.m.) with a standard deviation of
13.3 kms-1, essentially the same as in IC 4651. The modest
sample sizes do not allow further detailed study of, e.g., the orientation
of rotational axes, etc.
| MEI | Other | V | b-y | <RV> |
|
n | O/C | P( |
P(RV) |
|
Rem. | |||
| 5289 | E1 | 12.91 | 0.342 | -29.84 | 1.49 | 3 | 2.26 | 0.88 | 1875 | 0.471 | 0.657 | 36.3 | 5.7 | |
| 5456 | E2 | 13.46 | 0.344 | -38.55 | 2.01 | 3 | 3.49 | 2.21 | 1107 | 0.008 | 0.002 | 26.9 | 2.7 | |
| 5658 | E102 | 12.10 | 0.370 | -36.77 | 10.70 | 4 | 21.41 | 9.78 | 2887 | 0.000 | 0.578 | 34.0 | 3.4 | |
| 5682 | E3 | 12.28 | 0.373 | -29.61 | 2.70 | 6 | 6.62 | 10.22 | 2894 | 0.000 | 0.707 | 9.8 | 1.0 | |
| 5797 | E67 | 12.38 | 0.366 | -16.47 | 15.47 | 5 | 34.60 | 50.77 | 1488 | 0.000 | 0.358 | 7.9 | 1.4 | |
| 5920 | E3 | 12.05 | 0.374 | -28.68 | 0.57 | 6 | 1.40 | 1.10 | 2885 | 0.319 | 0.179 | 29.9 | 3.0 | |
| 5930 | E75 | 12.00 | 0.187 | -32.16 | 2.31 | 1 | 2.31 | 1.00 | 0 | - | 0.607 | 23.2 | 5.6 | pm |
| 6097 | E15 | 13.51 | 0.374 | -30.60 | 0.67 | 4 | 1.33 | 1.57 | 2174 | 0.062 | 0.920 | 10.0 | 1.8 | |
| 6176 | E14 | 13.12 | 0.335 | -31.27 | 1.59 | 3 | 2.75 | 1.02 | 1878 | 0.352 | 0.812 | 34.1 | 5.9 | |
| 6277 | E66 | 12.70 | 0.348 | -30.28 | 0.58 | 5 | 1.29 | 1.37 | 2541 | 0.116 | 0.757 | 17.5 | 2.1 | |
| 6282 | A1225 | 14.11 | 0.394 | -31.28 | 0.39 | 3 | 0.65 | 0.97 | 1397 | 0.405 | 0.727 | 6.4 | 4.3 | |
| 6333 | E6 | 10.38 | 0.779 | -30.89 | 0.08 | 11 | 0.26 | 0.78 | 5156 | 0.800 | 0.868 | 0.0 | 0.0 | M* |
| 6342 | E37 | 12.22 | 0.360 | -35.69 | 2.81 | 3 | 4.87 | 1.76 | 1489 | 0.046 | 0.118 | >50 | - | |
| 6375 | E74 | 12.12 | 0.388 | -30.77 | 0.84 | 6 | 2.07 | 1.60 | 2211 | 0.027 | 0.995 | 32.1 | 3.2 | |
| 6686 | E65 | 10.85 | 0.728 | -30.77 | 0.07 | 21 | 3.51 | 9.91 | 5155 | 0.000 | 0.990 | 1.1 | 0.0 | MO |
| 6725 | A1227 | 13.25 | 0.384 | -33.11 | 18.82 | 2 | 26.61 | 19.39 | 774 | 0.000 | 0.901 | 16.7 | 2.8 | |
| 6726 | A1230 | 13.23 | 0.386 | -30.00 | 0.53 | 4 | 36.70 | 37.69 | 1117 | 0.000 | 0.620 | 6.7 | 3.8 | sb2* |
| 6733 | E35 | 12.50 | 0.337 | -29.68 | 6.23 | 5 | 12.33 | 2.05 | 1890 | 0.000 | 0.866 | 14.7 | 4.7 | sb2* |
| 6752 | E | 13.72 | 0.368 | -32.15 | 1.37 | 3 | 2.37 | 3.34 | 1104 | 0.000 | 0.484 | 7.3 | 2.0 | |
| 6762 | A1215 | 14.13 | 0.655 | -74.80 | 0.74 | 1 | 0.74 | 1.00 | 0 | - | 0.000 | 10.7 | 5.6 | |
| 6770 | E78 | 13.02 | 0.338 | -22.89 | 4.06 | 1 | 4.06 | 1.00 | 0 | - | 0.068 | 27.8 | 17.2 | pm |
| 6905 | A1228 | 12.94 | 0.344 | -28.48 | 1.03 | 5 | 2.30 | 2.30 | 2175 | 0.001 | 0.198 | 15.7 | 2.3 | |
| 7016 | E34 | 13.42 | 0.358 | -29.89 | 0.73 | 4 | 1.21 | 0.84 | 2897 | 0.556 | 0.590 | 25.2 | 2.7 | |
| 7036 | E73 | 14.04 | 0.413 | 20.90 | 57.25 | 2 | 80.96 | 86.33 | 346 | 0.000 | 0.367 | 6.1 | 7.0 | |
| 7111 | E17 | 12.62 | 0.341 | -29.37 | 4.09 | 1 | 4.09 | 1.00 | 0 | - | 0.749 | 10.0 | 12.1 | pm |
| 7181 | A4222 | 13.04 | 0.352 | -13.94 | 1.01 | 1 | 1.01 | 1.00 | 0 | - | 0.000 | 10.1 | 4.1 | |
| 7254 | E16 | 12.38 | 0.357 | -33.10 | 5.40 | 2 | 7.63 | 3.11 | 1091 | 0.002 | 0.675 | 67.8 | 6.8 | |
| 7309 | E61 | 13.12 | 0.360 | -34.50 | 8.15 | 4 | 16.30 | 11.62 | 2192 | 0.000 | 0.651 | 24.0 | 2.4 | |
| 7492 | E38 | 13.57 | 0.421 | -46.19 | 0.66 | 2 | 0.33 | 0.35 | 1483 | 0.723 | 0.000 | 11.6 | 2.4 | |
| 7646 | E12 | 10.32 | 0.661 | -31.18 | 0.13 | 7 | 0.22 | 0.63 | 4873 | 0.896 | 0.595 | 1.1 | 1.4 | M |
| 7753 | A1211 | 13.97 | 0.417 | -32.18 | 1.67 | 2 | 2.36 | 3.50 | 1491 | 0.001 | 0.520 | 10.1 | 1.8 | |
| 7754 | E99 | 12.38 | 0.347 | -31.74 | 0.55 | 5 | 0.91 | 0.75 | 2886 | 0.699 | 0.525 | 28.1 | 2.8 | |
| 7947 | E62 | 13.48 | 0.832 | 9.47 | 0.88 | 1 | 0.88 | 1.00 | 0 | - | 0.000 | 7.3 | 5.4 | |
| 8080 | E77 | 12.08 | 0.380 | -29.56 | 0.92 | 5 | 2.06 | 2.71 | 1846 | 0.000 | 0.483 | 13.8 | 1.0 | |
| 8263 | E70 | 12.06 | 0.392 | -31.24 | 0.73 | 5 | 1.63 | 1.22 | 1848 | 0.206 | 0.766 | 65: | - | |
| 8276 | E5 | 12.81 | 0.329 | -29.84 | 1.14 | 4 | 2.29 | 1.47 | 2594 | 0.099 | 0.616 | 23.9 | 2.4 | |
| 8302 | A1208 | 12.60 | 0.358 | -30.58 | 3.49 | 4 | 9.35 | 9.53 | 1143 | 0.000 | 0.962 | 9.9 | 5.0 | sb2* |
| 8303 | A4106 | 13.71 | 0.375 | -31.09 | 0.66 | 4 | 1.31 | 1.35 | 2181 | 0.141 | 0.835 | 13.6 | 1.6 | |
| 8437 | E79 | 13.55 | 0.360 | -28.94 | 0.65 | 4 | 1.17 | 0.90 | 1510 | 0.502 | 0.249 | 21.8 | 2.3 | |
| 8480 | E39 | 12.31 | 0.373 | -29.59 | 0.73 | 5 | 1.50 | 0.92 | 2545 | 0.504 | 0.469 | 27.6 | 10.7 | |
| 8506 | E63 | 12.60 | 0.345 | -38.66 | 12.40 | 3 | 21.47 | 13.06 | 406 | 0.000 | 0.527 | 22.2 | 3.9 | |
| 8540 | E98 | 10.87 | 0.694 | -30.36 | 0.14 | 7 | 0.30 | 0.83 | 4872 | 0.673 | 0.614 | 1.0 | 0.0 | M |
| 8642 | E33 | 13.57 | 0.379 | -29.67 | 1.26 | 4 | 2.52 | 3.11 | 2898 | 0.000 | 0.569 | 11.6 | 1.4 | |
| 8665 | L244 | 11.11 | 0.526 | -30.53 | 0.16 | 27 | 10.92 | 18.63 | 4472 | 0.000 | 0.773 | 1.6 | 1.5 | MO,sb2 |
| 8803 | E64 | 13.72 | 0.370 | -29.91 | 0.40 | 4 | 0.51 | 0.64 | 1797 | 0.747 | 0.570 | 9.2 | 1.7 | |
| 8878 | A1114 | 13.65 | 0.406 | 6.39 | 2.03 | 2 | 2.88 | 3.07 | 1107 | 0.002 | 0.000 | 7.2 | 3.6 | |
| 9025 | E60 | 10.87 | 0.673 | -30.46 | 0.14 | 8 | 0.25 | 0.65 | 4872 | 0.901 | 0.705 | 1.9 | 1.5 | M |
| 9054 | A1108 | 14.05 | 0.395 | -31.76 | 0.81 | 3 | 1.41 | 2.23 | 1404 | 0.007 | 0.545 | 7.0 | 2.6 | |
| 9115 | E71 | 12.92 | 0.353 | -31.02 | 0.42 | 5 | 0.94 | 1.12 | 2896 | 0.305 | 0.862 | 13.5 | 1.2 | |
| 9122 | E58 | 10.87 | 0.717 | -30.58 | 0.13 | 8 | 0.30 | 0.83 | 4872 | 0.703 | 0.920 | 0.0 | 0.0 | M |
| 9263 | A4107 | 13.91 | 0.385 | -29.54 | 1.22 | 3 | 2.11 | 2.84 | 1127 | 0.000 | 0.518 | 7.7 | 3.4 | |
| 9266 | E97 | 11.62 | 0.772 | 19.33 | 0.49 | 1 | 0.49 | 1.00 | 0 | - | 0.000 | 5.8 | 2.6 | |
| 9278 | E59 | 12.87 | 0.354 | -28.35 | 1.75 | 5 | 20.81 | 21.59 | 1499 | 0.000 | 0.288 | 4.2 | 5.2 | sb2* |
| 9357 | E18 | 11.77 | 0.381 | -31.51 | 1.16 | 6 | 2.85 | 4.04 | 2898 | 0.000 | 0.685 | 16.3 | 1.0 |
| MEI | Other | V | b-y | <RV> |
|
n | O/C | P( |
P(RV) |
|
Rem. | |||
| 9488 | A1104 | 13.53 | 0.640 | 42.59 | 0.59 | 1 | 0.59 | 1.00 | 0 | - | 0.000 | 2.2 | 0.0 | |
| 9532 | E7 | 14.17 | 0.402 | -30.51 | 0.38 | 4 | 0.76 | 1.33 | 1405 | 0.158 | 0.867 | 2.1 | 3.0 | |
| 9536 | E56 | 12.18 | 0.378 | -28.28 | 0.71 | 5 | 0.73 | 0.46 | 2545 | 0.937 | 0.122 | 27.8 | 10.8 | |
| 9640 | E32 | 13.13 | 0.341 | -22.30 | 6.04 | 4 | 12.09 | 14.17 | 1845 | 0.000 | 0.173 | 14.9 | 1.5 | |
| 9745 | E53 | 13.44 | 0.403 | -31.07 | 0.24 | 7 | 0.28 | 0.44 | 2900 | 0.982 | 0.832 | 8.3 | 1.2 | |
| 9791 | E96 | 10.34 | 0.788 | -31.44 | 0.12 | 8 | 0.33 | 0.95 | 4872 | 0.515 | 0.389 | 0.1 | 0.0 | M |
| 9826 | E54 | 12.13 | 0.443 | 9.30 | 0.47 | 4 | 0.94 | 1.66 | 1844 | 0.042 | 0.000 | 3.1 | 2.1 | |
| 10019 | A2202 | 12.68 | 0.375 | -29.15 | 1.25 | 4 | 2.49 | 2.40 | 1494 | 0.000 | 0.398 | 23.8 | 4.5 | sb3* |
| 10029 | E55 | 12.23 | 0.389 | -31.60 | 11.08 | 4 | 16.25 | 14.78 | 2211 | 0.000 | 0.940 | 14.8 | 10.9 | sb2* |
| 10195 | L241 | 10.86 | 0.720 | -30.74 | 0.10 | 22 | 8.15 | 19.91 | 4098 | 0.000 | 0.980 | 4.8 | 0.5 | MO |
| 10387 | E0 | 13.57 | 0.774 | -34.22 | 1.64 | 1 | 1.64 | 1.00 | 0 | - | 0.057 | 5.2 | 9.8 | pm |
| 10393 | E8 | 10.67 | 0.666 | -31.54 | 0.14 | 8 | 0.37 | 0.96 | 4873 | 0.529 | 0.325 | 0.1 | 1.8 | M |
| 10807 | A2105 | 13.95 | 0.429 | -31.38 | 0.86 | 4 | 1.72 | 1.89 | 2181 | 0.015 | 0.712 | 11.3 | 2.0 | |
| 10811 | E81 | 13.31 | 0.352 | -33.45 | 5.08 | 3 | 8.80 | 5.29 | 1923 | 0.000 | 0.610 | 27.8 | 4.4 | |
| 10866 | E28 | 12.19 | 0.388 | -30.94 | 1.61 | 7 | 4.25 | 2.06 | 2248 | 0.000 | 0.934 | 36.2 | 3.6 | |
| 10963 | E95 | 11.95 | 0.514 | -29.93 | 0.30 | 6 | 0.74 | 1.21 | 2887 | 0.206 | 0.573 | 12.0 | 0.9 | |
| 11020 | A3205 | 14.26 | 0.422 | -11.23 | 0.65 | 1 | 0.65 | 1.00 | 0 | - | 0.000 | 2.9 | 5.4 | |
| 11123 | E82 | 13.73 | 0.392 | -43.00 | 0.98 | 1 | 0.98 | 1.00 | 0 | - | 0.000 | 15.4 | 4.3 | |
| 11218 | M812 | 11.06 | 0.676 | -30.36 | 0.37 | 4 | 0.73 | 2.09 | 1815 | 0.004 | 0.643 | 0.5 | 1.8 | M* |
| 11453 | E27 | 10.16 | 0.652 | -29.41 | 0.19 | 7 | 0.51 | 1.39 | 4872 | 0.083 | 0.093 | 2.1 | 1.3 | M |
| 11504 | E94 | 11.63 | 0.394 | -28.02 | 0.61 | 6 | 1.49 | 2.37 | 1489 | 0.000 | 0.080 | 10.3 | 1.8 | sb3* |
| 11507 | E11 | 12.41 | 0.309 | -22.64 | 6.56 | 3 | 11.37 | 2.78 | 2208 | 0.001 | 0.227 | 20.2 | 7.9 | |
| 11662 | A2217 | 14.01 | 0.461 | -28.27 | 0.81 | 1 | 0.81 | 1.00 | 0 | - | 0.132 | 13.1 | 2.9 | pm |
| 11670 | E41 | 13.14 | 0.369 | -34.71 | 5.20 | 4 | 10.39 | 12.28 | 1842 | 0.000 | 0.464 | 11.2 | 1.6 | |
| 11709 | E19 | 12.24 | 0.412 | -31.41 | 0.90 | 5 | 2.01 | 1.58 | 1841 | 0.041 | 0.702 | 32.1 | 3.2 | |
| 11808 | E50 | 12.41 | 0.362 | -32.65 | 4.09 | 4 | 8.18 | 9.53 | 1495 | 0.000 | 0.663 | 13.5 | 1.4 | |
| 11887 | E10 | 11.53 | 0.373 | -28.95 | 2.77 | 4 | 5.53 | 2.46 | 1845 | 0.001 | 0.562 | 31.3 | 7.5 | |
| 12008 | E49 | 13.38 | 0.365 | -26.06 | 2.59 | 4 | 5.18 | 4.95 | 2897 | 0.000 | 0.113 | 14.4 | 3.6 | |
| 12137 | A3213 | 14.08 | 0.455 | -31.00 | 0.46 | 2 | 0.65 | 1.05 | 714 | 0.306 | 0.874 | 3.1 | 6.6 | |
| 12227 | E93 | 8.80 | 1.031 | -29.80 | 0.14 | 7 | 0.37 | 1.16 | 4872 | 0.243 | 0.226 | 1.9 | 1.1 | M |
| 12270 | E26 | 12.99 | 0.355 | -30.97 | 0.40 | 6 | 0.99 | 1.03 | 2893 | 0.385 | 0.888 | 16.8 | 1.7 | |
| 12362 | E48 | 12.77 | 0.749 | -77.60 | 1.82 | 2 | 2.57 | 4.85 | 1830 | 0.000 | 0.000 | 4.0 | 0.0 | |
| 12453 | A2214 | 13.19 | 0.346 | -28.56 | 2.84 | 3 | 4.91 | 7.02 | 1107 | 0.000 | 0.490 | 6.8 | 2.0 | |
| 12854 | E86 | 13.74 | 0.383 | -29.49 | 0.71 | 5 | 1.60 | 1.82 | 2544 | 0.014 | 0.429 | 14.0 | 1.4 | |
| 12889 | A3226 | 14.12 | 0.423 | -33.29 | 0.46 | 2 | 0.18 | 0.27 | 714 | 0.784 | 0.094 | 5.9 | 2.7 | |
| 12935 | E22 | 10.92 | 0.681 | -29.87 | 0.13 | 8 | 0.28 | 0.79 | 4873 | 0.764 | 0.260 | 1.6 | 0.0 | M |
| 13113 | E45 | 14.18 | 0.430 | -29.63 | 0.34 | 3 | 0.55 | 0.92 | 1405 | 0.431 | 0.445 | 4.2 | 4.3 | |
| 13238 | E44 | 12.65 | 0.352 | -31.70 | 0.94 | 6 | 2.30 | 3.08 | 1845 | 0.000 | 0.585 | 9.1 | 1.8 | |
| 13247 | E87 | 13.80 | 0.699 | -14.46 | 0.82 | 1 | 0.82 | 1.00 | 0 | - | 0.000 | 7.6 | 5.7 | |
| 13324 | E85 | 13.65 | 0.388 | -28.34 | 0.45 | 4 | 0.19 | 0.21 | 2213 | 0.988 | 0.109 | 16.2 | 1.3 | |
| 13482 | E88 | 14.40 | 0.438 | -28.31 | 2.53 | 2 | 3.57 | 4.00 | 1523 | 0.000 | 0.400 | 3.6 | 8.9 | |
| 13755 | E25 | 12.66 | 0.366 | -32.15 | 0.42 | 4 | 0.67 | 0.80 | 1841 | 0.590 | 0.354 | 9.1 | 1.8 | |
| 14290 | E91 | 10.58 | 0.652 | -31.37 | 0.07 | 27 | 9.27 | 23.90 | 4470 | 0.000 | 0.436 | 0.2 | 1.0 | MO |
| 14331 | A2230 | 13.76 | 0.408 | -33.57 | 0.72 | 2 | 1.01 | 1.04 | 347 | 0.299 | 0.081 | 10.6 | 2.6 | |
| 14364 | E24 | 12.76 | 0.368 | -31.34 | 7.64 | 4 | 15.29 | 18.51 | 1841 | 0.000 | 0.941 | 12.4 | 1.8 | |
| 14527 | E83 | 10.90 | 0.688 | -31.85 | 0.26 | 7 | 0.68 | 1.83 | 4873 | 0.004 | 0.185 | 2.2 | 1.4 | M* |
| 14560 | E84 | 13.09 | 0.384 | -33.45 | 0.39 | 4 | 0.56 | 0.71 | 2209 | 0.689 | 0.071 | 15.3 | 1.4 | |
| 14641 | E23 | 10.65 | 0.684 | -31.19 | 0.07 | 21 | 1.86 | 5.17 | 5155 | 0.000 | 0.583 | 0.9 | 0.0 | MO |
| - | M811 | 10.72 | - | -31.13 | 0.21 | 4 | 0.42 | 1.25 | 1815 | 0.200 | 0.647 | 0.4 | 1.7 | M |
| - | M817 | 10.68 | - | -31.75 | 0.21 | 4 | 0.42 | 1.20 | 1815 | 0.230 | 0.220 | 1.6 | 0.0 | M |
| Orbital element: | 6686 (L97) | 8665 (L244) | 10195 (L241) | 14641 (L236) | 14290 (L139) |
| P [d] | 5021 | 1318.2 | 75.162 | 335.540 | 2764 |
| 40 | 1.5 | 0.012 | 0.057 | 30 | |
| T [HJD-2440000] | 5798 | 7860.6 | 9031.5 | 8956.76 | 8133 |
| 89 | 2.5 | 1.7 | 0.36 | 79 | |
| e | 0.18 | 0.773 | 0.090 | 0.000 | 0.19 |
| 0.02 | 0.017 | 0.016 | 0.007 | 0.04 | |
| 111.3 | 359.6 | 57.4 | 270.0 | 58.8 | |
| 7.2 | 5.0 | 7.7 | - | 10.8 | |
| -30.78 | -30.53 | -30.74 | -31.37 | -31.19 | |
| 0.07 | .16 | 0.10 | 0.05 | 0.07 | |
| K1 [km s-1] | 4.95 | 20.1 | 11.04 | 15.96 | 2.62 |
| 0.11 | 1.0 | 0.14 | 0.07 | 0.10 | |
| K2 [km s-1] | 17.4 | ||||
| 1.3 | |||||
|
|
336.1 | 231 | 11.37 | 73.66 | 97.6 |
| 7.9 | 14 | 0.15 | 0.34 | 4.0 | |
|
|
200 | ||||
| 16 | |||||
| f(m) [ |
0.0600 | 0.0104 | 0.1415 | 0.0049 | |
| 0.0043 | 0.0004 | 0.0019 | 0.0006 | ||
|
|
0.85 | ||||
| 0.10 | |||||
|
|
0.98 | ||||
| 0.11 | |||||
| 0.33 | 0.67 | 0.45 | 0.23 | 0.30 | |
|
|
21 | 22 | 28 | 27 | 21 |
As discussed in detail in Nordström et al. (1997), comparison of the observed
and expected standard deviations of multiple radial-velocity observations
of a star allows to compute the probability
![]() that the velocity
is constant, i.e. that the star is single.
that the velocity
is constant, i.e. that the star is single.
![]() is given in Table 1 for all programme stars with at least 2 observations.
Consistent with other studies using CORAVEL data, we adopt the conservative
variability/duplicity criterion
is given in Table 1 for all programme stars with at least 2 observations.
Consistent with other studies using CORAVEL data, we adopt the conservative
variability/duplicity criterion
![]() to distinguish the
certain binaries from the probable single stars in
Table 1. A few binaries were identified by a double correlation
peak in just a single observation. Our detection efficiency
decreases rapidly for binaries with periods longer than our typical
to distinguish the
certain binaries from the probable single stars in
Table 1. A few binaries were identified by a double correlation
peak in just a single observation. Our detection efficiency
decreases rapidly for binaries with periods longer than our typical
![]() (
(![]() 2000 days) and for small velocity amplitudes, so undetected
binaries certainly remain in IC 4651.
2000 days) and for small velocity amplitudes, so undetected
binaries certainly remain in IC 4651.
Of the 104 observed stars, 45 (43%) are found to be binaries, but the fractions are very different for cluster and field stars (42/86 or 49%, vs. 3/13 or 23%). This is readily understandable because field stars were not monitored systematically once their non-member status had become clear. In contrast, the lower binary frequency among the cluster giants (7/19 or 37%) than among the main-sequence stars (35/67 or 52%) is probably real, as the red giants have been monitored for longer periods and with better precision than the hotter, faster-rotating F-type main-sequence stars. Destruction of the closest systems after Roche lobe overflow and mass exchange as the primaries expand upon leaving the main sequence provides a natural explanation of this difference.
The deviation of the individual stellar radial velocities from the cluster
mean, compared to the combined effects of individual measuring uncertainties
![]() and the internal velocity dispersion in the cluster,
allows to compute individual membership probabilities P(RV) as discussed
by Nordström et al. (1997). The conservative limit
P(RV) < 0.01 is taken
to identify the definite non-members. Stars with a P(RV) indicating
membership, but based only on a single radial velocity (hence unknown binary
status), are labeled "probable'' members, provided their position in the
CMD is also consistent with membership (see Table 1).
and the internal velocity dispersion in the cluster,
allows to compute individual membership probabilities P(RV) as discussed
by Nordström et al. (1997). The conservative limit
P(RV) < 0.01 is taken
to identify the definite non-members. Stars with a P(RV) indicating
membership, but based only on a single radial velocity (hence unknown binary
status), are labeled "probable'' members, provided their position in the
CMD is also consistent with membership (see Table 1).
First, we must compute the cluster mean velocity. The 12 non-variable
red giants in Table 1 yield a mean velocity for IC 4651 of
![]() kms-1, similar to that reported
by Mermilliod et al. (1995) from all 19 cluster giants (
kms-1, similar to that reported
by Mermilliod et al. (1995) from all 19 cluster giants (
![]() kms-1). The standard deviation from the cluster average
of a single mean velocity is 0.75 kms-1 for the red
giants; correcting for the average observational error (0.15 kms-1), we find a true cluster velocity dispersion
of 0.74 kms-1. The velocity dispersion of IC 4651
is thus much larger than that of NGC3680 (0.30 kms-1),
reflecting a larger mass, but maybe also indicating a difference
in the dynamical evolution of the two clusters.
kms-1). The standard deviation from the cluster average
of a single mean velocity is 0.75 kms-1 for the red
giants; correcting for the average observational error (0.15 kms-1), we find a true cluster velocity dispersion
of 0.74 kms-1. The velocity dispersion of IC 4651
is thus much larger than that of NGC3680 (0.30 kms-1),
reflecting a larger mass, but maybe also indicating a difference
in the dynamical evolution of the two clusters.
Using these values for the cluster mean velocity and internal dispersion in
IC 4651 we computed membership probabilities P(RV) for all the stars in Table 1. The mean radial velocity of the 32 single F-type main-sequence
and turnoff members so defined is
![]() kms-1,
with a standard deviation per star of 1.59 kms-1, or 1.42 kms-1 once the average measuring uncertainty of 0.72 kms-1 has been subtracted. The difference from the mean velocity
of the giants, only 0.12 kms-1, is much smaller than that
in NGC 3680 (Nordström et al. 1997), probably because of the improved zero-point
calibration used in the present study (cf. Fig. 2b).
Within the uncertainties, it remains compatible with the difference in
gravitational red-shift between dwarfs and giants, but does not require the use
of different mean velocities for the two groups of stars. The final membership
probabilities P(RV), listed in Table 1, have therefore been
computed with the final weighted mean velocity:
kms-1,
with a standard deviation per star of 1.59 kms-1, or 1.42 kms-1 once the average measuring uncertainty of 0.72 kms-1 has been subtracted. The difference from the mean velocity
of the giants, only 0.12 kms-1, is much smaller than that
in NGC 3680 (Nordström et al. 1997), probably because of the improved zero-point
calibration used in the present study (cf. Fig. 2b).
Within the uncertainties, it remains compatible with the difference in
gravitational red-shift between dwarfs and giants, but does not require the use
of different mean velocities for the two groups of stars. The final membership
probabilities P(RV), listed in Table 1, have therefore been
computed with the final weighted mean velocity:
![]() kms-1.
kms-1.
With these definitions, the P(RV) values in Table 1 classify
86 stars as certain and 5 stars as probable cluster members, while only 13
of the 104 observed stars are found to be field stars, a much lower percentage
than in NGC3680. Of the 13 field stars, 2 are single, 3 are binaries, and 8
are of unknown type. Figure 3 shows the turn-off and red
giant region of the CMD of IC 4651, identifying the different categories of
stars. Because the new photometry by Meibom (2000) approximately doubled
the number of cluster stars just as the CORAVEL observing campaign came
to an end, the CMD still contains many stars without cluster membership
and duplicity data; these are ripe targets for follow-up with multi-fiber
spectrographs.
 |
Figure 3:
a) The upper main sequence and turn-off of IC 4651. Single and binary
cluster members are shown as filled and open circles, respectively; crosses
denote stars without radial-velocity data. MEI numbers are given for the 13 binary stars more than
|
| Open with DEXTER | |
Several determinations of reddening and metal abundance of IC4651 have been
made; see Kjeldsen & Frandsen (1991) for earlier results. Most recently,
Anthony-Twarog & Twarog (2000) made determinations of the reddening and metallicity of
IC 4651:
![]() (sem),
(sem),
![]() (based on the intrinsic colour relation of Olsen (1984),
and
E(b-y) = 0.071,
(based on the intrinsic colour relation of Olsen (1984),
and
E(b-y) = 0.071,
![]() (based on the intrinsic colour
relation of Nissen 1988).
(based on the intrinsic colour
relation of Nissen 1988).
On the basis of uvby-
![]() photoelectric and CCD photometry
Nissen (1988) reported
photoelectric and CCD photometry
Nissen (1988) reported
![]() and
and
![]() as a mean of 10 individual measurements. Anthony-Twarog Twarog (1987) reported
as a mean of 10 individual measurements. Anthony-Twarog Twarog (1987) reported
![]() and
and
![]() as a mean of 66 and 11 measurements, respectively. The new radial velocity
data reveal that of the 10 stars used by Nissen (1988), only 2 are
single members, 7 are binary members and one is of unknown type and
membership. Of the 11 stars used by Anthony-Twarog Twarog (1987) for determination
of reddening, 4 are single members, 5 are binary members, and
2 are non-members.
as a mean of 66 and 11 measurements, respectively. The new radial velocity
data reveal that of the 10 stars used by Nissen (1988), only 2 are
single members, 7 are binary members and one is of unknown type and
membership. Of the 11 stars used by Anthony-Twarog Twarog (1987) for determination
of reddening, 4 are single members, 5 are binary members, and
2 are non-members.
Radial velocity data exist for 46 of the 66 stars used by Anthony-Twarog Twarog (1987)
to determine [Fe/H]. 16 of those are
single members. Using only single member stars,
![]() and
E(b-y) = 0.078 are derived as mean values from the study of
Nissen (1988), and
and
E(b-y) = 0.078 are derived as mean values from the study of
Nissen (1988), and
![]() ,
E(b-y) = 0.074 from the study of
Anthony-Twarog Twarog (1987), making the two studies more consistent.
Adopting a mean reddening of
,
E(b-y) = 0.074 from the study of
Anthony-Twarog Twarog (1987), making the two studies more consistent.
Adopting a mean reddening of
![]() (equivalent to
E(B-V) = 0.11), good agreement with the result by Eggen (1971)
(
(equivalent to
E(B-V) = 0.11), good agreement with the result by Eggen (1971)
(
![]() )
is obtained. Comparison of the
metallicity index (m1) between the present study and Nissen (1988) shows
a mean difference
)
is obtained. Comparison of the
metallicity index (m1) between the present study and Nissen (1988) shows
a mean difference
![]() of
of
![]() .
Thus, the
mean metallicity determined for single member stars from the studies of
Nissen (1988) and Anthony-Twarog Twarog (1987) (
.
Thus, the
mean metallicity determined for single member stars from the studies of
Nissen (1988) and Anthony-Twarog Twarog (1987) (
![]() ), should
be reduced to
), should
be reduced to
![]() .
.
Very recently, Bragaglia et al. (2001)
quote a preliminary determination of
![]() from
high-resolution spectroscopy of five unspecified clump giants. Within the
likely systematic differences between the techniques, this is quite
consistent with the photometric value, in contrast to the case of NGC 3680,
where Pasquini et al. (2001) found
from
high-resolution spectroscopy of five unspecified clump giants. Within the
likely systematic differences between the techniques, this is quite
consistent with the photometric value, in contrast to the case of NGC 3680,
where Pasquini et al. (2001) found
![]() from spectroscopy of red
giants, while the photometric result of Nissen (1988) and
Nordström et al. (1997) was
from spectroscopy of red
giants, while the photometric result of Nissen (1988) and
Nordström et al. (1997) was
![]() .
.
As our final result for the reddening is identical to that adopted in
Paper I, the distance to IC 4651 determined there by direct fitting of the
Hyades main sequence to that of IC4651, (m-M0) = 10.03 or a distance
from the Sun of
![]() kpc, is also adopted here.
kpc, is also adopted here.
Although not all candidate members of IC 4651 have radial-velocity
data, the identification of member and binary stars in the inner region
of the cluster (for
![]() ,
together with the unambiguous identification of the lower main sequence,
allow for a critical test of stellar evolution models. Previous discussions,
e.g. Nordström et al. (1997) or Anthony-Twarog & Twarog (2000) and references in these papers,
have proved conclusively that convective core overshooting must be included
in stellar models for the mass range of IC 4651.
However, present-day models differ in their physical description of the
phenomenon loosely referred to as "overshooting'', leading to subtle
differences in model predictions which can only be tested by comparing
with high-quality photometric data for established, single cluster members.
,
together with the unambiguous identification of the lower main sequence,
allow for a critical test of stellar evolution models. Previous discussions,
e.g. Nordström et al. (1997) or Anthony-Twarog & Twarog (2000) and references in these papers,
have proved conclusively that convective core overshooting must be included
in stellar models for the mass range of IC 4651.
However, present-day models differ in their physical description of the
phenomenon loosely referred to as "overshooting'', leading to subtle
differences in model predictions which can only be tested by comparing
with high-quality photometric data for established, single cluster members.
Two sets of stellar isochrones are considered here: First, the models by
the Geneva group (Schaller et al. 1992), using OPAL radiative opacities
(Iglesias et al. 1992) and an overshooting distance d of
![]() in units of the pressure scale height at the classical core boundary. These
models were calculated for solar metallicity (Z = 0.02). Linear
interpolation in the
in units of the pressure scale height at the classical core boundary. These
models were calculated for solar metallicity (Z = 0.02). Linear
interpolation in the
![]() and
and ![]() of the Geneva models for Z=0.02 and
Z=0.04 (Schaller et al. 1992; Schaerer et al. 1993) allows an approximation to
models for the observed metallicity of IC4651.
of the Geneva models for Z=0.02 and
Z=0.04 (Schaller et al. 1992; Schaerer et al. 1993) allows an approximation to
models for the observed metallicity of IC4651.
Second, we also used the new Yale 2000 isochrones (Yi et al. 2002),
using improved opacities (Iglesias & Rogers 1992) and equations of state
(Rogers et al. 1996). Table 1 in Yi et al. 2002 summarizes the input
physics. Helium diffusion and convective core overshoot
![]() have been taken into consideration. The models are based
on a scaled solar chemical composition, and linear interpolation of
models for Z=0.020 and Z=0.040
was used to approximate the metallicity of IC4651.
have been taken into consideration. The models are based
on a scaled solar chemical composition, and linear interpolation of
models for Z=0.020 and Z=0.040
was used to approximate the metallicity of IC4651.
Colour transformations from effective temperatures to B-V are provided in the Yale models, using both the latest tables of Lejeune et al. (1998) and the modified Green et al. (1987) tables. We have, however, preferred to recompute (b-y) and (v-y) colours from the effective temperatures and metallicities of the models, using the transformation by Edvardsson et al. (1993) for F dwarfs within its range of validity (see Nordström et al. 1997 for a discussion of temperature-colour relations).
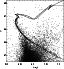 |
Figure 4:
Yale 2000 1.8 Gyr (solid;
|
| Open with DEXTER | |
Figure 4 shows the CMD of IC 4651 with both Geneva (dot-dashed line) and a Yale 2000 isochrone (solid lines) fits to the cluster sequence. Both models fit the turn-off and upper main-sequence region very well, while the Yale model fits the cluster all the way from the turn-off to the faintest part of the lower main-sequence. Below we will focus on the turn-off region and use our membership and duplicity information to make detailed fits to single member stars. However, the excellent match to the well-defined lower main sequence of IC4651 not only makes the isochrone fit much more secure; it is also crucial for determining the contribution of the lower main-sequence stars to the cluster mass (Sect. 5). Determining the present-day mass function of IC4651 is of key importance in the analysis of its dynamical evolution, the main respect in which IC4651 differs from its "sister'' cluster NGC3680.
The true shape of the cluster turn-off is of critical importance for tests of
overshooting in stellar evolution models, and for determining the cluster
age. Our radial velocity data have identified 32 single member stars defining
the cluster sequence in the turn-off region of the CMD, but in contrast to
NGC3680, the whole lower main sequence is now also well defined in IC4651. We
will fit the two sets of stellar evolution models to the turnoff as defined by
the single cluster stars only. Because the theoretical (b-y) colours
are only properly calibrated in the F-dwarf domain, the fits are less
reliable for
![]() (
(
![]() ).
).
For clarity, our isochrone fits to IC4651 are shown in three separate (b-y) vs. V diagrams (Figs. 5, 6, and 7). Each diagram shows the single member stars plotted as large solid dots. The 3-4 single stars (MEI 9745, 10807, 12137, 14560; asterisks) significantly to the right of the well-defined single member sequence cannot be explained by scatter due to the uncertainty in the photometry. More radial velocity observations or new proper motion data might reveal that they are in fact long period binary stars and/or non-members with radial velocities indistinguishable from true cluster members. The smaller dots represent binary member stars and photometric cluster members (i.e., stars without RV data).
Figure 5 shows a fit of the
1.58, 1.69, and 1.82 Gyr
Geneva isochrones, interpolated to the Hyades metallicity
![]() (see above), fitted for
E(b-y) = 0.062. The isochrones
are shifted in magnitude corresponding to
(see above), fitted for
E(b-y) = 0.062. The isochrones
are shifted in magnitude corresponding to
![]() (Crawford & Mandwewala 1976 ). The applied distance modulus of 9.75 is close to the
value (10.03) determined in Meibom (2000) from a direct fit to the Hyades
main sequence, and to the values listed in Kjeldsen & Frandsen (1991) for IC 4651.
(Crawford & Mandwewala 1976 ). The applied distance modulus of 9.75 is close to the
value (10.03) determined in Meibom (2000) from a direct fit to the Hyades
main sequence, and to the values listed in Kjeldsen & Frandsen (1991) for IC 4651.
Figure 6 shows a fit of the 1.51, 1.58, and 1.69 Gyr
Geneva isochrones, interpolated to the Hyades metallicity
![]() ,
fitted for
E(b-y) = 0.071, and shifted in magnitude
corresponding to
,
fitted for
E(b-y) = 0.071, and shifted in magnitude
corresponding to
![]() .
The applied distance
modulus is 9.75.
.
The applied distance
modulus is 9.75.
 |
Figure 5: 1.58, 1.69, and 1.82 Gyr Geneva isochrones for Hyades metallicity fit to the single cluster members (large dots, asterisks) in the turn-off region. Small dots denote binary cluster members and stars without radial-velocity observations. The isochrones are shifted in colour and magnitude corresponding to a reddening of E(b-y) = 0.062. |
| Open with DEXTER | |
 |
Figure 6: 1.51, 1.58, and 1.69 Gyr Geneva isochrones for Hyades metallicity fit to the single cluster members in the turn-off region of IC 4651, but for a reddening of E(b-y) = 0.071. Symbols as in Fig. 5. |
| Open with DEXTER | |
The Geneva models fit the unevolved main sequence and the lower part
of the turnoff well. They also describe the shape of the redward
curvature very precisely all the way to the tip of the main-sequence
turn-off. The best fit for
E(b-y) = 0.062 is obtained using the
1.69 Gyr isochrone, while for
E(b-y) = 0.071 the 1.58 Gyr
isochrone gives the best fit to the single turn-off members. Note
that the 1.58 Gyr Geneva isochrone also fits the only known single
sub-giant member. The ![]() 0.1 Gyr age difference between the
preferred isochrones for
E(b-y) = 0.062 and
E(b-y) = 0.071gives a good estimate of the uncertainty introduced by the reddening
alone.
0.1 Gyr age difference between the
preferred isochrones for
E(b-y) = 0.062 and
E(b-y) = 0.071gives a good estimate of the uncertainty introduced by the reddening
alone.
Figure 7 shows the Yale 2000 1.7, 1.8 and 1.9 Gyr
isochrones (Yi et al. 2002) for
![]() and
E(b-y) = 0.071,
using a distance modulus of 9.72. These new overshooting models also
give a good fit of the turn-off, although the redward curvature is
slightly sharper, suggesting a correction (reduction) of the
overshooting parameter of the Yale models. Note that the ages of
the Yale models are birth-line rather than ZAMS ages, but the
difference (
and
E(b-y) = 0.071,
using a distance modulus of 9.72. These new overshooting models also
give a good fit of the turn-off, although the redward curvature is
slightly sharper, suggesting a correction (reduction) of the
overshooting parameter of the Yale models. Note that the ages of
the Yale models are birth-line rather than ZAMS ages, but the
difference (
![]() 0.05 Gyr) is likely to be negligible in
all but the very youngest clusters.
0.05 Gyr) is likely to be negligible in
all but the very youngest clusters.
 |
Figure 7:
Yale 2000 isochrones for 1.7, 1.8 and 1.9 Gyr, fitted to the
single cluster members in the turn-off region. Symbols as in Figs. 5 and 6. The isochrones have
|
| Open with DEXTER | |
Both the 1.58 Gyr Geneva isochrone and the 1.8 Gyr Yale isochrones give
excellent fits to the established single cluster members in the turn-off
region of IC 4651 for
E(b-y) = 0.071. The relatively small difference
of ![]() 0.2 Gyr in age between the best fit of the two different models
are due to subtle differences in the stellar model parameters. A similar
difference in age between the Geneva models and models constructed using
the Yale Stellar Evolution Code is found for NGC 3680; see
Nordström et al. (1997) vs. Kozhurina-Platais et al. (1997).
0.2 Gyr in age between the best fit of the two different models
are due to subtle differences in the stellar model parameters. A similar
difference in age between the Geneva models and models constructed using
the Yale Stellar Evolution Code is found for NGC 3680; see
Nordström et al. (1997) vs. Kozhurina-Platais et al. (1997).
Figure 8 includes giant cluster members on
the photometric system of Anthony-Twarog & Twarog (2000) (see Meibom 2000, Fig. 2)
together with the Geneva 1.58 Gyr and Yale 1.8 Gyr
isochrones fitted to the single turn-off stars. In the red giant
region solid dots represent single stars and open squares represent
binary stars. The fact that the isochrones do not fit the single
giant members is acceptable because our observations were calibrated
primarily for the main-sequence region, and because the uvbycolour calibrations are less reliable for red giants.
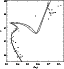 |
Figure 8: Turn-off and red giant stars of IC 4651 with the Geneva (1.58 Gyr, solid line) and Yale isochrones (1.8 Gyr, dotted lines) fitted to the single turnoff members. Dots, asterisks: Single cluster stars; open squares: giant binaries. |
| Open with DEXTER | |
Previous discussions of stellar models tended to debate simply for or against overshooting, resulting in age estimates for IC4651 in the whole range from 1.3 Gyr (Mazzei & Pigatto 1988) to 4.0 Gyr (Maeder 1990), even when based on the same photometry. The need for extended convective cores in stellar evolution models for stars more massive than the Sun is now well established, but the physical modeling of the overshooting phenomenon needs to be better understood; see, e.g. Giménez et al. (1999) for a comprehensive discussion. The tight sequence of single cluster members defined in IC 4651 by our new data should allow further refinement of the overshooting formalism and lead to improved age estimates for IC4651.
Errors in E(b-y) and [Fe/H] will affect the derived cluster age.
The uncertainty of the estimated age is derived from the uncertainties in
[Fe/H] and E(b-y). Anthony-Twarog & Twarog (2000) give 0.003 and 0.012 as the
standard errors of the mean E(b-y) and [Fe/H], respectively,
based on the colour relations of Olsen (1984), but give no estimate
of the uncertainty on the E(b-y) and [Fe/H] as based on the colour
relations of Nissen (1988). A realistic estimate of the uncertainty
in E(b-y) was considered by Nissen (1988) to be ![]() 0.012
corresponding to
0.012
corresponding to ![]() 0.15 Gyr in the models used here. Combining this
with the effect of an uncertainty in [Fe/H] of 0.05 dex leads to an
estimated total uncertainty on the age of IC4651 of
0.15 Gyr in the models used here. Combining this
with the effect of an uncertainty in [Fe/H] of 0.05 dex leads to an
estimated total uncertainty on the age of IC4651 of ![]() 0.15 Gyr.
0.15 Gyr.
The best fit of both the Geneva and Yale 2000
isochrones is obtained using Hyades metallicity
![]() and
E(b-y) = 0.071. Both are close to the values derived
(Sect. 3.3) using our membership information and data from earlier
studies (Anthony-Twarog Twarog 1987; Nissen 1988). The resulting age range is
1.6-1.8 Gyr, leading to a best estimate for the age of IC4651 of
and
E(b-y) = 0.071. Both are close to the values derived
(Sect. 3.3) using our membership information and data from earlier
studies (Anthony-Twarog Twarog 1987; Nissen 1988). The resulting age range is
1.6-1.8 Gyr, leading to a best estimate for the age of IC4651 of
![]() Gyr, not including systematic effects in the models.
IC 4651 therefore appears to be slightly older than its sister
cluster NGC 3680, by
Gyr, not including systematic effects in the models.
IC 4651 therefore appears to be slightly older than its sister
cluster NGC 3680, by
![]() Gyr.
Gyr.
Mass segregation, i.e. increasing central concentration of progressively more massive stars (Spitzer & Mathieu 1987), is seen in several open clusters (see e.g. Raboud & Mermilliod 1998a; 1997; Mathieu 1985). On average, binary systems are more massive than single stars. It is therefore expected to find binaries, together with the most massive single stars, to be concentrated towards the center of a relaxed cluster. If IC4651 is older than its own relaxation time, the currently accepted dynamical models predict the appearance of such segregation of stellar masses; see, e.g, de la Fuente Marcos (1997); Portegies Zwart et al. (2001).
Our photometry and radial-velocity data allow us to test for mass segregation in IC 4651. The radial-velocity data unambiguously classify 86 turnoff or giant stars as single or binary cluster members. From their proximity to the well-defined cluster sequence in the (v-y) vs. V CMD, we identify another 652 stars as probable (photometric) members, especially on the lower main sequence.
The coordinates of the cluster center,
![]() ,
,
![]() ,
were determined as the mean of the
coordinates for the 86 certified single
and binary cluster members. The projected radial distance from the cluster
center was then computed for all 738 known and candidate cluster stars.
Next, the sample was divided into bright and faint subsamples,
corresponding to mass ranges of
,
were determined as the mean of the
coordinates for the 86 certified single
and binary cluster members. The projected radial distance from the cluster
center was then computed for all 738 known and candidate cluster stars.
Next, the sample was divided into bright and faint subsamples,
corresponding to mass ranges of
![]() and
and
![]() ,
respectively. The goal was to
create two samples with comparable numbers of stars (after field star
subtraction), and with the largest possible ratio between the mean masses
of the two samples. Dividing between bright and faint stars at V = 13.4,
corresponding to
,
respectively. The goal was to
create two samples with comparable numbers of stars (after field star
subtraction), and with the largest possible ratio between the mean masses
of the two samples. Dividing between bright and faint stars at V = 13.4,
corresponding to
![]() ,
yielded 144 stars on the more
massive side and 592 stars in the less massive group, for a ratio
between the mean masses of the two samples of 1.89. The observed
field was then divided into 15 annuli with radii from
,
yielded 144 stars on the more
massive side and 592 stars in the less massive group, for a ratio
between the mean masses of the two samples of 1.89. The observed
field was then divided into 15 annuli with radii from
![]() to
to
![]() ,
in steps of one arcminute.
,
in steps of one arcminute.
Using location in the CMD as the only criterion for identifying cluster
stars has the drawback of including a fraction of field stars. The
contamination by field stars increases with increasing magnitude and
becomes significant on the main sequence below
![]() .
.
In order to estimate the radial density distribution of field stars both
brighter and fainter than
![]() ,
we calculated the distance
to the cluster center of field stars in selected areas above and below
the cluster sequence in the CMD. Figure 9 shows the
radial density distribution for the field stars above (solid) and below
(dotted) the lower cluster sequence
,
we calculated the distance
to the cluster center of field stars in selected areas above and below
the cluster sequence in the CMD. Figure 9 shows the
radial density distribution for the field stars above (solid) and below
(dotted) the lower cluster sequence
![]() .
The mean density levels of the two distributions are indicated by horizontal
lines. In order to estimate the density of field stars on the lower cluster
sequence itself we calculated the average of the two mean levels,
.
The mean density levels of the two distributions are indicated by horizontal
lines. In order to estimate the density of field stars on the lower cluster
sequence itself we calculated the average of the two mean levels, ![]() 0.75 star per square arcminute (dashed line). The same mean density levels
were obtained by dividing the total number of stars in each of the two
test groups by the total area of the observed field. A similar estimate
of the field contamination on the upper cluster sequence
0.75 star per square arcminute (dashed line). The same mean density levels
were obtained by dividing the total number of stars in each of the two
test groups by the total area of the observed field. A similar estimate
of the field contamination on the upper cluster sequence
![]() resulted in a field star density of
resulted in a field star density of ![]() 0.08 star per
square arcminute.
0.08 star per
square arcminute.
Note that the method of counting field stars adjacent to the cluster
sequence will inevitably include some cluster binaries above the
sequence and possibly also some cluster stars
scattered into the area below the cluster sequence due to photometric
errors in these faint stars. Correcting for these contributions would
lead to a even lower estimate of the field star density. For the moment,
however, we adopt a field density of 0.08 stars per square arcminute
for
![]() and 0.75 stars per square
arcminute for
and 0.75 stars per square
arcminute for
![]() .
.
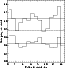 |
Figure 9:
Radial density distribution of field stars just above (solid) and below
(dotted) the lower main sequence of IC4651
|
| Open with DEXTER | |
Figure 10 shows the radial density distribution
of the certain (radial-velocity) and probable (photometric) cluster members.
The cluster star densities do not fall to the estimated field star levels,
even at the edge of the observed field. This is especially striking for the
lower main sequence, but true also for the brighter stars, showing that even
the field observed in the present study was not sufficient to cover the
entire cluster: Additional cluster stars, both faint and bright, are very
likely still to be found outside the currently known field of IC4651.
 |
Figure 10:
Radial density distributions of the bright and faint certain and probable
cluster members. The solid histogram represents the bright sample |
| Open with DEXTER | |
After subtraction of the estimated field star contributions (see Fig.
10), the total numbers of stars in the high-
and low-mass samples defined previously (i.e. brighter and fainter than
![]() )
are 110 and 228,
respectively. The normalized and field-subtracted radial density
distributions of these two samples are shown in Fig. 11.
)
are 110 and 228,
respectively. The normalized and field-subtracted radial density
distributions of these two samples are shown in Fig. 11.
A ![]() test was applied to test for mass segregation in IC4651.
Because the field star levels were determined without use of the radial
density distributions, all radial bins out to
test was applied to test for mass segregation in IC4651.
Because the field star levels were determined without use of the radial
density distributions, all radial bins out to
![]() were used
in the test. The resultant probability that cluster stars with masses
greater and smaller than
were used
in the test. The resultant probability that cluster stars with masses
greater and smaller than
![]() are not drawn from
the same parent radial distribution is 96.4%. It is indeed noticeable
in Fig. 11 that nearly all the turn-off
and red giant stars are located at radii smaller than
are not drawn from
the same parent radial distribution is 96.4%. It is indeed noticeable
in Fig. 11 that nearly all the turn-off
and red giant stars are located at radii smaller than
![]() .
However, the derived formal probability is quite sensitive to the applied
field star correction and the magnitude chosen to separate the two samples.
Overall, we conclude that our data do provide moderately strong evidence
for mass segregation in IC4651.
.
However, the derived formal probability is quite sensitive to the applied
field star correction and the magnitude chosen to separate the two samples.
Overall, we conclude that our data do provide moderately strong evidence
for mass segregation in IC4651.
This investigation of the radial density distribution of cluster
and field stars, together with the perfect match of the isochrones
to the faintest parts of the cluster sequence, indicates a need for
an even deeper photometric study of IC4651 in an even larger field.
A larger field should add many faint, but also some bright stars to the
membership list and also provide a better estimate of the field star
densities from observations at larger radii. This, in turn, would allow
to define better the true richness and size of IC4651, as well as
further improve the definition of the lower cluster main sequence.
Photometric observations in the Johnson V and I bands covering a large
field (
![]() )
around the cluster have already been
obtained by the authors; it would be most interesting to complement these
with a comprehensive radial-velocity survey using a multi-fiber instrument.
If these data were to show that the true size of this "well-known''
cluster is even larger than the factor of two relative to the classical
literature value which we have already found, then one might have serious
doubts about the current catalogue values for other, less well-studied
clusters.
)
around the cluster have already been
obtained by the authors; it would be most interesting to complement these
with a comprehensive radial-velocity survey using a multi-fiber instrument.
If these data were to show that the true size of this "well-known''
cluster is even larger than the factor of two relative to the classical
literature value which we have already found, then one might have serious
doubts about the current catalogue values for other, less well-studied
clusters.
 |
Figure 11:
Normalized and field-subtracted radial density distribution of the
certain and probable cluster members. The solid histogram represents
the high-mass stars |
| Open with DEXTER | |
Based on the relation between stellar mass and V magnitude
for the best-fit Geneva and Yale 2000 isochrones, masses for
individual stars were derived. Fifteen brightness intervals
were used to divide the cluster mass range from
![]() to
to
![]() into mass intervals with a roughly constant
width of
into mass intervals with a roughly constant
width of
![]() .
Single radial-velocity members are then
simply assigned a mass according to their V magnitude.
.
Single radial-velocity members are then
simply assigned a mass according to their V magnitude.
Decomposition of binaries. Stars identified as binaries
from the radial-velocity data (except MEI 11507, a possible blue
straggler) were decomposed into single stars in the following way:
Assuming a mass-luminosity relation of
![]() on the upper
main-sequence (Christensen-Dalsgaard 1993), a displacement of
on the upper
main-sequence (Christensen-Dalsgaard 1993), a displacement of
![]() in V from the best-fit isochrone corresponds to a mass ratio between
the primary and secondary component of
in V from the best-fit isochrone corresponds to a mass ratio between
the primary and secondary component of ![]() 2. Therefore, binaries
located within
2. Therefore, binaries
located within
![]() from the
isochrone are assumed to consist of a primary component with a mass
corresponding to that of the isochrone, and a secondary component with
half that mass.
from the
isochrone are assumed to consist of a primary component with a mass
corresponding to that of the isochrone, and a secondary component with
half that mass.
For each of the 13 binaries located more than
![]() above the best-fit isochrone (MEI 7753, 6725, 6726, 11670, 7309, 9278,
14364, 8302, 11507, 5658, 8080, 9357, 11504; see Fig. 3a),
we assumed a series of possible secondary components on the lower
main sequence. Next, corresponding primary component loci in the CMD
were computed so as to match the observed location of the binary. The
intersection between the track for the primary component and the
cluster sequence then fixes the brightness and therefore the mass of
both the primary and secondary star. Figure 12 shows an
example of such a primary track for the binary MEI6725.
above the best-fit isochrone (MEI 7753, 6725, 6726, 11670, 7309, 9278,
14364, 8302, 11507, 5658, 8080, 9357, 11504; see Fig. 3a),
we assumed a series of possible secondary components on the lower
main sequence. Next, corresponding primary component loci in the CMD
were computed so as to match the observed location of the binary. The
intersection between the track for the primary component and the
cluster sequence then fixes the brightness and therefore the mass of
both the primary and secondary star. Figure 12 shows an
example of such a primary track for the binary MEI6725.
 |
Figure 12:
Binary stars (circles) near the turn-off of IC 4651 together with
the best-fit Geneva isochrone. For a sequence of assumed lower
main-sequence secondaries, the filled circles show the locus of the
matching primary components reproducing the observed position of the
binary system MEI6725. The primary component on the isochrone has a
mass of
|
| Open with DEXTER | |
By the procedures illustrated above, the 42 known binary systems were
decomposed into single stars. Figure 13 shows the mass
function of the individual stars resulting from the decomposition plus
the single stars identified by radial velocity measurements. The
estimated total mass contribution from this group of cluster stars is
![]() .
.
The many (![]() 650) new cluster stars found at all magnitudes in our
CCD photometry have no observational data on membership and duplicity
as yet. Nevertheless, they more than double the number of cluster
members in the turnoff region, and the entire low-mass cluster population
is a new discovery. Their contribution to the total cluster mass and mass
function is therefore important and cannot be neglected. The method we
have used to estimate masses for these "new'' photometric cluster stars
is similar to that used for the established radial-velocity members.
650) new cluster stars found at all magnitudes in our
CCD photometry have no observational data on membership and duplicity
as yet. Nevertheless, they more than double the number of cluster
members in the turnoff region, and the entire low-mass cluster population
is a new discovery. Their contribution to the total cluster mass and mass
function is therefore important and cannot be neglected. The method we
have used to estimate masses for these "new'' photometric cluster stars
is similar to that used for the established radial-velocity members.
The number of field stars in each mass bin was determined using the method described in Sect. 5.1: in each brightness interval (corresponding to a mass interval), stars were counted in two areas bordering the cluster sequence. The mean density of stars was used to estimate the number of field stars overlapping the cluster sequence. As explained above, this method is likely to slightly overestimate the field star density. After field subtraction 301 stars were left on the cluster sequence.
Due to mass segregation, however, the binary fraction amongst the
outlying stars is probably smaller than the 55% found in Sect. 3.1
for the turnoff stars in the inner region; we have assumed an average
binary fraction of 40% (120 of the 301 candidate members). We also
assume that each binary consists of a primary component with mass
corresponding to its location on the isochrone and a secondary
component with half that mass. The resulting mass function for
the "new'' main-sequence stars is shown in Fig. 14.
The lack of stars with mass of
![]() is due to the
effects of binning. The estimated contribution to the cluster mass
from the 421 individual stars (181 single stars, 120 primary components,
and 120 secondary components) is
is due to the
effects of binning. The estimated contribution to the cluster mass
from the 421 individual stars (181 single stars, 120 primary components,
and 120 secondary components) is
![]() .
.
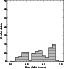 |
Figure 13: The mass function of the single members and the components of the 42 decomposed binaries. |
| Open with DEXTER | |
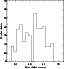 |
Figure 14: Mass function of the 421 individual outer-field and lower main-sequence photometric members (181 single stars, 120 primary components, and 120 secondary components). |
| Open with DEXTER | |
Summing the mass contributions from the two groups of stars with and
without radial velocity measurements, we estimate the present total
mass of IC4651 within our
![]() field
to be
field
to be
![]() .
.
With masses now estimated for every plausible cluster star, the
dynamical state of IC 4651 can be discussed in more quantitative
terms. Figure 15 shows the overall present-day
mass function for IC 4651. The open histogram includes the masses
of both single stars and individual components of decomposed binary
systems. The shaded histogram shows the subsample of stars with radial
velocity measurements. For comparison, we also show the three different
initial mass functions (IMFs) by Salpeter (1955, solid), Miller & Scalo (1979, dotted) and Kroupa et al. (1993, dashed), normalized
to the total number of stars in the range
![]() and scaled
to match the number of single stars now observed in the mass bin
and scaled
to match the number of single stars now observed in the mass bin
![]() .
Comparing the IMFs with the present-day mass
function enables us to estimate the total initial number and mass
of stars in IC4651 as well as the fraction lost during the lifetime
of the cluster.
.
Comparing the IMFs with the present-day mass
function enables us to estimate the total initial number and mass
of stars in IC4651 as well as the fraction lost during the lifetime
of the cluster.
The following results for the initial number of stars and total mass
of IC 4651 are obtained:
![]() ,
,
![]() ,
and
,
and
![]() for the Salpeter, Miller-Scalo and
Kroupa et al. IMFs, respectively. Adopting the least extreme
Miller-Scalo IMF (which Padoan et al. 1996 support from both theory
and observations), we find that IC 4651 now contains only
for the Salpeter, Miller-Scalo and
Kroupa et al. IMFs, respectively. Adopting the least extreme
Miller-Scalo IMF (which Padoan et al. 1996 support from both theory
and observations), we find that IC 4651 now contains only ![]() 7%
of its original stars and
7%
of its original stars and ![]() 12% of its initial mass, all computed
within the observed field. We note, however, that also stars in the mass
range 1.75-
12% of its initial mass, all computed
within the observed field. We note, however, that also stars in the mass
range 1.75-
![]() may well have been lost from the cluster.
Our estimate of the initial cluster mass as well as the mass lost from
the cluster is therefore likely to be somewhat conservative.
may well have been lost from the cluster.
Our estimate of the initial cluster mass as well as the mass lost from
the cluster is therefore likely to be somewhat conservative.
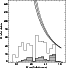 |
Figure 15:
Overall present-day mass function for IC 4651 together with the IMFs
by Salpeter (solid), Miller & Scalo (dotted) and Kroupa et al.
(dashed), normalized to match the
|
| Open with DEXTER | |
Mass loss from the cluster can be due to two different processes: 1) Evolution of high mass stars, and 2) evaporation of low mass stars (dynamical mass loss). We discuss these in turn.
1): the original, most massive cluster stars in the
range
![]() -
-
![]() have completed their
evolution to white dwarf or neutron star remnants. The above IMFs
predict that IC4651 initially contained 308-490 stars in the mass
range
have completed their
evolution to white dwarf or neutron star remnants. The above IMFs
predict that IC4651 initially contained 308-490 stars in the mass
range
![]() .
Adopting the Miller-Scalo IMF, a total of
.
Adopting the Miller-Scalo IMF, a total of
![]() or
or ![]() 35% of the initial cluster mass has
thus been lost due to evolution of the
35% of the initial cluster mass has
thus been lost due to evolution of the ![]() 430 most massive stars
in the young IC4651 (
430 most massive stars
in the young IC4651 (![]() 5% of the initial population)
5% of the initial population)
Nordström et al. (1997) also found that NGC3680 has lost ![]() 30% of
its initial mass due to evolution of its high mass stars. This similarity
is indeed expected because the ages and turn-off masses
30% of
its initial mass due to evolution of its high mass stars. This similarity
is indeed expected because the ages and turn-off masses
![]() of the two clusters are nearly identical. We
note that both in IC4651 and NGC3680, a substantial fraction of the
high-mass component might still be present in the form of stellar
remnants, primarily white dwarfs. Such white dwarfs are expected to be
bright enough to remain observable with medium-sized telescopes.
of the two clusters are nearly identical. We
note that both in IC4651 and NGC3680, a substantial fraction of the
high-mass component might still be present in the form of stellar
remnants, primarily white dwarfs. Such white dwarfs are expected to be
bright enough to remain observable with medium-sized telescopes.
2): low-mass stars are lost from the central regions of the
cluster due to mass segregation, and again from the outer parts due to
galactic tidal effects (de la Fuente Marcos 1997; Terlevich 1987). Formation of an
outer halo of low-mass stars at 1-2 tidal radii from the cluster center
(Terlevich 1987) causes the outermost stars to dynamically evaporate
from the cluster. In IC4651, many of the newly discovered lower main-sequence
stars are located outside the central field observed in previous studies.
Above, we have found indications of moderate mass segregation (see
Sect. 5.1), and we have also shown that additional cluster stars
are likely to be found at even greater distances from IC4651 than
those covered in our photometric survey field, the radius of which
is less than half the estimated tidal radius of IC4651
![]() .
Further studies may succeed in mapping the outer halo of IC4651 and
define its true radial extent.
.
Further studies may succeed in mapping the outer halo of IC4651 and
define its true radial extent.
From the information available within our observed fields, IC 4651 appears
to have lost ![]() 53% of its original mass due to evaporation of low-mass
stars
53% of its original mass due to evaporation of low-mass
stars
![]() ,
corresponding
to
,
corresponding
to
![]() 88% of the initial number of stars. For comparison,
NGC3680 was found to have lost
88% of the initial number of stars. For comparison,
NGC3680 was found to have lost
![]() 90% of its original low-mass
stars (Nordström et al. 1997), containing
90% of its original low-mass
stars (Nordström et al. 1997), containing ![]() 60% of the cluster mass within
the central
60% of the cluster mass within
the central
![]() .
.
Our "twin'' clusters IC4651 and NGC3680 are almost identical in age, turn-off mass, and metallicity. They differ strongly, on the other hand, in present (and perhaps also initial) stellar population and total mass. Both clusters have undergone significant dynamical evolution, but NGC3680 is clearly in a far more advanced stage than IC4651, judged by our results on mass segregation and evaporation of low-mass stars. In fact, if significant numbers of stars of all masses were lost from NGC3680 in this process, the two clusters could have been even more similar at birth.
As with other twins, it is natural to search for an explanation of these differences in terms of environmental effects: theory (e.g. Terlevich 1987) suggests that a single close encounter with a massive object may completely disrupt an open cluster in about 108 yr. Depending on their orbits in the Galactic disk, both clusters will have been exposed to close passages of giant molecular clouds or other massive objects which may cause tidal stripping of their low mass stars, and indeed Friel (1995) demonstrated that no open clusters older than the Hyades have survived significantly closer to the Galactic center than the Sun.
The galactic positions of the two clusters are:
![]() ,
,
![]() and r = 1.0 kpc for IC4651; and
and r = 1.0 kpc for IC4651; and
![]() ,
,
![]() and r = 1.3 kpc for NGC3680. IC4651 is thus closer
to both the galactic plane and the galactic center than NGC 3680 and would
naively be expected to be more, not less dynamically evolved than the
latter as we observe.
and r = 1.3 kpc for NGC3680. IC4651 is thus closer
to both the galactic plane and the galactic center than NGC 3680 and would
naively be expected to be more, not less dynamically evolved than the
latter as we observe.
In order to explore whether the current positions of the two clusters are a
good guide to their dynamical histories, the brightest stars in both were
identified in the TYCHO2 catalogue Høg et al. (2000). Average absolute proper
motions were computed to be
![]() ,
,
![]() masyr-1 (IC4651, 6 stars) and
masyr-1 (IC4651, 6 stars) and
![]() ,
,
![]() masyr-1 (NGC3680, 7 stars); their uncertainty is about
1 masyr-1, depending mostly on which outlying points are eliminated.
From these and their known
positions, distances, and mean radial velocities, the space motions given in
Table 3 follow. From these in turn, the orbital motions were
integrated backwards for 1.6 Gyr in the Dehnen & Binney (1998) axisymmetric
Galactic potential (their Model 2, assuming a Solar galactocentric distance
of R0 = 8 kpc). The resulting orbital parameters are also listed
in Table 3. Uncertainties in (U,V,W) due to errors in proper
motions, distances, and radial velocities are evaluated to be of the order
of 2 kms-1, in the orbital parameters about 0.1 kpc.
masyr-1 (NGC3680, 7 stars); their uncertainty is about
1 masyr-1, depending mostly on which outlying points are eliminated.
From these and their known
positions, distances, and mean radial velocities, the space motions given in
Table 3 follow. From these in turn, the orbital motions were
integrated backwards for 1.6 Gyr in the Dehnen & Binney (1998) axisymmetric
Galactic potential (their Model 2, assuming a Solar galactocentric distance
of R0 = 8 kpc). The resulting orbital parameters are also listed
in Table 3. Uncertainties in (U,V,W) due to errors in proper
motions, distances, and radial velocities are evaluated to be of the order
of 2 kms-1, in the orbital parameters about 0.1 kpc.
| Cluster | U | V | W |
|
|
|
e |
| IC 4651 | -22 | 6 | 4 | 7.0 | 10.2 | 0.20 | 0.19 |
| NGC 3680 | -22 | -10 | -7 | 7.2 | 8.3 | 0.41 | 0.07 |
Table 3 reveals a non-trivial result: The mean galactocentric distance of IC4651 (8.6 kpc) is in fact larger than that of NGC3680 (7.7 kpc) despite the fact that they happen to lie in the reverse order at present. Thus, the actual orbits of the two clusters provide a natural explanation why NGC3680 is the more evolved of the two, a situation that appeared puzzling when only a snapshot of their present locations was considered. A further step, which we have not taken, would be to follow these orbits backwards in time and search for any close passages of massive objects in the disk - a task which would also require building an inventory of such objects and their velocities.
Our new Strömgren uvby photometry and long-term radial velocity measurements have substantially deepened our understanding of IC4651. The detailed analysis presented in this paper places IC4651 in the small company of open star clusters for which data of similar detail and completeness exist (i.e., the Hyades, Pleiades, Praesepe, NGC3680, and NGC6231).
The present study has radically revised previous views of IC4651 as
regards mass, stellar population, and size. In addition, our
understanding of its present structure and dynamical state has been
greatly improved. Together with NGC 3680 (Nordström et al. 1997),
IC4651 now provides an important benchmark for studies of the
dynamical evolution of open star clusters.
Our main results on IC4651 can be summarized as follows:
1) IC4651 is at least twice as rich in stars as previously thought, and the main sequence is well defined
to
![]() .
.
2) From precise radial-velocity data for 104 stars brighter than
![]() ,
the membership and duplicity of these stars have been investigated.
In total, 86 (80%) of the 104 stars are found to be cluster members;
of these, 42 (49%) are spectroscopic binaries. 5 stars are classified
as "possible members'', and 13 (13%) are field stars. Thus, at least
for the brighter sample of cluster stars, the frequency of binaries is
high (similar to NGC 3680, Nordström et al. 1997), while field star
contamination is relatively unimportant.
,
the membership and duplicity of these stars have been investigated.
In total, 86 (80%) of the 104 stars are found to be cluster members;
of these, 42 (49%) are spectroscopic binaries. 5 stars are classified
as "possible members'', and 13 (13%) are field stars. Thus, at least
for the brighter sample of cluster stars, the frequency of binaries is
high (similar to NGC 3680, Nordström et al. 1997), while field star
contamination is relatively unimportant.
From 12 single red-giant and 32 single F-type main-sequence
members we determine a mean cluster radial velocity of
![]() kms-1. The internal radial-velocity dispersion as
determined from the single giants and corrected for observational
errors, is 0.74 kms-1.
kms-1. The internal radial-velocity dispersion as
determined from the single giants and corrected for observational
errors, is 0.74 kms-1.
The single main-sequence stars in IC 4651 have a mean rotational
velocity of
![]() km s-1 (standard error
of the mean), with a standard deviation of 13.1 kms-1.
km s-1 (standard error
of the mean), with a standard deviation of 13.1 kms-1.
3) Using only single member stars measured by
Nissen (1988), and Anthony-Twarog Twarog (1987) and calculating the
metallicity-index (m1) from the new uvby data, the reddening
and metallicity of IC 4651 were found to be
![]() and
and
![]() .
The latter is in good agreement with the
recent preliminary spectroscopic determination by Bragaglia et al. (2001).
The distance as determined from a direct fit to the Hyades main sequence
is
.
The latter is in good agreement with the
recent preliminary spectroscopic determination by Bragaglia et al. (2001).
The distance as determined from a direct fit to the Hyades main sequence
is
![]() kpc.
kpc.
4) Two sets of stellar evolution models fitted to the
single cluster members in the turn-off show that overshooting from the
convective cores is significant in IC 4651. The preferred models have
![]() (Hyades) and are fit for
E(b-y) = 0.071.
We estimate the age of IC 4651 to be in the range 1.6-1.8 Gyr, with
a mean value of
(Hyades) and are fit for
E(b-y) = 0.071.
We estimate the age of IC 4651 to be in the range 1.6-1.8 Gyr, with
a mean value of
![]() Gyr.
Gyr.
5) From the best-fit isochrone, we have assigned
individual masses to all stars in IC4651; the mass at the tip
of the turn-off is
![]() .
Binaries were decomposed
into their primary and secondary components, and the contribution to
the mass function from the secondary components of known spectroscopic
binaries as well as suspected binaries in the outer field was estimated.
The current total mass of IC4651 within our field of
.
Binaries were decomposed
into their primary and secondary components, and the contribution to
the mass function from the secondary components of known spectroscopic
binaries as well as suspected binaries in the outer field was estimated.
The current total mass of IC4651 within our field of
![]() is estimated to be
is estimated to be
![]() ,
leading to
an estimated tidal cutoff radius of
,
leading to
an estimated tidal cutoff radius of
![]() .
.
6) The initial stellar population and total mass
of the cluster are estimated by fitting three initial mass
functions to the present-day mass function. Using the least
extreme IMF (Miller-Scalo), IC 4651 is estimated to have contained
![]() 8300 stars initially, with a total mass of
8300 stars initially, with a total mass of
![]() .
Thus, only
.
Thus, only ![]() 7% of the original cluster stars and
7% of the original cluster stars and ![]() 12%
of the initial mass now remain within the field studied.
12%
of the initial mass now remain within the field studied.
Of the initial cluster mass, about ![]() 35%
35%
![]() has been lost due to the evolution of the most massive stars,
while
has been lost due to the evolution of the most massive stars,
while ![]() 53% has been lost dynamically, corresponding to
53% has been lost dynamically, corresponding to
![]() 88% of the initial population of low mass cluster stars.
Further studies may reveal how large a fraction
of the low mass stars may remain gravitationally bound in an
extended halo around IC4651. The spatial distribution of the cluster
members and field stars strongly suggests that enlarging the
observed field should lead to the detection of even more distant
cluster stars; the field studied so far still only reaches about
half the estimated tidal radius.
88% of the initial population of low mass cluster stars.
Further studies may reveal how large a fraction
of the low mass stars may remain gravitationally bound in an
extended halo around IC4651. The spatial distribution of the cluster
members and field stars strongly suggests that enlarging the
observed field should lead to the detection of even more distant
cluster stars; the field studied so far still only reaches about
half the estimated tidal radius.
7) Computing the Galactic orbits of both clusters reveals that IC4651 is, on average, more distant from the Galactic center than NGC3680, although the opposite is true for their present locations. This may provide a natural explanation why NGC3680 is dynamically more evolved than IC4651 despite their identical ages.
Acknowledgements
We thank Michael I. Andersen and Frank Grundahl for help in planning and performing the photometric observations and data reduction, and Stephane Udry and Michel Mayor for reducing the CORAVEL observations in Geneva. We are much indebted to Dr. Johan Holmberg, Lund Observatory, for computing the space motions and Galactic orbits discussed in Sect. 5.3. Finally, we thank Robert D. Mathieu and Sydney Barnes for helpful discussions and comments and Sukyoung Yi for providing the new Yale isochrones.
This study has been supported by the Danish Natural Science Research Council via its Ground Based Astronomical Instrument Center, and by the US National Science Foundation under grant No. AST9731302 (R. D. Mathieu, P.I.). S. Meibom gratefully acknowledges a Ph.D. fellowship from "Forskeruddannelsesrådet" (The Danish Research Academy). B. Nordström thanks the Carlsberg Foundation and the Swedish Research Council for financial support.