![\begin{figure}
\par\includegraphics[width=8.5cm,clip]{1127SBP.EPS}
\end{figure}](/articles/aa/full/2001/46/aa10308/Timg1.gif) |
Figure 1: Radio burst on November 27, 1999 (thick line: right polarization component; thin line: left polarization component). |
| Open with DEXTER | |
A&A 380, 318-322 (2001)
DOI: 10.1051/0004-6361:20011121
M. Wang1,2 - Q. Fu1 - R. Xie1 - Ch. Duan1,2
1 - Yunnan Observatory, National Astronomical Observatories,
Chinese Academy of Science, Kunming 650011, PR China
2 -
National Astronomical Observatories,
Chinese Academy of Science, Beijing 100012, PR China
Received 26 September 2000 / Accepted 27 June 2001
Abstract
Two special fine structures observed with the 5.2-7.6 GHz high temporal and
spectral resolution spectrometer of Beijing Astronomical Observatory (BAO)
are described and analyzed in this paper. Because they appear as the letters N and M on dynamic spectra, we call them centimetric type N and type
M bursts, respectively. They have very short durations (about 500 ms and 300 ms), small flux densities (tens of SFU), narrow bandwidth (relative bandwidth
about 10%), and very small polarization degree (close to the accuracy limit
of observation instruments), but each branch has considerable high
frequency drift rates (several GHz/s). We think that they are an extension of
metric or decimetric type U(N) bursts in centimetric wavelengths.
Type N and type M bursts may be due to the mirroring effect
near the foot point, as well as a scattering effect caused by whistler wave
turbulence near the top of loop. They may also be interpreted by the
quasi-periodic particle injection into coronal loops.
Key words: Sun: activity - Sun: corona - Sun: general - Sun: radio radiation
As the sensitive tracer of propagation of subrelativistic electron beams along open magnetic field lines in solar corona and interplanetary medium, solar radio type III bursts have been studied for more than 40 years. Type III bursts have not been understood clearly, and have captured the attention of solar physicists up to now.
If electron beams travel along closed magnetic field lines, the radio bursts will appear as an inverted capital U on the dynamic spectra. Type U bursts are also interpreted as being produced by subrelativistic electron beams (e.g. Maxwell & Swarup 1958; Stewart 1974; Benz et al. 1977; Benz et al. 1979). Some type U bursts, in which the third ascending branch following the second descending branch are observed instantly, this bursts appears as a capital letter N on the dynamic spectra (Dumas et al. 1982; Caroubalos et al. 1987; Hillaris et al. 1988). With the development of high temporal and high spectral resolution and high sensitivity observation instruments, type III bursts, as well as the type U(N) bursts have been observed in decimetric wavelengths (Güdel & Benz 1988; Aurass & Klein 1997; Aschwanden et al. 1992). However we note that no type U(N) bursts have been reported in centimetric wavelengths.
In this paper, we introduce two centimetric fine structures observed with high temporal (5 ms) and spectral (20 MHz) resolution by a new digital multi-channel spectrometer of the Beijing Astronomical Observatory (BAO). On the dynamic spectra, these two fine structures appear as capital N and M very clearly, therefore, we call them centimetric type N and type M bursts, respectively. This may be the first observation of type N/M bursts in such high frequencies. Because of the similarity to the type N burst in lower frequencies on the dynamic spectra, we think that they are extension of metric and decimetric type N bursts, and they are also due to the plasma emission excited by electron beams.
In Sect. 2, the type N and M bursts are described in detail, and the important observational parameters are measured and analyzed. The discussion and conclusions are given in Sects. 3 and 4.
The solar radio spectrometer of BAO has been in operation since July 1999. The total bandwidth is 2400 MHz, which is covered by 120 channels with a bandwidth of 20 MHz each. The temporal resolution is 5 ms. The flux density and circular polarization of solar radio bursts are recorded digitally. The sensitivity is better than 2% of the quiet-Sun level, and the accuracy of the polarization measurement is better than 5-10% (see Fu et al. 1995, for details).
As shown in Fig. 1, a small solar radio burst with several impulses superimposed was observed at 04:51-04:56 UT on 27 November 1999. According to the Solar Geophysical Data (SGD), a C9.9/1N flare in the Active Region NOAA 8778 located at S12 W15 was recorded. Generally, it is difficult to observed the fine structures in centimetric wavelengths.
![\begin{figure}
\par\includegraphics[width=8.5cm,clip]{1127SBP.EPS}
\end{figure}](/articles/aa/full/2001/46/aa10308/Timg1.gif) |
Figure 1: Radio burst on November 27, 1999 (thick line: right polarization component; thin line: left polarization component). |
| Open with DEXTER | |
At 04:52 UT (corresponding to the impulsive phase of the flare), two fine structures was found at 04:52:21 UT and 04:52:23 UT, respectively. Figures 2 and 3 show their dynamic spectra.
![\begin{figure}
\par\includegraphics[width=8.2cm,clip]{wm_n.ps}
\end{figure}](/articles/aa/full/2001/46/aa10308/Timg2.gif) |
Figure 2: Dynamic spectrum of type N burst on November 27, 1999. |
| Open with DEXTER | |
![\begin{figure}
\par\includegraphics[width=8.2cm,clip]{wm_m.ps}
\end{figure}](/articles/aa/full/2001/46/aa10308/Timg3.gif) |
Figure 3: Dynamic spectrum of type M burst on November 27, 1999. |
| Open with DEXTER | |
In Fig. 2, there is an undeveloped type U (or J) burst, immediately followed by the third ascending branch at the end of the descending branch, appearing as a capital letter N on the dynamic spectrum. As its counterpart in metric or decimetric wavelengths, we call it a centimetric type N burst. Another branch with a slower frequency drift rate (reverse drift) near the beginning of the second descending branch was found.
In Fig. 3, there is a typical type U burst first, then the third ascending branch followed the second descending branch and developed to a weaker type U burst, appearing as a capital letter M on the dynamic spectrum. Thus, we call it a centimetric type M burst.
The total duration of the type N burst is about 485 ms, and the total
bandwidth
is about 0.84 GHz (from 5.24 to 6.08 GHz), i.e., the relative bandwidth
of the type N burst (
![]() )
is about 15%. The bandwidth
of the second descending branch is much broader than the other two branches.
)
is about 15%. The bandwidth
of the second descending branch is much broader than the other two branches.
The total duration of the type M burst is about 270 ms. The total bandwidth
of it is about 0.44 GHz (5.50 to 6.04 GHz), i.e., the relative bandwidth of
the type M burst (
![]() )
is about 9%.
)
is about 9%.
From Figs. 2 and 3, we found that the polarization degrees of these two bursts are very weak, close to the accuracy limit of polarization measurement of the instruments.
The observational characteristics of each ascending branch (AB) and
descending branch (DB) of the type N and type M bursts, such as typical
half power duration D, typical flux density I, bandwidth
![]() ,
and frequency drift rate d
,
and frequency drift rate d![]() /dt are listed in Tables 1 and 2,
respectively.
/dt are listed in Tables 1 and 2,
respectively.
| Branch | D(ms) | I(SFU) |
|
d |
| 1st AB | 40 | 15 | 0.24 GHz | 3.40 GHz/s |
| 2nd DB | 80 | 30 | 0.86 GHz | 2.50 GHz/s |
| 3rd AB | 30 | 20 | 0.34 GHz | 9.70 GHz/s |
| Branch | D(ms) | I(SFU) |
|
d |
| 1st AB | 80 | 25 | 0.50 GHz | 7.10 GHz/s |
| 2nd DB | 70 | 30 | 0.42 GHz | 5.10 GHz/s |
| 3rd AB | 45 | 15 | 0.42 GHz | 6.00 GHz/s |
| 4th DB | 40 | 15 | 0.34 GHz | 9.70 GHz/s |
The centimetric type N and M bursts are reported here, probably for the first time. Because of the similarity of the spectral morphology between the centimetric and decimetric (or metric ) type U(N/M) bursts, we can propose that the centimetric type U(N/M) bursts are the extension of metric and decimetric type U(N) burst.
It is obvious that the total duration of type U(N) bursts decreases with increasing frequency, from 5-40 s at metric bands (Suzuki & Dulk 1985), to several seconds in the frequency range of 150-470 MHz (Caroubalos et al. 1987), to about 1 s at decimetric bands (Aschwanden et al. 1992), to several hundreds of milliseconds at the centimetric bands of our observations. The reason is that the electron beams would travel through a denser medium in the lower corona, where the beams are isotropized faster by Coulomb collisions. So the duration of electron beams is shorter in the lower corona than in the higher corona. A shorter duration of type U bursts also implies smaller loops.
The statistical results of Caroublos et al. (1987) showed that the durations increased along the three successive branches of type N burst, and they believed that it was strong evidence that the three branches were due to the same electron beams. Our observations are not consistent with their results. Because there are only two samples of type N/M bursts, we cannot obtain more reliable relationships between duration of the successive branches and time. A possible interpretation of our observations is that not all electrons were mirrored, and then excited the third ascending branch.
Like metric and decimetric type U(N) burst, the centimetric type N and M bursts have lower degrees of polarization. This suggests that the centimetric type U(N/M) bursts are due to the second harmonic plasma emission, because the polarization degree of the fundamental plasma emission is generally very high.
As noted earlier, the circular polarization degree of the type N and M bursts are too small to be measured precisely, their values are less than 10% throughout the burst, close to the accuracy limits of our instruments. This is consistent with previous observations (e.g. Aschwanden et al. 1992; Aurass & Klein 1997). From this, we can conclude that their emission mechanism is the second harmonic plasma emission.
At lower frequencies (especially less than 100 MHz), the two branches of the type U bursts generally have different signs of polarization because of the different magnetic field direction to the earth. But at higher frequencies, type U bursts show that both branches have almost the same sense of polarization. The interpretation of this phenomenon is given in terms of the mode coupling, i.e., the quasi-transverse region in the overlying loop systems (Benz et al. 1977). No obvious polarization reversal was detected in centimetric type U(N/M) bursts.
The relative bandwidth of centimetric type U(N/M) bursts is about 10%, close to the results of Aschwanden et al. (1992), but shorter than the statistical results of metric and decimetric type U(N) bursts of Aurass et al. (1997).
Centimetric type U(N/M) bursts have a much higher frequency drift rate (several GHz/s) than that of type U bursts in the lower frequencies (about 100 MHz/s). This may suggest a faster beam velocity and smaller density scale height in the centimetric type U(N/M) bursts.
It is generally accepted that metric and decimetric type U bursts are due to the subrelativistic electron beams travelling along the closed magnetic field lines. Subsequently, the third ascending branches of type N are caused by the same electron beams, which are mirrored in the vicinity near the foot point of the corona loop. This idea was supported by the numerical simulation (Hillaris et al. 1988; Kalický et al. 1996) and the analysis combined with spatial resolution data (Aurass & Klein 1997).
If the reflected beams travel along the closed magnetic fields lines, it may excite the second type U burst again, resulting in a type M burst on the dynamic spectra.
Furthermore, Karlický et al. (1996) suggested that type U(N) bursts can be not only excited by the mirroring effect but also by the scattering of electron beams at a zone of enhanced whistler wave turbulence near the top of the loop.
In Fig. 2, the beginning of the second descending branch (from 5.42 to 5.62 GHz) has another branch with a slower frequency drift rate (reverse drift). This appears somewhat like the capital letter U (or J). The dynamic spectrum of Fig. 2 is very similar to Figs. 5B and 10A of Karlický et al. (1996). Now we can describe the whole process of this type N burst. After the electron beams traveled through the top of the loop, some electrons were scattered by the whistler wave turbulence, and formed the type U(J) bursts near the beginning of the second descending branch. The other electrons continued to travel downwards; when they were close to the foot point of the loop, they were mirrored and excited the third ascending branch.
For the type M burst, the reflected beam traveled in the initial loop and excited another relatively weaker type U burst. Because of the loss of energy and velocity, the reflected beam could not reach the region as high as the first type U burst, so its turnover frequency (about 5.58 GHz) was slightly greater than that of the first type U burst (about 5.50 GHz).
Type U bursts are much rarer than the normal type III bursts. Furthermore, the results of Aurass & Klein (1997) indicated that only 10% out of the total type U bursts in decimetric wavelength were type N bursts.
Because of the collisions with the ambient plasma, the beams will be decelerated and lose their energy. So the emission excited by the beams would be fainter and fainter. The previous observations and our data support this conclusion. For subrelativistic electron beams, they will be isotropized within one second in the lower corona. We think that this is why we could not observe frequently type U(N/M) bursts.
There may be alternative ways of forming a type N and type M burst. Quasi-periodic sequences of type III bursts were not rare during flares (e.g. Zhao et al. 1991), and decimetric type U bursts in quasi-periodic sequences have been also observed (Aschwanden et al. 1993). The periodicity of type III and type U bursts can be interpreted by the model of quasi-periodic particle (electrons) injection (Aschwanden et al. 1993, 1994). Especially, if the period of the subsequent type U burst is similar to the duration of the type U burst, a chance coincidence of the end of a first type U burst with the start of the second type U burst, which make a type M burst, is relatively high.
According to the observational characteristics of the centimetric type N and M bursts, we can estimate the parameters of the source. All the following calculations are based on the assumption of the second harmonic plasma radiation.
The electron density is given by the relation between the observed frequency
and source plasma frequency, from the center frequency of about 5.7 GHz of the
type N and type M bursts, we get the electron density
![]() cm-3 (for the second harmonic radiation).
cm-3 (for the second harmonic radiation).
A lower limit of the beam velocity ![]() can be estimated from the requirement
of the deflection time
can be estimated from the requirement
of the deflection time ![]() by Coulomb collisions with the thermal particles.
by Coulomb collisions with the thermal particles.
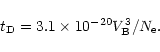 |
(1) |
Under the assumption of the plasma emission excited by the electron beams in a
barometric solar atmosphere, there is a linear relationship between the
frequency drift rate and frequency (Benz et al. 1983); here we take the
temperature
![]() K.
K.
In order for the corona plasma to be magnetically confined,
![]() ,
in this case, under the assumption of
,
in this case, under the assumption of
![]() K and
K and
![]() cm-3, the magnetic
field strength could exceed 26 Gauss.
cm-3, the magnetic
field strength could exceed 26 Gauss.
Using Eq. (5) of Benz et al. (1983):
 |
(2) |
The optical thickness for the fundamental radiation is given by Dulk (1985):
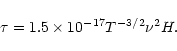 |
(3) |
We discuss the new observations of centimetric type N and type M bursts observed on 27 November 1999. We think they are extension of metric and decimetric type U(N) bursts in centimetric wavelengths. They can be interpreted as the second harmonic plasma emission excited by the same subrelativistic electron beams mirrored near the footpoint. If the reflected beams again travel along open or closed magnetic field lines, we may see a type N or type M burst.
Quasi-periodic particle (electrons) acceleration and injection may be an alternative interpretation of type N and type M bursts, especially when the period of the subsequent type U bursts is similar to the duration of the type U bursts.
As fine structures, type N and type M bursts suggest that there are more complex and smaller magnetic configurations in the lower corona. We believe that some new spectral features of solar microwave bursts will be observed in the future. This is helpful for us to understand the characteristic of the corona magnetic arch, corona condition, and the behavior of electron beams.
Acknowledgements
We would like to thank Dr. Ji Shuchen and Ning Zongjun for helpful discussion. This work is supported by the National NSF of China grant (No. 19833050 and No. 19973016) and Chinese Academy of Sciences.