A&A 365, L231-L236 (2001)
The X-ray spectrum of the supernova remnant 1E 0102.2-7219
A. P. Rasmussen1
-
E. Behar1 -
S. M. Kahn1
-
J. W. den Herder2
-
K. van der Heyden2
Send offprint request: A. P. Rasmussen
1 - Columbia Astrophysics Laboratory, 550 West 120th Street, New York, NY 10027, USA
2 -
University of Utrecht & Space Research Organization of The Netherlands, Sorbonnelaan 2, 3584 CA Utrecht, The Netherlands
Received 4 October 2000 / Accepted 10 November 2000
Abstract
In this letter we present the soft X-ray (5-35 Å) spectrum of
the supernova remnant (SNR) 1E 0102.2-7219 in the Small
Magellanic Cloud, acquired by the reflection grating spectrometers
(RGS) aboard ESA's XMM-Newton Observatory.
Because the RGS features a large dispersion angle, spatial-spectral
confusion is suppressed even for moderately extended
(
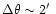 )
sources. Consequently, these data,
along with the spectrum of N132d (Behar et al. #!Behar00!#),
provide what are probably the most detailed soft X-ray spectrum of
entire SNRs. The diagnostic power of performing spectroscopy using
groups of emission lines from single ions is demonstrated. In
particular, the bright Lyman and helium series lines for light
elements (C VI, O VII, O VIII, Ne IX &
Ne X) show peculiar ratios, where the values
)
sources. Consequently, these data,
along with the spectrum of N132d (Behar et al. #!Behar00!#),
provide what are probably the most detailed soft X-ray spectrum of
entire SNRs. The diagnostic power of performing spectroscopy using
groups of emission lines from single ions is demonstrated. In
particular, the bright Lyman and helium series lines for light
elements (C VI, O VII, O VIII, Ne IX &
Ne X) show peculiar ratios, where the values
![${\left[1s-np\right]/\left[1s-(n+1)p\right]}$](/articles/aa/full/2001/01/aaxmm41/img14.gif) are
systematically weaker than expected for electron impact excitation
close to ionization equilibrium, indicating nonequilibrium
ionizing (NEI) conditions in the source.
The well known temperature diagnostics
are
systematically weaker than expected for electron impact excitation
close to ionization equilibrium, indicating nonequilibrium
ionizing (NEI) conditions in the source.
The well known temperature diagnostics
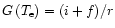 of helium-like triplets
(O VII & Ne IX) confirm this suggestion, with values
that are inconsistent with ionization equilibrium. The temperatures
implied are well above the maximum emission temperature
of helium-like triplets
(O VII & Ne IX) confirm this suggestion, with values
that are inconsistent with ionization equilibrium. The temperatures
implied are well above the maximum emission temperature 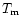 for
each ion, and consistent with a purely ionizing plasma. The density
diagnostics
for
each ion, and consistent with a purely ionizing plasma. The density
diagnostics
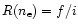 meanwhile, are consistent with the
low density limit, as expected.
meanwhile, are consistent with the
low density limit, as expected.
Key words: atomic processes - line: formation - ISM: individual objects: 1E 0102.2-7219 - galaxies: magellanic clouds
- X-rays: ISM
Author for correspondance: arasmus@astro.columbia.edu
The supernova remnant 1E 0102.2-7219 (E0102) is a bright,
oxygen-rich remnant located in the Small Magellanic Cloud (SMC),
approximately 60 kpc distant. Although it is young ( 1000 years
old) and clearly very energetic, it has no bright emission lines of
Fe nor significant continuum above 5 keV. It has received substantial
attention recently, with the availability of high quality Chandra data (Gaetz et al. 2000; Hughes et al. 2000) as well as Hubble Space
Telescope data (Blair et al. 2000). Its ionization structure in terms of
spatial stratification was clearly seen for the first time with Chandra (Gaetz et al. 2000), and details of the outgoing blast wave's
kinematics and emission spectrum were used to argue that a large
fraction of E0102's energy is spent toward cosmic ray acceleration
(Hughes et al. 2000). It was also observed with the High Energy
Transmission Grating Spectrometer (HETGS) aboard Chandra to
explore the spatially resolved line of sight velocity structures to
isolated emission lines in the spectrum, which help to elucidate the
initial geometry and mass distribution of the initial supernova event,
as well as probe the kinetics of various spectrally resolved
components making up the SNR structure (Flanagan 2000).
1000 years
old) and clearly very energetic, it has no bright emission lines of
Fe nor significant continuum above 5 keV. It has received substantial
attention recently, with the availability of high quality Chandra data (Gaetz et al. 2000; Hughes et al. 2000) as well as Hubble Space
Telescope data (Blair et al. 2000). Its ionization structure in terms of
spatial stratification was clearly seen for the first time with Chandra (Gaetz et al. 2000), and details of the outgoing blast wave's
kinematics and emission spectrum were used to argue that a large
fraction of E0102's energy is spent toward cosmic ray acceleration
(Hughes et al. 2000). It was also observed with the High Energy
Transmission Grating Spectrometer (HETGS) aboard Chandra to
explore the spatially resolved line of sight velocity structures to
isolated emission lines in the spectrum, which help to elucidate the
initial geometry and mass distribution of the initial supernova event,
as well as probe the kinetics of various spectrally resolved
components making up the SNR structure (Flanagan 2000).
In our work, we concentrate on the high resolution X-ray spectroscopy
afforded by the RGS (den Herder et al. 2001) aboard XMM-Newton
(Jansen et al. 2001), which has nearly nominal spectral
resolution, even for moderately extended objects such as E0102.
E0102 was observed early in XMM-Newton's
Calibration phase, during revolution 0065 (16 April, 2000) for a total
of 42.8 ksec. All three EPIC (Turner et al. 2001, Struder et al. 2001) instruments and
both RGS (den Herder et al. 2001) instruments were operated
simultaneously. Here, our primary focus is on the high resolution
X-ray spectrum of E0102, and we restrict attention to the RGS
data![[*]](/icons/foot_motif.gif) .
.
The data were processed using custom software, originally developed for
the analysis of RGS ground calibration data, which is nearly identical
in function to the RGS branch of the Science Analysis System
(SAS). Telemetered CCD events were read in, frame by frame for each CCD
node, and were offset corrected on a pixel by pixel basis using
median readout maps, compiled from about 40 DIAGNOSTIC
(den Herder et al. 2001) images per CCD chip. This process nearly eliminates
flickering pixels from the dataset. Gain and CTI corrections were
performed to align the signal/energy scale across all CCD
readouts. Then, event reconstruction was performed on connected
pixels containing significant signal, and the composite event signals
were calculated by summing up signals from individual pixels.
The standard event grade combinations used were those which fall within
a
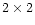 pixel region, where two pixels diagonally opposed to
one another within the
pixel region, where two pixels diagonally opposed to
one another within the
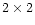 were not grouped into composites.
The event coordinates were then mapped into focal plane
angular coordinates (dispersion and
cross-dispersion)
were not grouped into composites.
The event coordinates were then mapped into focal plane
angular coordinates (dispersion and
cross-dispersion)![[*]](/icons/foot_motif.gif) . Using the star tracker attitude history updates
for the revolution, the coordinates of the source, and a preliminary
boresight axis, aspect corrections were applied to the focalplane event
angular coordinates
. Using the star tracker attitude history updates
for the revolution, the coordinates of the source, and a preliminary
boresight axis, aspect corrections were applied to the focalplane event
angular coordinates![[*]](/icons/foot_motif.gif) .
.
Events were extracted in the dispersion-pulseheight
plane with masks utilized by the response matrix
generator. Surviving events were then windowed in the focal plane,
where background subtraction was performed using background sampling
regions off to one side of the illumination pattern in the
cross-dispersion direction![[*]](/icons/foot_motif.gif) . The size
of the spatial extraction was 58
. The size
of the spatial extraction was 58
 in the
cross-dispersion direction, which comfortably includes the entire
spectrum. A spectrum file was created by computing the
background corrected countrates in each dispersion channel. Finally, a
response generator was used to produce an observation specific
response matrix that provided an array of nominal wavelength/energy values
corresponding to each channel in the spectrum file. Line fluxes were
estimated by comparing an emission model, folded through the response
matrix, directly to the spectrum in the usual manner. This procedure
was performed for first (m=-1) and second (m=-2) orders for each
spectrometer.
in the
cross-dispersion direction, which comfortably includes the entire
spectrum. A spectrum file was created by computing the
background corrected countrates in each dispersion channel. Finally, a
response generator was used to produce an observation specific
response matrix that provided an array of nominal wavelength/energy values
corresponding to each channel in the spectrum file. Line fluxes were
estimated by comparing an emission model, folded through the response
matrix, directly to the spectrum in the usual manner. This procedure
was performed for first (m=-1) and second (m=-2) orders for each
spectrometer.
To reduce the contamination from sporadic background rate fluctuations
in which the RGS counting rate doubles from the
quiescent rate of about
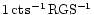 ,
a set of
good time intervals (GTIs) were chosen to filter out the high
background data. This reduced the effective exposure time from
37.9 to 29.7 ksec for both RGS instruments.
,
a set of
good time intervals (GTIs) were chosen to filter out the high
background data. This reduced the effective exposure time from
37.9 to 29.7 ksec for both RGS instruments.
The windowed background contribution to the (m=-1) total
countrate for this extraction was nearly flat, at
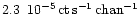 or, equivalently,
or, equivalently,
 at 15 Å
at 15 Å ![[*]](/icons/foot_motif.gif) .
.
Using the analysis procedure described above, we generated the RGS
spectra shown
in Figs. 1 and
2.
![\begin{figure}
\par\includegraphics[angle=-90,width=8.8cm,clip]{xmm41_f1.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg25.gif) |
Figure 1:
The first order (m=-1) RGS spectrum of
E0102. The data obtained from the two RGS instruments
are plotted separately (RGS1 in black, RGS2 in red). The 20-24 Å gap
in the RGS2 spectrum is due to failed electronics for CCD 4 in that
instrument. For clarity, the data are provided in both linear and
logarithmic scales. Identifications of the principle lines are
provided |
| Open with DEXTER |
![\begin{figure}
\par\includegraphics[angle=-90,width=18cm,clip]{xmm41_f2.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg26.gif) |
Figure 2:
Detail of the 8-20 Å region of the RGS data. First
and second order extractions are plotted separately (m=-1 in black,
m=-2 in red) to facilitate line identification. The data from the two
spectrometers have been averaged for each order extraction. The higher
spectral resolution and resilience to source extent in is clearly seen
in m=-2, where some line complexes blended in m=-1 are resolved |
| Open with DEXTER |
The spectrum is dominated
by a small number of emission lines, the brightest of which are
prominent transitions of highly stripped light ions of C, O, Ne and
Mg. Nitrogen, which is cosmically less abundant than oxygen by a
factor of a few, is absent in spectrum (N VII
 is
expected at 24.7 Å).
Only very weak Fe L lines are detected, which are dwarfed by
the strong transitions of the light, even Z elements.
We detect bright lines from the Lyman series of C VI,
O VIII, Ne X and Mg XII, and from
the helium series of O VII, Ne IX, Mg XI and
Si XIII. Most of these ions are represented by several
emission lines in the spectrum, clearly resolved and measurable in
this sparse spectrum. Single ion spectroscopy permits
independent measurements of astrophysical quantities that affect line
production, such as electron temperature and density, or opacity
within the object and in other intervening material.
is
expected at 24.7 Å).
Only very weak Fe L lines are detected, which are dwarfed by
the strong transitions of the light, even Z elements.
We detect bright lines from the Lyman series of C VI,
O VIII, Ne X and Mg XII, and from
the helium series of O VII, Ne IX, Mg XI and
Si XIII. Most of these ions are represented by several
emission lines in the spectrum, clearly resolved and measurable in
this sparse spectrum. Single ion spectroscopy permits
independent measurements of astrophysical quantities that affect line
production, such as electron temperature and density, or opacity
within the object and in other intervening material.
To synthesize the appropriate RGS response for E0102,
we need to quantify the angular distribution of the X-ray emission,
which is convolved with the other quantities contributing to the
line spread function. As a preliminary approximation, we used a
public Chandra image (ObsIds 1231 & 1423) as an energy
independent distribution, characterized by  20
20
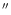 half power
width (HPW) and
half power
width (HPW) and  33
33
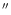 FWHM. The
image was collapsed along the dispersion axis according to the roll of
XMM-Newton during the observation. This one dimensional distribution
was used as input to the response generator. Comparing the
prediction to the brightest, isolated emission line
(O VIII Ly
FWHM. The
image was collapsed along the dispersion axis according to the roll of
XMM-Newton during the observation. This one dimensional distribution
was used as input to the response generator. Comparing the
prediction to the brightest, isolated emission line
(O VIII Ly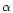 ), we see that the prediction is far
narrower than the data, indicating a velocity distribution resolved by
the RGS (see Fig. 3).
), we see that the prediction is far
narrower than the data, indicating a velocity distribution resolved by
the RGS (see Fig. 3).
![\begin{figure}
\par\includegraphics[width=5.5cm,clip]{xmm41_f3.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg28.gif) |
Figure 3:
Illustration of the line profile
used for estimating line fluxes. The plot compares the point source
line spread function for RGS1, the approximate monochromatic line
profile based on E0102's angular distribution (derived from the
Chandra image), and an heuristic wavelength broadening template
function that is applied in addition to the angular distribution |
| Open with DEXTER |
Further accurate modeling
requires coupling a line of sight velocity distribution to the angular
distribution, but for the time being we are able to place a lower
limit to the ejecta's expansion,
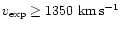 ,
using the square velocity profile model of
an expanding shell. This lower limit is consistent with the broadening
seen in all of the lines. Any contribution from a high velocity
(
,
using the square velocity profile model of
an expanding shell. This lower limit is consistent with the broadening
seen in all of the lines. Any contribution from a high velocity
(
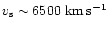 )
blast wave is difficult
to see in this profile because it accounts for only
)
blast wave is difficult
to see in this profile because it accounts for only  10% of
the light (Hughes et al. 2000) and is coincident with the broad scatter
distribution off of the gratings.
10% of
the light (Hughes et al. 2000) and is coincident with the broad scatter
distribution off of the gratings.
Spectral modeling was performed by applying this velocity distribution
to all lines. While there is already evidence from Chandra that
different velocity distributions are present for different charge
states (Flanagan 2000), we use this distribution for the
purpose of estimating line fluxes. Estimates of the line fluxes are
provided in Table 1, according to the current
response matrices.
Table 1:
Estimates of line fluxes at the telescope
aperture. Wherever possible, line fluxes were determined using the two
spectrometers separately and the 90% confidence limits
are also listed. For each emission line or complex, the RGS1
fluxes are given in the first row, followed by the RGS2 derived fluxes.
Systematic differences between the spectrometers may be seen
by the discrepancies in derived flux values. Because the calibration
of the RGS instruments is ongoing, these flux values are uncertain
by
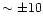 %.
Also, the absolute flux
in the O VII K
%.
Also, the absolute flux
in the O VII K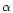 is systematically underestimated by
about 20%, due to the presence of an instrumental oxygen absorption
edge nearby. The largest discrepancy seen (
is systematically underestimated by
about 20%, due to the presence of an instrumental oxygen absorption
edge nearby. The largest discrepancy seen ( 30%) is in the flux
values for the Mg XI, is about twice as large as expected.
The top part of the table gives the total line intensities in the
K
30%) is in the flux
values for the Mg XI, is about twice as large as expected.
The top part of the table gives the total line intensities in the
K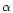 ,
K
,
K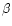 and K
and K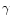 transitions for each ion; the bottom
part gives the breakdown between the resonance (r),
intercombination (i) and forbidden (f) transitions for the helium like
species O VII, Ne IX and Mg XI. All fluxes are in
units of
transitions for each ion; the bottom
part gives the breakdown between the resonance (r),
intercombination (i) and forbidden (f) transitions for the helium like
species O VII, Ne IX and Mg XI. All fluxes are in
units of

| Ion |
Transition |
| |
K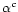 |
K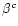 |
K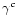 |
| |
|
|
|
| C VI |
0.94+.38-.16 |
0.69+.17-.11 |
NA |
| |
1.01+.20-.20 |
0.52+.13-.13 |
NA |
| O VIIa |
30.0+.66-.68 |
4.12+.30-.30 |
2.02+.19-.19 |
| |
NA |
4.88+.30-.30 |
2.17+.18-.17 |
| O VIII |
29.8+.68-.68 |
6.70+.31-.32 |
3.07+.21-.22 |
| |
32.8+.65-.65 |
7.39+.30-.30 |
3.49+.21-.21 |
| Ne IX |
20.4+.63-.70 |
2.44+.21-.21 |
1.16+.16-.15 |
| |
21.2+.54-.53 |
2.63+.22-.22 |
1.68+.17-.17 |
| Ne X |
12.5+.43-.43 |
3.04+.27-.27 |
0.87+.23-.13 |
| |
14.3+.46-.45 |
2.44+.21-.21 |
1.08+.09-.23 |
| Mg XIb |
5.28+.31-.37 |
NA |
NA |
| |
3.52+.26-.26 |
NA |
NA |
| Mg XII |
1.29+.26-.21 |
NA |
NA |
| |
1.22+.17-.17 |
NA |
NA |
|
He K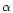 (r) (r) |
He K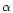 (i) (i) |
He K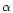 (f) (f) |
| |
|
|
|
| O VIIa |
19.5+.66-.69 |
2.48+.41-.39 |
8.00+.40-.42 |
| |
NA |
NA |
NA |
| Ne IX |
13.8+.63-.70 |
1.12+.59-.54 |
5.48+.44-.59 |
| |
13.6+.54-.53 |
1.54+.49-.49 |
6.08+.36-.35 |
| Mg XIb |
3.84+.31-.37 |
0+.48-0 |
1.44+.29-.26 |
| |
2.78+.26-.26 |
0+.38-0 |
0.74+.19-.18 |
a O VII K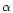 fluxes are underestimated here by about 20%.
fluxes are underestimated here by about 20%.
b Discrepancy in Mg XI K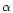 fluxes is currently not understood.
fluxes is currently not understood.
c K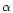 ,
K
,
K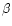 and K
and K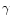 represent the 1-2, 1-3 and
1-4 transitions, respectively.
represent the 1-2, 1-3 and
1-4 transitions, respectively.
As indicated below, there is a known instrumental
oxygen feature not included in these response matrices that we treat
separately. The instrumental response and effective area are described
briefly in a separate article (den Herder et al. 2001).
We attempt to correct, at least partially, for effects of
intervening absorption and the known oxygen edge referred to above.
Figure 4 shows the
correction function utilized to estimate line fluxes from the
source corrected for these effects.
![\begin{figure}
\includegraphics[angle=-90,width=8.8cm,clip]{xmm41_f4a.eps} \inc...
...{0.5mm}
\includegraphics[angle=-90,width=4.2cm,clip]{xmm41_f4c.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg34.gif) |
Figure 4:
Estimated resonance line ratios emitted at the
source. Line emission ratios for individual ions are calculated by
correcting the values in Table 1 according to the
Galactic absorption and the additional instrumental oxygen absorption
edge not implemented in the current response matrices. The correction
function used is shown in the upper plot.
Estimated flux ratios are given in the lower two plots, with the
confidence limits associated with them for each RGS. The expected
dependences of these ratios on electron temperature  are
provided in the curves. We used the APEC (Smith et al. 1999) CIE
model to produce these, so we expect slightly different temperature
dependences for NEI cases.
The lower left plot shows the ratios of
are
provided in the curves. We used the APEC (Smith et al. 1999) CIE
model to produce these, so we expect slightly different temperature
dependences for NEI cases.
The lower left plot shows the ratios of
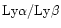 (C VI, O VIII & Ne X) and (C VI, O VIII & Ne X) and
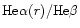 (O VII & Ne IX); the lower right plot
gives
(O VII & Ne IX); the lower right plot
gives
 (O VIII & Ne X) and
(O VIII & Ne X) and
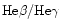 (O VII & Ne IX)
(O VII & Ne IX) |
| Open with DEXTER |
We adopted a neutral Galactic
column of
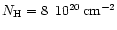 from other
estimates toward the SMC (e.g., Blair et al. 2000), and also an
instrumental oxygen photoelectric absorption profile characterized by
from other
estimates toward the SMC (e.g., Blair et al. 2000), and also an
instrumental oxygen photoelectric absorption profile characterized by
 .
The curve was used to correct the nominal
line fluxes listed in Table 1 and emission line
ratios for individual ions were estimated. These are displayed in the
lower two plots of Fig. 4.
.
The curve was used to correct the nominal
line fluxes listed in Table 1 and emission line
ratios for individual ions were estimated. These are displayed in the
lower two plots of Fig. 4.
The estimated emitted line ratios have not been corrected for any
additional intervening absorption, e.g., contributions from the
SMC. Any such column density would systematically affect the line
ratios at long wavelength, which may be reflected by the low value
for the C VI
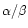 ratio. As shown in the
figure, the predicted intraseries ratios
ratio. As shown in the
figure, the predicted intraseries ratios
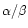 and
and
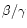 for heliumlike and hydrogenlike ions are dependent on
for heliumlike and hydrogenlike ions are dependent on
 ,
but approach their minimum values in the limit of high
temperature. Our ratio estimates, compared to the current APEC
(Smith et al. 1999) collisional ionization equilibrium (CIE) emission
model, suggest electron temperatures
,
but approach their minimum values in the limit of high
temperature. Our ratio estimates, compared to the current APEC
(Smith et al. 1999) collisional ionization equilibrium (CIE) emission
model, suggest electron temperatures  that are
significantly higher than the maximum line emission temperatures
that are
significantly higher than the maximum line emission temperatures
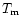 in CIE. Consistent temperature estimates are obtained
for Ne IX line ratios, which yield
in CIE. Consistent temperature estimates are obtained
for Ne IX line ratios, which yield
 .
In other cases a self consistent
.
In other cases a self consistent  cannot be obtained in
the APEC CIE model, given the estimated emission ratios. For example,
O VII, derived
cannot be obtained in
the APEC CIE model, given the estimated emission ratios. For example,
O VII, derived
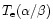 and
and
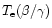 values are inconsistent by a factor of
2.5 or so. For O VIII, the APEC curves do not intersect the
line ratio confidence limits.
values are inconsistent by a factor of
2.5 or so. For O VIII, the APEC curves do not intersect the
line ratio confidence limits.
We performed sample line ratio calculations using HULLAC
(Bar-Shalom et al. 1998) in which the plasma is underionized
as compared to CIE, in order to simulate the purely
ionizing case. These ratios were within  10% of the APEC ratios
for the O VIII case for both
10% of the APEC ratios
for the O VIII case for both
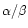 and
and
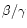 .
For O VII however, the HULLAC line ratios
deviate from the APEC CIE values and assymptotically approach 4.8 and
2.5 for the
.
For O VII however, the HULLAC line ratios
deviate from the APEC CIE values and assymptotically approach 4.8 and
2.5 for the
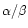 and
and
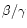 ratios, respectively.
Comparing the O VII line ratios to the HULLAC values, we
obtain a self consistent temperature range of
ratios, respectively.
Comparing the O VII line ratios to the HULLAC values, we
obtain a self consistent temperature range of
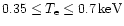 .
Interestingly, the line ratios for O VIII remain low as
compared to either the CIE (APEC) or the ionizing (HULLAC) cases.
.
Interestingly, the line ratios for O VIII remain low as
compared to either the CIE (APEC) or the ionizing (HULLAC) cases.
In spite of the angular extent of the source and the velocity
broadening of the lines, we resolve the He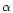 line complexes of
O VII and Ne IX sufficiently to estimate contributions
from the forbidden, intercombination and resonance transitions that
provide robust diagnostics for the temperature and density of the
emitting medium (Gabriel & Jordan 1969; Pradhan 1982). Some
contamination from satellite line blending is present in the flux
estimates of the intercombination (i) and forbidden (f) lines, but
only at the 3 and 6% level for O VII and Ne IX,
respectively (Pradhan & Shull 1981). These effects are smaller or
comparable to the line flux confidence limits and are also taken into
consideration in diagnostic line ratio calculations
(Pradhan 1982). Figure 5 shows the
heliumlike triplets of O VII and Ne IX, modelled using
the known line wavelengths, and the line velocity profile adopted
above (Fig. 3).
line complexes of
O VII and Ne IX sufficiently to estimate contributions
from the forbidden, intercombination and resonance transitions that
provide robust diagnostics for the temperature and density of the
emitting medium (Gabriel & Jordan 1969; Pradhan 1982). Some
contamination from satellite line blending is present in the flux
estimates of the intercombination (i) and forbidden (f) lines, but
only at the 3 and 6% level for O VII and Ne IX,
respectively (Pradhan & Shull 1981). These effects are smaller or
comparable to the line flux confidence limits and are also taken into
consideration in diagnostic line ratio calculations
(Pradhan 1982). Figure 5 shows the
heliumlike triplets of O VII and Ne IX, modelled using
the known line wavelengths, and the line velocity profile adopted
above (Fig. 3).
![\begin{figure}
\includegraphics[width=4.2cm,clip]{xmm41_f5a.eps}\hspace*{0.5mm}
\includegraphics[width=4.cm,clip]{xmm41_f5b.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg43.gif) |
Figure 5:
Derivation of the R (density) and G (temperature) line
ratio diagnostics for O VII (left) and Ne IX (right).
The data were fit using three line
features, where we have used the heuristic velocity distribution
function derived from O VIII Ly 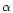 as a template to
describe each (see Fig. 3). One parameter
adjusted the ensemble line position (relative line positions were
fixed), and the three line intensities were allowed to vary to fit the
data. Individual contributions from the f, i and r lines are
shown. The
as a template to
describe each (see Fig. 3). One parameter
adjusted the ensemble line position (relative line positions were
fixed), and the three line intensities were allowed to vary to fit the
data. Individual contributions from the f, i and r lines are
shown. The
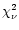 values are 1.4 and 0.9 for the O VII
and Ne IX triplets, respectively
values are 1.4 and 0.9 for the O VII
and Ne IX triplets, respectively |
| Open with DEXTER |
While the density diagnostic R
ratios obtained were consistent with the low density
limit at the 90% confidence level, the temperature diagnostic Gvalues are low. We measure at
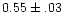 and
and
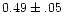 for
O VII and Ne IX, respectively. It should be noted here
that these small values for G are inconsistent with collisional
equilibrium, and require underionization,
strongly suppressing photons produced in recombination channels
from O VIII and Ne IX (Pradhan 1982).
The electron temperatures corresponding to these ratios are
approximately 0.35 and 0.65 keV for O VII and Ne IX
respectively, in agreement with the 90% confidence limits derived
from the resonance line ratio estimates above.
In CIE conditions for these temperatures, the fractional population of
these charge states whould be only
for
O VII and Ne IX, respectively. It should be noted here
that these small values for G are inconsistent with collisional
equilibrium, and require underionization,
strongly suppressing photons produced in recombination channels
from O VIII and Ne IX (Pradhan 1982).
The electron temperatures corresponding to these ratios are
approximately 0.35 and 0.65 keV for O VII and Ne IX
respectively, in agreement with the 90% confidence limits derived
from the resonance line ratio estimates above.
In CIE conditions for these temperatures, the fractional population of
these charge states whould be only  2% for both O VII and
Ne IX (Arnaud & Rothenflug 1985).
The fact that consistent values for
2% for both O VII and
Ne IX (Arnaud & Rothenflug 1985).
The fact that consistent values for  are obtained for
both O VII and Ne IX using all three available
diagnostics, suggest that K
are obtained for
both O VII and Ne IX using all three available
diagnostics, suggest that K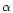 resonance line suppression
effects (such as absorption or resonant scattering) are small.
resonance line suppression
effects (such as absorption or resonant scattering) are small.
The Fe L lines that we do see in the SNR most likely trace the
swept up ISM rather than the ejecta of the precursor. The Fe:O
abundance ratio that we measure is about 1:900, compared to 1:15 or so
in the SMC (Russell & Dopita 1992). With the exception of Fe, the
composition of the SNR is dominated by products of 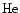 burning, the SN ejecta. The absence of emission from
burning, the SN ejecta. The absence of emission from  is
suggestive that any nitrogen in the progenitor had been either blown
off or burned into other products.
is
suggestive that any nitrogen in the progenitor had been either blown
off or burned into other products.
Analysis of the heliumlike ion spectra for O VII and
Ne IX yields self consistent pictures for these plasmas:
There exist temperatures which simultaneously yield the line ratios
seen (G,
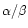 &
&
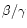 )
provided that the
neighboring charge states (O VIII & Ne X) are
underpopulated there. The presence of bright emission lines from the
hydrogenlike ions must then indicate a different volume containing
them. This is in fact consistent with the Chandra findings
(Gaetz et al. 2000; Flanagan 2000) that emission from
O VII and O VIII appear to originate from different
regions within the SNR. In the Chandra HETGS
"spectroheliograms'', isolated line images show that the bright
O VII emission occurs significantly inside of the region
emitting in O VIII. The inner, O VII region is
presumably ejecta that has more recently encountered the inward moving
shock than the outer O VIII region.
)
provided that the
neighboring charge states (O VIII & Ne X) are
underpopulated there. The presence of bright emission lines from the
hydrogenlike ions must then indicate a different volume containing
them. This is in fact consistent with the Chandra findings
(Gaetz et al. 2000; Flanagan 2000) that emission from
O VII and O VIII appear to originate from different
regions within the SNR. In the Chandra HETGS
"spectroheliograms'', isolated line images show that the bright
O VII emission occurs significantly inside of the region
emitting in O VIII. The inner, O VII region is
presumably ejecta that has more recently encountered the inward moving
shock than the outer O VIII region.
The emission line pattern of the O VIII Lyman series clearly
requires better understanding; these ratios are better constrained and
are consistent across RGS instruments, yet they are inconsistent with
current electron impact excitation (EIE) models. Opacity effects to
the Ly 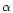 flux should be negligible because of the consistency
found in the O VII and Ne IX He series data.
flux should be negligible because of the consistency
found in the O VII and Ne IX He series data.
We consider charge exchange (CE) as a possible mechanism that might
contribute to produce the observed flux ratios.
The emission spectral signature associated
with a CE reaction generally resembles that of recombination
(Wise & Sarazin 1989), because in both mechanisms, an electron is injected
into a high quantum level and then cascades. The flux ratios due to
pure recombination of O IX to O VIII are about 4.7 and
2.6 for
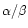 and
and
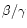 ,
respectively (using
HULLAC). The same ratios for EIE in the high temperature limit, are
6.2 and 3.0. Weighting the contributions from CE and EIE
appropriately, it may be possible to produce the observed values of
,
respectively (using
HULLAC). The same ratios for EIE in the high temperature limit, are
6.2 and 3.0. Weighting the contributions from CE and EIE
appropriately, it may be possible to produce the observed values of
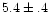 and
and 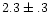 ,
respectively.
We cannot preclude the presence of CE reactions involving
O VIII in the emission line ratios of O VII,
although the emission there appears generally consistent with extreme
NEI conditions.
,
respectively.
We cannot preclude the presence of CE reactions involving
O VIII in the emission line ratios of O VII,
although the emission there appears generally consistent with extreme
NEI conditions.
In CE reactions, the exchanged electrons are injected into specific
high (n,l) levels according to energy conservation.
Spectra from such reactions have been observed extensively
in tokamak and ion beam experiments, for example, in highly ionized
 (Rice et al. 1986) and
(Rice et al. 1986) and
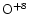 (Bliman et al. 1985). In the latter work, CE reactions of
(Bliman et al. 1985). In the latter work, CE reactions of
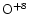 with molecular
with molecular 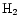 yielded values of
yielded values of
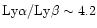 and
and
 (40% relative error in each) and a preferential injection into
n=5. Calculations performed for interactions with atomic hydrogen
yield values with comparable variances, but all well below ratios
predicted by EIE.
(40% relative error in each) and a preferential injection into
n=5. Calculations performed for interactions with atomic hydrogen
yield values with comparable variances, but all well below ratios
predicted by EIE.
In order for CE to contribute significantly to the X-ray line
emission from a SNR, low ionization species must come into close
proximity with more heavily ionized metals (such as
O IX). Given that the CE and EIE rate coefficients are of order
 and
and
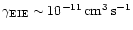 ,
respectively, CE can be
important if the neutral fraction for hydrogen is greater than
,
respectively, CE can be
important if the neutral fraction for hydrogen is greater than
 10-5 or so (Wise & Sarazin 1989). This is well above the neutral
fraction expected for the hot ionized plasma where the X-ray emission
is thought to originate. In the case of E0102, the amounts of H and
He present in the ejecta are probably very low, so the metal ion to
electron ratio should be extremely high as compared to solar abundance
material. Consequently, CE is more likely to be important in such a plasma,
particularly if inhomogeneities are present and mixing occurs between
regions.
10-5 or so (Wise & Sarazin 1989). This is well above the neutral
fraction expected for the hot ionized plasma where the X-ray emission
is thought to originate. In the case of E0102, the amounts of H and
He present in the ejecta are probably very low, so the metal ion to
electron ratio should be extremely high as compared to solar abundance
material. Consequently, CE is more likely to be important in such a plasma,
particularly if inhomogeneities are present and mixing occurs between
regions.
There is abundant evidence that the X-ray emitting media in
SNRs are far from homogeneous. For example, [O III] emission is
seen in many SNRs, including E0102 (Dopita et al. 1981) and some of the
bright knots seen there correspond well to X-ray bright features
(Gaetz et al. 2000). Efforts to numerically model shock-cloud
interactions produce strong fragmentation, turbulent mixing action and
complex filamentary structures (e.g., Xu & Stone 1995). These general
predictions should apply also to interactions between ejecta clumps
and the reverse shock. The material in E0102 may be comprised
primarily of metals, and cooling rates there can be
enormous, so radiative shock conditions may exist even at high
 .
The survivability of ejecta clumps and filaments
embedded in strong shocks would consequently improve, so interfaces
between hot, tenuous media and warm, denser filaments could be
prevalent.
.
The survivability of ejecta clumps and filaments
embedded in strong shocks would consequently improve, so interfaces
between hot, tenuous media and warm, denser filaments could be
prevalent.
At these interfaces, one expects a steady flux of high charge state
ions from the hot medium. These ions are likely to charge exchange
with the lower ionization states in the clouds, giving rise to X-ray
emission lines. Since the charge exchange cross-section for the ions
is considerably larger than the photoelectric absorption cross-section
for the emitted line photons, the emitted line radiation should emerge
and contribute to the observed X-ray spectrum of the remnant. This
process should be important if the mean intercloud separation is
smaller than the characteristic distance d an ion will move through
the hot gas before it is collisionally excited:
 |
(1) |
The Chandra image of E0102 (Gaetz et al. 2000) exhibits significant
structure on spatial scales comparable to or less than this value. It
therefore seems plausible that charge exchange at cloud interfaces plays
a significant role in producing the anomalous line ratios that we
observe in O VIII.
Acknowledgements
This work is based on observations obtained with XMM-Newton, an
ESA science mission with instruments and contributions directly
funded by ESA Member States and the USA (NASA). We thank our
referree, T. J. Gaetz, for insightful comments and suggestions that
substantially improved this manuscript. We also thank D. W. Savin for
useful discussions on charge transfer processes and resulting emission.
-
Arnaud, M., & Rothenflug, R. 1985, A&AS, 60, 425
In the text
NASA ADS
-
Bar-Shalom, A., Klapisch, M., Goldstein, W. H., & Oreg, J. 1998, unpublished
In the text
-
Behar, E., Rasmussen, A. P., Griffiths, R. G., et al. 2001, A&A, 365, L242
-
Blair, W. P., Morse, J. A., Raymond, J. C., et al. 2000, ApJ, 537, 667
In the text
NASA ADS
-
Bliman, S., Bonnet, J. J., Montenesquieu, A. B., et al. 1985, Nucl. Instrum. Meth. Phys. Res., Sect. B, 9,
371
In the text
-
den Herder, J. W., Brinkman, A. C., Kahn, S. M., et al. 2001, A&A, 365, L7
In the text
-
Dopita, M. A., Tuohy, I. R., & Matthewson, D. S. 1981, ApJL, 248, L105
In the text
NASA ADS
-
Flanagan, K. 2000, in X-Ray Astronomy 2000, ed. R. Giacconi, L. Stella, &
S. Serio, Publications of the Astronomical Society of the Pacific Conference
Series (PASP)
In the text
-
Gabriel, A. H. & Jordan, C. 1969, MNRAS, 145, 241
In the text
NASA ADS
-
Gaetz, T. J., Butt, Y. M., Edgar, R. J., et al. 2000, ApJ, 534, L47
In the text
NASA ADS
-
Hughes, J. P., Rakowski, C. E., & Decourchelle, A. 2000, ApJ, in press
In the text
-
Jansen, F., Lumb, D., Altieri, B., et al. 2001, A&A, 365, L1
In the text
-
Pradhan, A. K. 1982, ApJ, 263, 477
In the text
NASA ADS
-
Pradhan, A. K., & Shull, J. M. 1981, ApJ, 249, 821
In the text
NASA ADS
-
Rice, J. E., Marmar, E. S., Terry, J. L., Kallne, E., & Kallne, J. 1986, Phys.
Rev. Lett., 56, 50
In the text
NASA ADS
-
Russell, S. C. & Dopita, M. A. 1992, ApJ, 384, 508
In the text
NASA ADS
-
Sasaki, M., Stadlbauer, T. F. X., Haberl, F., Filipovic, M. D., & Bennie,
P. J. 2000, A&A, 365, L237
-
Smith, R. K., Brickhouse, N., Raymond, J., & Liedahl, D. 1999, unpublished,
version 1.0.1 available at http://hea-www.harvard.edu/APEC.
In the text
-
Strüder, L., Briel, U. G., Dennerl, K., et al. 2001, A&A, 365, L18
In the text
-
Turner, M. J., Abbey, A., Arnaud, M., et al. 2001, A&A, 365, L27
In the text
-
Wise, M. W., & Sarazin, C. L. 1989, ApJ, 345, 384
In the text
NASA ADS
- Xu, J., & Stone, J. M. 1995, ApJ, 454, 172
In the text
NASA ADS
© ESO 2001
![\begin{figure}
\par\includegraphics[angle=-90,width=8.8cm,clip]{xmm41_f1.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg25.gif)
![\begin{figure}
\par\includegraphics[angle=-90,width=18cm,clip]{xmm41_f2.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg26.gif)
![\begin{figure}
\par\includegraphics[width=5.5cm,clip]{xmm41_f3.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg28.gif)
![\begin{figure}
\includegraphics[angle=-90,width=8.8cm,clip]{xmm41_f4a.eps} \inc...
...{0.5mm}
\includegraphics[angle=-90,width=4.2cm,clip]{xmm41_f4c.eps}\end{figure}](/articles/aa/full/2001/01/aaxmm41/Timg34.gif)
