A&A 403, 725-730 (2003)
DOI: 10.1051/0004-6361:20030400
Variability of EUV-spectra from the quiet upper solar atmosphere: Intensity and Doppler shift
A. Brkovic1,3 - H. Peter1 - S. K. Solanki2
1 - Kiepenheuer-Institut für Sonnenphysik, Schöneckstr. 6, 79104
Freiburg, Germany
2 -
Max-Planck-Institut für Aeronomie, Max-Planck-Str. 2, 37191
Katlenburg-Lindau, Germany
3 -
Institute of Astronomy, ETH-Zentrum, 8092 Zürich, Switzerland
Received 15 May 2002 / Accepted 11 March 2003
Abstract
We have studied SUMER and CDS time series of spectra and
images of quiet-Sun regions at the solar disc centre. The data contain
ultraviolet emission lines sampling temperatures of the chromosphere,
transition region and corona. We find a high correlation between average
net Doppler shifts and relative brightness variabilities of the studied
lines (correlation coefficient of 0.92), suggesting a connection between
the two quantities. The anti-correlation between differential
emission measures and relative brightness variabilities is weaker
(correlation coefficient of -0.78).
We discuss the observed relationships on the basis of differential
emission measures and linear wave calculations.
Key words: Sun: chromosphere - Sun: corona - Sun:
transition region - Sun: UV radiation
1 Introduction
Recent EUV observations have revealed that transition region lines are on
average redshifted, while the coronal lines are blueshifted (Peter & Judge
1999; Teriaca et al. 1999; and references therein). Models
proposed to explain these shifts include siphon flows through loops,
explosive events, waves due to nano-flares or return of spicular material
(Antiochos 1984;
McClymont & Craig 1987;
Mariska 1988;
Hansteen 1993;
Spadaro et al. 1996;
for a discussion see Peter & Judge 1999).
Early studies of the correlation between Doppler shifts and line
intensities in the quiet Sun gave inconsistent results. Athay et al.
(1983) and Dere et al. (1984) found no correlation between
intensity and velocity in the data covering the C IV 1548 Å transition
region line. Gebbie et al. (1981) analysed spectra of the C II
1336 Å, Si IV 1393 Å and C IV 1548 Å lines and found that
redshifted regions were correlated with regions of bright network emission
and blueshifted regions tend to be associated with darker areas. More
recently, Stucki et al. (2000) and Hansteen et al.
(2000) both obtained a positive correlation between network
emission and redshift of transition region lines in the quiet Sun. In
addition, Stucki et al. (2000) also showed that in coronal holes,
the sign of the correlation is reversed. Such a correlation supports the
model of Hansteen (1993)
who proposed that nano-flares
occurring at the top of coronal loops generate MHD waves that propagate
downward along the magnetic fields towards and through the transition
region in their footpoints, which lie in the network. The net redshifts
in TR spectral lines are a result of the correlation between the intensity
and velocity that occurs in downward propagating acoustic waves.
Studies of line intensity variability (e.g., Rabin & Dowdy 1992; Harrison
1997; Krucker et al. 1997; Brkovic et al. 2000) as well as of line
shifts are numerously represented in the literature, the latter both on
theoretical and observational basis (see above). Although both quantities
have been related to other parameters, e.g., line formation temperature,
intensity (at a given spatial location) and line width, the two quantities
have never so far, to our knowledge, been compared with each other for a
group of spectral lines. This work tries to establish the connection
between spatial averages of relative changes in line intensities and
of net Doppler shifts. The former are observed in the quiet Sun using time
series of spectra recorded by SUMER and movies obtained with CDS. The latter
are taken from the literature. In addition we analyse the relationship
between differential emission measures and relative intensity variabilities.
After the description of the observations in Sect. 2 we describe
in Sect. 3 our results concerning the time variability of line
intensities and Doppler shifts and the relation to the differential
emission measure and the intensity distribution. These results are
discussed in Sect. 4 with respect to selection effects due to the
emission measure distribution, and a simple model to understand the close
relation between Doppler shift and intensity fluctuations in terms of linear
waves are described. Before concluding the paper some remarks are
made on the structure of the transition region.
2 Observations
For the evaluation of the variabilities in both intensity and Doppler
shift as well as differential emission measures quiet regions at Sun
centre have been observed using the Solar Ultraviolet Measurements of
Emitted Radiation (SUMER) spectrometer (Wilhelm et al. 1995)
and the Normal Incidence Spectrometer (NIS) of the Coronal Diagnostic
Spectrometer (CDS, Harrison et al. 1995) onboard the SOHO
spacecraft. A list of the lines analysed and temperatures of line
formation are given in Table 1. The Mg IX 368.1 Å line was observed
only with CDS, He I 584.3 Å and O V 629.7 Å were observed
with both CDS and SUMER; all other lines were observed only with SUMER.
These lines cover chromospheric, transition region and coronal
temperatures.
SUMER observed with detector B on 14 and 16 February 1997 (R. Thomas)
and 25 February 1997 (D. Gigas) using the
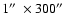 slit #2 and on 22, 23 and 25 April 1997 (I. Rüedi) using
slit #2 and on 22, 23 and 25 April 1997 (I. Rüedi) using
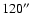 long slits #3 (
long slits #3 (
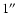 wide)
and #6 (
wide)
and #6 (
 wide).
The pixel size was
wide).
The pixel size was
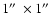 ,
except for the slit
#6 where it was
,
except for the slit
#6 where it was
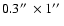 .
The SUMER slit was kept
at a fixed location on the solar surface by compensating for solar
rotation. Several instrumental corrections have been applied to the
data before the analysis. For the flat-field correction we used
flat-field images taken on 27 February 1997 and on 24 April 1997.
The pin cushion distortion of the image and the inclination of the
spectral lines with respect to the detector columns were removed. The
effects of the dead-time and gain-depletion of the detector were
almost negligible, but the corrections due to these effects have been
applied anyway. In the next step we fit the line profiles, except for
the N III 764.4 Å and C III 1175.7 Å lines, at each spatial
position and for each time step. For all lines least-squares fits of a
single Gaussian plus a linear background turned out to be sufficient.
The fitting procedure failed to give reliable fits for N III due to a
low signal-to-noise ratio and for C III which is strongly blended (actually
it is a multiplet of six C III lines). Since we were interested only in the
variations of the total intensity formed at a particular temperature
we spectrally summed over the line profile after subtracting for the
continuua determined from the N IV 765.2 Å and He I 584.3 Å
(recorded in 2nd order) lines, respectively.
.
The SUMER slit was kept
at a fixed location on the solar surface by compensating for solar
rotation. Several instrumental corrections have been applied to the
data before the analysis. For the flat-field correction we used
flat-field images taken on 27 February 1997 and on 24 April 1997.
The pin cushion distortion of the image and the inclination of the
spectral lines with respect to the detector columns were removed. The
effects of the dead-time and gain-depletion of the detector were
almost negligible, but the corrections due to these effects have been
applied anyway. In the next step we fit the line profiles, except for
the N III 764.4 Å and C III 1175.7 Å lines, at each spatial
position and for each time step. For all lines least-squares fits of a
single Gaussian plus a linear background turned out to be sufficient.
The fitting procedure failed to give reliable fits for N III due to a
low signal-to-noise ratio and for C III which is strongly blended (actually
it is a multiplet of six C III lines). Since we were interested only in the
variations of the total intensity formed at a particular temperature
we spectrally summed over the line profile after subtracting for the
continuua determined from the N IV 765.2 Å and He I 584.3 Å
(recorded in 2nd order) lines, respectively.
CDS/NIS was employed in its movie mode, i.e., with a
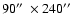 slit. In this mode a filtergram covering a part of the solar
surface corresponding to the slit size is produced simultaneously at each
wavelength. Due to the overlap of the images from neighbouring wavelengths
spectral information within each spectral line is lost. After correction
for solar rotation, performed on the ground, each pixel follows the
same point on the solar surface during the whole time series. To the actual
exposure time an overhead of four or five seconds per frame must be added
(the overhead is mainly accrued by reading out the CCD and preparing it for
the next exposure). Due to telemetry constraints somewhat less than half of
the data along the slit were read out and consequently only a smaller area
of the solar disc is covered. The correction for solar rotation further
reduces the size of the field of view, which finally is
slit. In this mode a filtergram covering a part of the solar
surface corresponding to the slit size is produced simultaneously at each
wavelength. Due to the overlap of the images from neighbouring wavelengths
spectral information within each spectral line is lost. After correction
for solar rotation, performed on the ground, each pixel follows the
same point on the solar surface during the whole time series. To the actual
exposure time an overhead of four or five seconds per frame must be added
(the overhead is mainly accrued by reading out the CCD and preparing it for
the next exposure). Due to telemetry constraints somewhat less than half of
the data along the slit were read out and consequently only a smaller area
of the solar disc is covered. The correction for solar rotation further
reduces the size of the field of view, which finally is
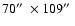 (
(
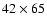 )
pixels, with a pixel size of
)
pixels, with a pixel size of
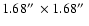 .
.
For more information about observations related to the variability
see also Brkovic et al. (2002) and for observations which
provided absolute shifts see Peter & Judge (1999) and Teriaca
et al. (1999).
3 Results
The time variability 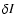 is described by the RMS variation of the
intensity during the time series. The (average) intensity is the average
over the whole duration of the observations. The relative variability
is described by the RMS variation of the
intensity during the time series. The (average) intensity is the average
over the whole duration of the observations. The relative variability
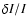 is defined as the ratio of the RMS to the intensity. These
three parameters were determined for each spatial pixel for the spectral
lines of interest. Finally, averages over all spatial locations were
formed for each line.
A similar procedure was employed to determine the RMS fluctuations of the
(relative) Doppler shifts.
is defined as the ratio of the RMS to the intensity. These
three parameters were determined for each spatial pixel for the spectral
lines of interest. Finally, averages over all spatial locations were
formed for each line.
A similar procedure was employed to determine the RMS fluctuations of the
(relative) Doppler shifts.
Figure 1a shows the Doppler shift variability of
each spectral line as a function of its temperature of formation. The
noise in the variability is found as the average of line position
errors determined from fits, over the period of observations and has
been removed. Negative values in the plot reflect the fact that the
lines formed at low temperatures (log T < 4.2) show very
small RMS fluctuations of the line position, which are smaller than
the noise introduced by the fitting errors, i.e., for these lines we
do not detect a solar RMS fluctuation in Doppler shift. In
Figs. 1b,c we plot the relative
intensity variability and mean Doppler shift as a function of its
temperature of formation. Note that the variabilities obtained by CDS,
denoted by squares in Fig. 1b, have been corrected in
order to make them comparable to the SUMER results (cf. Brkovic et
al. 2002). The error bars denote standard deviations. The
arrow attached to the symbol representing Mg IX indicates that the
measured variability of this line is an upper limit, due to noise.
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{MS2692f1.eps}
\end{figure}](/articles/aa/full/2003/20/aa2692/Timg15.gif) |
Figure 1:
Doppler shift variability a), relative intensity variability
b) and mean Doppler shift c) vs. formation temperature and relative
variability vs. mean Doppler shift d). |
![\begin{figure}
\includegraphics[width=8.8cm,clip]{MS2692f2.eps}\end{figure}](/articles/aa/full/2003/20/aa2692/Timg16.gif) |
Figure 2:
Differential emission measure vs. formation temperature a)
and relative intensity variability vs. differential emission measure
b). |
Since measuring absolute shifts was not one of the original aims of
these observations they are not well suited to obtaining reliable
values of the mean shift. We have therefore preferred to use the
values published by Peter & Judge (1999) and by Teriaca et
al. (1999). We face the problem that these
authors analysed some spectral lines not present in our sample and
vice versa. There are twelve common ions, designated by asterisks in
the Table 1, for which measured values of both the relative
variability and the Doppler shift are available. In order to preserve
the advantages of both investigations (the data of Teriaca et al.
1999 contain more lines, while only Peter & Judge
1999 include a truly coronal line in their sample) we have
combined the mean velocities from both and fit them with 2 straight
lines, one for log T < 5.3, the other for log T 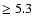 (dashed lines in Fig. 1c). From the fit for log T < 5.3 we obtained Doppler shifts for N III and N IV (triangles in
Fig. 1c), therefore we did not estimate errors for
these lines.
The estimated Doppler shift of 9.2 km s-1 for N IV
765.2 Å agrees very well with the value of 9 km s-1 published by
Brekke et al. (1997).
(dashed lines in Fig. 1c). From the fit for log T < 5.3 we obtained Doppler shifts for N III and N IV (triangles in
Fig. 1c), therefore we did not estimate errors for
these lines.
The estimated Doppler shift of 9.2 km s-1 for N IV
765.2 Å agrees very well with the value of 9 km s-1 published by
Brekke et al. (1997).
Figure 1d shows the average relative variability as
a function of Doppler shift. The temperature dependence of the mean
(spatially and temporally averaged) Doppler shift (
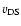 )
and the
relative variability are similar. The dashed line is a linear fit
through the data points,
)
and the
relative variability are similar. The dashed line is a linear fit
through the data points,
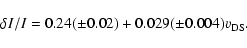 |
(1) |
The correlation coefficient between the relative variability and
Doppler shift is 0.92. According to probability calculus this
extremely tight relationship has a probability below 10-3 of
being due to chance, suggesting a physical connection between these
two quantities. A proposal for this connection is presented in Sect. 4.2.
3.2 Differential emission measure
Comparing Fig. 1b and the behaviour of the
differential emission measure (DEM) up
to one million K (the maximum temperature of our observations) we
notice that the relative variabilities and DEMs are anti-correlated.
Recall that DEM as a function of T decreases from 104 K
towards higher temperatures. Near
 K it reaches its
minimum, then it increases until
K it reaches its
minimum, then it increases until
 K and finally it
decreasess again. For the quantification of the relation of the DEM
and the RMS variations of the intensity we calculated DEMs using the
CHIANTI package (Dere et al. 1997). Of course, for the optically thick
He I line this does not make sense because of uncertainty in the
temperature of formation, so we skipped it. The plot of log DEM as a
function of log T is shown in Fig. 2a. Our results
are in a fair agreement with the standard DEM curve (cf. Mariska
1992).
K and finally it
decreasess again. For the quantification of the relation of the DEM
and the RMS variations of the intensity we calculated DEMs using the
CHIANTI package (Dere et al. 1997). Of course, for the optically thick
He I line this does not make sense because of uncertainty in the
temperature of formation, so we skipped it. The plot of log DEM as a
function of log T is shown in Fig. 2a. Our results
are in a fair agreement with the standard DEM curve (cf. Mariska
1992).
In the next step we directly compare log DEMs and relative
variabilities (Fig. 2b). The dashed line is a linear
fit through the data points. A simple comparison by eye of
Figs. 1d and 2b shows that for our data
the relation of the RMS fluctuations to the Doppler shifts is much
closer than that of the RMS fluctuations to the DEM. This is quantified
by the correlation coefficients, which are 0.92 and -0.78, respectively.
This might imply that relative variabilities are related more strongly to
Doppler shifts than to differential emission measures. However more data
are needed to draw a final conclusion.
Another interesting point which deserves to be mentioned is the
result presented by Wilhelm et al. (1998), who
analysed full Sun and Sun centre observations during the minimum of
solar activity in 1996. They found that the logarithms of line
radiances are normally distributed. Their plot of the widths of the
distributions (their Fig. 9) is qualitatively similar to our
Fig. 1b.
If one considers the distribution of intensities to be largely due to
temporal fluctuations, one should expect the width of the intensity
distribution to be related to the RMS fluctuations. This argument still
holds when considering spatial variations and assuming that the different
locations on the Sun are independent.
In this sense the RMS fluctuations (Fig. 1b) and the
widths of the intensity distributions (Wilhelm et al. 1998,
Fig. 9) are different ways to look at the same problem under the
assumption outlined above (see also Pauluhn et al. 2000).
4 Discussion
In the previous section we showed that relative variabilities of intensity
are highly correlated to Doppler shifts and modestly anti-correlated
to differential emission measures. We proceed with two simple, but
different interpretations of these results, before discussing implications
for the transition region structure.
Even though the anti-correlation between the differential emission measure (DEM) and the RMS variations of the intensity is not very high
(Sect. 3.2) we will outline a scenario for this
anti-correlation.
Using the definition of the DEM (e.g. Mariska 1992)
for constant pressure
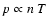 ,
with the (electron) number density n, the DEM is given by
,
with the (electron) number density n, the DEM is given by
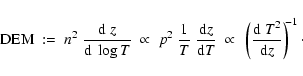 |
(2) |
This implies that the DEM is smallest where the temperature gradient is
largest and vice versa.
Taking, for the sake of argument an anti-correlation of DEM and RMS
intensity fluctuations for granted this means that the latter are
largest where the temperature gradient is steepest.
This leads to a selection effect which may give rise to a correlation
between intensity fluctuations and DEM (cf. Fig. 2b).
When the DEM gets smaller, the amount of emitting material gets
smaller and thus there is less averaging along the line of sight
(assuming that the individual structures do not become correspondingly
finer).
Thus one would expect the strongest fluctuations where the DEM is smallest.
Conversely, at temperatures where the DEM is large (e.g. large scale
height at coronal temperatures) there is a more effective averaging
along the line of sight.
Additionally, there may be more structures along the line of sight,
causing the observed fluctuations to decrease with T, while the
local fluctuations increase.
4.2 Relation to compressible fluctuations
Here we propose that the process leading to the tight relation of the
intensity RMS variations and the net Doppler shifts as shown in
Fig. 1d is based on compressible fluctuations.
This was inspired by sound waves, i.e., longitudinal compressions, which
lead also to net Doppler shifts: towards the red if the wave is propagating
away from the observer, towards the blue in the opposite case.
This behaviour can be shown in simple terms analytically for optically
thin lines formed in an isothermal constant-pressure atmosphere.
Investigating this effect Hansteen (1993) numerically modeled
coronal loops including sound waves propagating along the loops in order to
understand the transition region line shifts (solving the full 1D problem
along the loop).
Even though the real Sun is much more complicated, we use the simple
ansatz that compressible fluctuations cause a sinusoidal variation of the
intensity at line centre,
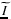 ,
at a given location in the
atmosphere, where the respective line of interest is formed,
,
at a given location in the
atmosphere, where the respective line of interest is formed,
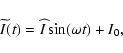 |
(3) |
where I0 can be considered as the mean line-centre intensity.
Accordingly the velocity,
 ,
varies as
,
varies as
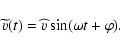 |
(4) |
Here
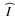 and
and
 denote the amplitude of the
intensity and velocity fluctuations, the wave frequency is given by
denote the amplitude of the
intensity and velocity fluctuations, the wave frequency is given by
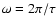 ,
with
,
with 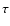 being the period of the wave, and tdenotes the time.
being the period of the wave, and tdenotes the time.
The phase lag, 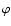 ,
between the intensity and the velocity
fluctuations is 0 for a pure upward moving sound wave and
,
between the intensity and the velocity
fluctuations is 0 for a pure upward moving sound wave and
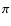 for a downward propagating sound wave.
Because of this the upward moving sound wave causes net blueshifts: for
for a downward propagating sound wave.
Because of this the upward moving sound wave causes net blueshifts: for
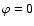 the intensity
the intensity
 is at its maximum when the gas is
moving upwards,
is at its maximum when the gas is
moving upwards,
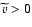 ,
and the intensity is lowest when the
gas is moving downwards,
,
and the intensity is lowest when the
gas is moving downwards,
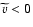 .
A net blueshift results by
averaging over one period
.
A net blueshift results by
averaging over one period 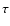 .
Similarly this gives a redshift for a
downward propagating wave with
.
Similarly this gives a redshift for a
downward propagating wave with
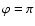 .
.
With this simple ansatz (3) and (4) it is
implicitly assumed that the atmosphere is "piecewise'' isothermal,
i.e., over the height of formation of the respective line the temperature
remains constant.
However, the atmosphere is not as simple as that and for a correct model
one would have to investigate also effects of the atmospheric structure as
well as of heat conduction and radiation; especially the time scales
involved in the latter processes.
Waves of any period can produce a net Doppler shift, even when not resolved
by observations (Hansteen 1993).
Therefore as a working hypothesis we assume that compressible fluctuations
with periods shorter than currently resolvable by observations, both
temporally and spatially, are leading to the observed net Doppler shifts.
Note, however, that in order to produce detectable velocity and
intensity fluctuations (and not just cause lineshifts from unresolved
motions, e.g. Wikstøl et al. 1997), the wavelength 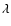 should
be at least of the order of the line formation length L.
Otherwise different (non-coherent) wave packages might cancel each other.
This puts a lower limit on the period,
should
be at least of the order of the line formation length L.
Otherwise different (non-coherent) wave packages might cancel each other.
This puts a lower limit on the period,
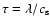 ,
of the wave,
i.e.,
,
of the wave,
i.e.,
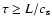 ,
with
,
with  being the sound speed.
For typical transition region values,
being the sound speed.
For typical transition region values,
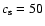 km s-1 at 105 K,
L=20 km (e.g., Mariska 1992) this would put the lower limit
of the wave period to
km s-1 at 105 K,
L=20 km (e.g., Mariska 1992) this would put the lower limit
of the wave period to
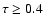 s.
s.
Using the above ansatz (3) and (4) the
line profile at time t is then given by
![\begin{displaymath}
I_v(t) =
\widetilde{I}(t) ~\exp\left[-\left(\frac{v-
\widetilde{v}(t)}{\sigma}\right)^2\right],
\end{displaymath}](/articles/aa/full/2003/20/aa2692/img45.gif) |
(5) |
with the (thermal) line width 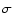 ,
i.e. the half line width at 1/e of the peak intensity.
Here the wavelength is given in velocity units and denoted by v.
,
i.e. the half line width at 1/e of the peak intensity.
Here the wavelength is given in velocity units and denoted by v.
As the fluctuations are assumed to be un-resolved in time, one has to average
the spectrum over one (or more) wave periods, which is given by
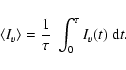 |
(6) |
This is the theoretical spectrum that one has to compare to observations
(after taking into account additional broadening due to, e.g., turbulence
on the Sun and instrumental effects).
Here we follow Hansteen (1993) and analyse the mean spectrum
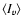 in terms of its moments.
in terms of its moments.
The zeroth moment of the line profile,
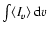 ,
is the (mean) total intensity,
,
is the (mean) total intensity,
 .
By using expressions (3)-(6) and
evaluating the integrals over wavelength and time this results in
.
By using expressions (3)-(6) and
evaluating the integrals over wavelength and time this results in
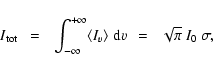 |
(7) |
i.e., the total intensity is given by the mean line-centre intensity I0
and the line width 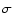 .
.
The line position, i.e., the Doppler shift,
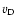 ,
is given by the
first moment of the line profile:
,
is given by the
first moment of the line profile:
 |
(8) |
i.e., the resulting Doppler shift
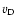 is proportional to the
relative variability of the intensity fluctuations
is proportional to the
relative variability of the intensity fluctuations
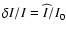 and the velocity amplitude
and the velocity amplitude
 .
The intensity and velocity fluctuations
.
The intensity and velocity fluctuations
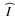 and
and
 in
Eq. (8) causing the net line shift
in
Eq. (8) causing the net line shift
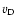 are
spatially or temporally not resolved!
are
spatially or temporally not resolved!
Nevertheless one might argue that the amplitude of the fluctuations
on shorter not resolved time scales are related to those on larger time
scales: a higher variability at high frequencies will in general be related
to stronger slow fluctuations.
This speculation is based on the idea of turbulence, where the
fluctuations on small and large scales are connected by a power law
(e.g., Cally 1990 argued that turbulence plays an important role in
the low transition region).
However, one has to be careful to simply copy the arguments of
turbulence to the present situation.
Dere (1989) analysed the power spectrum of spatial velocity
fluctuations for the 1548 Å and 1550 Å lines of C IV formed
at about 105 K.
He found the power spectrum to be too flat to be due to a turbulent
cascade, implying that most of the power is concentrated at small scales,
and he speculated that some driving mechanism such as magnetic reconnection
is maintaining the flat power spectrum. At other temperatures the shape of
the power spectrum is not known.
In conclusion it seems plausible that within the framework of this simplistic
model one might relate the un-resolved fluctuations
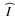 and
and
 in Eq. (8) to the observed temporally
and spatially resolved (RMS) fluctuations
in Eq. (8) to the observed temporally
and spatially resolved (RMS) fluctuations
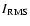 and
and
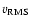 .
Then from Eq. (8) it follows that
.
Then from Eq. (8) it follows that
 ,
which is in good agreement with the linear
relation between the observed Doppler shifts and intensity (RMS)
fluctuations shown in Fig. 1d.
,
which is in good agreement with the linear
relation between the observed Doppler shifts and intensity (RMS)
fluctuations shown in Fig. 1d.
Following the suggestion of Peter & Judge (1999) below
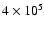 K the redshifts are caused by downward propagating waves,
above that temperature the blueshifts are caused by upward propagating
waves:
the phase lag
K the redshifts are caused by downward propagating waves,
above that temperature the blueshifts are caused by upward propagating
waves:
the phase lag 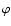 has a jump from 0 to
has a jump from 0 to 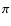 ,
i.e.,
,
i.e.,
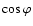 changes sign there.
Thus for the absolute value of the Doppler shift one expects
changes sign there.
Thus for the absolute value of the Doppler shift one expects
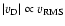 .
The observed relation between Doppler shift and Doppler shift (RMS)
variations is not as clear as for
.
The observed relation between Doppler shift and Doppler shift (RMS)
variations is not as clear as for
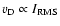 (a
correlation coefficient of 0.58 compared to 0.92), but still
remarkable (Fig. 3).
(a
correlation coefficient of 0.58 compared to 0.92), but still
remarkable (Fig. 3).
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{MS2692f3.eps}
\end{figure}](/articles/aa/full/2003/20/aa2692/Timg62.gif) |
Figure 3:
RMS of Doppler shift vs. absolute mean Doppler shift. For the
explanation of negative values see Sect. 3. |
Of course, this simple model can only outline an interpretation of the
relations of the observed RMS fluctuations to the Doppler shift, but it
suggests that the transition region line shifts are a consequence of
compressible fluctuations.
A more detailed (numerical) modeling for a "realistic'' transition region
is needed for further insight.
Such work is in progress.
4.3 Connection between the transition region and
the corona
There is a long-standing debate whether or not there is a continuous
connection from the chromosphere to the corona.
"Classical'' transition region models like that of Gabriel
(1976) assume that the transition region emission originates
from the thermal interface between the chromosphere and the corona.
Here, transition region emission stands for lines formed at temperatures
from say 20 000 K to below 106 K, if one assumes ionisation
equilibrium.
"Non-classical'' transition region models propose that this emission comes
from a structure or structures not connected to the corona (e.g., Feldman
1983, 1998).
However, it is not possible to decide between these two radically
different pictures based on observations alone, but one has to make implicit
assumptions on the physical nature of the structure in question
(Judge & McIntosh 1999).
For example, Wikstøl et al. (1998) showed that most of the
"observational facts'' usually used to argue in favour of a
"non-classical'' transition region can also be reproduced in a forward
model of a "classical'' continuous transition region from the chromosphere
to the corona when accounting for the dynamic nature of this region.
This whole debate is thoroughly reviewed by Judge & McIntosh
(1999).
The results of the present study as shown in Figs. 1-3 add a valuable piece of information
to this discussion.
When plotting emission measures or Doppler shifts versus formation
temperature (Figs. 2a and 1c) the lines fall
into two groups with formation temperatures below and above
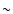
 K.
This is not the case for the "scatter plots'' of intensity fluctuation
vs. Doppler shift or DEM
(Figs. 1d and 2b)
as well as for Doppler shift fuctuations vs. Doppler shift (Fig. 3).
For a "non-classical'' transition region model this suggests that in the
different (geometrically not connected) structures the same process is
producing the line shifts.
K.
This is not the case for the "scatter plots'' of intensity fluctuation
vs. Doppler shift or DEM
(Figs. 1d and 2b)
as well as for Doppler shift fuctuations vs. Doppler shift (Fig. 3).
For a "non-classical'' transition region model this suggests that in the
different (geometrically not connected) structures the same process is
producing the line shifts.
5 Conclusions
We have investigated the variation of brightness and Doppler shifts from the
quiet Sun using time series obtained by SUMER and CDS in chromospheric,
transition region and coronal lines. We found a high correlation of 0.92 between averaged Doppler shifts and relative intensity
variabilities of the lines studied.
The fact that the relation between these two quantities is the same for
transition region and coronal lines argues that the same physical process
is acting to produce the net Doppler shift, intensity variability and
probably Doppler shift variability in the different atmospheric layers.
Based on the data for this analysis we found a less significant correlation
between the intensity variability and the differential emission measure
(correlation coefficient -0.78), which indicates that simple selection
effects are at most a part of the reason for the correlation between
intensity variability and Doppler shift.
Assuming that the connection between the net Doppler shifts and the
relative RMS intensity fluctuations is based on compressible fluctuations
like sound waves we show that a simple analytical model can nicely
reproduce the statistical relations between the Doppler shifts, Doppler
shift variations and intensity variations.
However, further modelling, that isbeyond the scope of this work, is
needed to provide a more solid foundation to these interpretations.
Acknowledgements
We are grateful to the SUMER and CDS teams and their open data policy.
The SUMER project is financially supported by DLR, CNES, NASA and the
ESA PRODEX programme (Swiss contribution). We thank the referee Philip
Judge for carefully reading and thoroughly commenting on the manuscript.
AB thanks J. O. Stenflo and M. C. E. Huber for their encouragement and
support. The work of AB was supported by the Swiss National Science
Foundation grant No. 21-45083.95, by a grant from the ETH-Zürich,
and by the Deutsche Forschungsgemeinschaft grant No. PE 782, which
is gratefully acknowledged. SOHO is a mission of international
cooperation between ESA and NASA.
- Antiochos, S. K. 1984, ApJ, 280, 416
NASA ADS
- Athay, R. G., Gurman, J. B., Henze, W., & Shine, R. A.
1983, ApJ, 265, 519
NASA ADS
- Brekke, P., Hassler, D. M., & Wilhelm, K. 1997, Sol. Phys., 175,
349
NASA ADS
- Brkovic, A., Rüedi, I., Solanki, S. K., et al.
2000, A&A, 353, 1083
NASA ADS
- Brkovic, A., Solanki, S. K., & Rüedi, I.
2002, A&A, 385, 257
NASA ADS
- Cally, P. S. 1990, ApJ, 355, 693
NASA ADS
- Dere, K. P. 1989, ApJ, 340, 599
NASA ADS
- Dere, K. P., Bartoe, J.-D. F., & Brueckner, G. E.
1984, ApJ, 281, 870
NASA ADS
- Dere, K. P., Landi, E., Mason, H. E., Monsignori
Fossi, B. C., & Young, P. R. 1997, A&AS, 125, 149
NASA ADS
- Feldman, U. 1983, ApJ, 275, 367
NASA ADS
- Feldman, U. 1998, ApJ, 507, 974
NASA ADS
- Gabriel, A. 1976, Phil. Trans. Royal Soc.
London, 281, 339
NASA ADS
- Gebbie, K. B., Hill, F., November, J., et al.
1981, ApJ, 251, L115
NASA ADS
- Hansteen, V. H. 1993, A&A, 402, 741
- Hansteen, V. H., Betta, R., & Carlsson, M.
2000, A&A, 360, 742
NASA ADS
- Harrison, R. A. 1997, Sol. Phys., 175, 467
NASA ADS
- Harrison, R. A., Sawyer, E. C., Carter, M. K., et al.
1995, Sol. Phys., 162, 233
NASA ADS
- Judge, P. G., & McIntosh, S. W. 1999, Sol. Phys., 190, 331
NASA ADS
- Krucker, S., Benz, A. O., Bastian, T. S., & Acton L. W.
1997, ApJ, 488, 499
NASA ADS
- Landini, M., & Monsignori Fossi, B. C. 1990, A&AS, 82,
229
NASA ADS
- Mariska, J. T. 1988, ApJ, 334, 489
NASA ADS
- Mariska, J. T. 1992, The Solar
Transition Region (Cambridge Univ. Press)
- McClymont, A. N., & Craig, I. J. D. 1987, ApJ, 312, 402
NASA ADS
- Peter, H., & Judge, P. G. 1999, ApJ, 522, 1148
NASA ADS
- Pauluhn, A., Solanki, S. K., Rüedi, I.,
Landi, E., & Schühle, U. 2000, A&A, 362, 737
NASA ADS
- Rabin, D., & Dowdy, J. F. Jr. 1992, ApJ, 398, 665
NASA ADS
- Spadaro, D., Lanza, A. F., & Antiochos, S. K. 1996, ApJ, 462, 1011
NASA ADS
- Stucki, K., Solanki, S. K., Schühle, U., & Rüedi, I.
2000, A&A, 362, L49
NASA ADS
- Teriaca, L., Banerjee, D., & Doyle, J. G.
1999, A&A, 349, 636
NASA ADS
- Wikstøl, Ø., Judge, P. J., & Hansteen,
V. 1997, ApJ, 483, 972
NASA ADS
- Wikstøl, Ø., Judge, P. J., & Hansteen,
V. 1998, ApJ, 501, 895
NASA ADS
- Wilhelm, K., Curdt, W., Marsch, E., et al.
1995, Sol. Phys., 162, 189
NASA ADS
- Wilhelm, K., Lemaire, P., Dammasch, I. E., et al.
1998, A&A, 334, 685
NASA ADS
Copyright ESO 2003
![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{MS2692f1.eps}
\end{figure}](/articles/aa/full/2003/20/aa2692/Timg15.gif)
![\begin{figure}
\includegraphics[width=8.8cm,clip]{MS2692f2.eps}\end{figure}](/articles/aa/full/2003/20/aa2692/Timg16.gif)
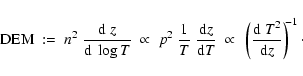
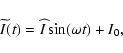
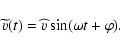
![\begin{displaymath}
I_v(t) =
\widetilde{I}(t) ~\exp\left[-\left(\frac{v-
\widetilde{v}(t)}{\sigma}\right)^2\right],
\end{displaymath}](/articles/aa/full/2003/20/aa2692/img45.gif)
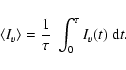
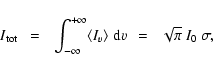

![\begin{figure}
\par\includegraphics[width=8.8cm,clip]{MS2692f3.eps}
\end{figure}](/articles/aa/full/2003/20/aa2692/Timg62.gif)