A&A 365, 144-156 (2001)
DOI: 10.1051/0004-6361:20000174
E. Dartois1 - L. d'Hendecourt2
Send offprint request: E. Dartois,
1 - Institut de RadioAstronomie Millimétrique (UPS2074),
300 rue de la piscine, 38406 Saint Martin d'Hères, France
2 -
Institut d'Astrophysique Spatiale (MR8617), Bât. 121, Université Paris XI, 91405 Orsay Cedex, France
Received 28 July 2000 / Accepted 29 September 2000
Abstract
We present a study of the 3.1 micron absorption band, attributed to the OH stretching mode of the water molecule in the form of solid ice, observed in many protostellar lines of sight by the SWS instrument on board ISO. Using ice optical constants and Mie theory, we obtain reasonable fits
to the peculiar band shapes observed in twelve sources. The fits clearly show that some scattering effects arise in this absorption band, as the grain sizes used in the calculations are of the order of a few tenths of a micron. In the fit residuals, we search for the ![]() 1 and
1 and ![]() 3 vibrations of
the ammonia molecule which fall in the same spectral region, leading only to upper limits for the NH3 content of a few percents of the total water ice content in these different lines of sight. We also discuss the occurrence of a 3.47
3 vibrations of
the ammonia molecule which fall in the same spectral region, leading only to upper limits for the NH3 content of a few percents of the total water ice content in these different lines of sight. We also discuss the occurrence of a 3.47 ![]() m absorption band which could be related to the formation of an ammonia hydrate in the ice mantles. On the assumption that this band is due merely to this hydrate and with the help of relevant laboratory experiments, we show that
ammonia represents therefore at most 5% in abundance relative to water ice in these interstellar grain mantles. Finally, this study sheds light on the "3.1
m absorption band which could be related to the formation of an ammonia hydrate in the ice mantles. On the assumption that this band is due merely to this hydrate and with the help of relevant laboratory experiments, we show that
ammonia represents therefore at most 5% in abundance relative to water ice in these interstellar grain mantles. Finally, this study sheds light on the "3.1 ![]() m/6
m/6 ![]() m column density paradox" obtained when comparing the water ice absorption band at 3.1 (stretching mode) and 6
m column density paradox" obtained when comparing the water ice absorption band at 3.1 (stretching mode) and 6 ![]() m (bending mode). We show that this paradox is resolved by considering different extinction regimes, in which scattering affects only the 3.1
m (bending mode). We show that this paradox is resolved by considering different extinction regimes, in which scattering affects only the 3.1 ![]() m band whereas pure absorption dominates at 6
m band whereas pure absorption dominates at 6 ![]() m.
m.
Key words: ISM: clouds - dust, extinction - ISM: lines and bands - ISM: magnetic fields
Author for correspondance: dartois@iram.fr
Nitrogen cosmic abundance is high (
![]() N
N![]() ,
Snow & Witt 1996), and the presence of solid state molecules
containing this atom appears therefore logical.
Hydrides of the most abundant elements O, C and N, in the form of H2O,
CH4 and NH3 were proposed as early as
1949 by van de Hulst and models of molecular cloud chemistry involving gas and grains interactions clearly showed that these species should be made and stored, at least partially, on interstellar grain mantles (Tielens 1982; d'Hendecourt et al. 1985). The detection of a strong absorption band at 3.1 micron in the mid 70's was firmly attributed to water ice, on the basis of laboratory experiments (Léger et al. 1979) followed by many others (Hagen et al. 1983; Smith et al. 1989) as well as further observations (Willner et al. 1982; Whittet 1983).
,
Snow & Witt 1996), and the presence of solid state molecules
containing this atom appears therefore logical.
Hydrides of the most abundant elements O, C and N, in the form of H2O,
CH4 and NH3 were proposed as early as
1949 by van de Hulst and models of molecular cloud chemistry involving gas and grains interactions clearly showed that these species should be made and stored, at least partially, on interstellar grain mantles (Tielens 1982; d'Hendecourt et al. 1985). The detection of a strong absorption band at 3.1 micron in the mid 70's was firmly attributed to water ice, on the basis of laboratory experiments (Léger et al. 1979) followed by many others (Hagen et al. 1983; Smith et al. 1989) as well as further observations (Willner et al. 1982; Whittet 1983).
The ISO satellite, observing above the atmosphere has fully confirmed the presence of massive amounts of H2O ice in the spectra of many protostars as well as of other solid state species such as CO, CO2, CH4 (d'Hendecourt et al. 1996; Schutte et al. 1996; Gerakines et al. 1999; Boogert et al. 2000). Yet, solid-state nitrogen containing species remain quite elusive and the detection of ammonia ice remains controversial. However, the ammonia molecule is detected since a long time in the gas phase in molecular clouds (e.g. Cheung et al. 1969), with relatively high abundances respective to H2 (10 -7-10-8, Ho & Townes 1983; Turner 1995).
Before ISO, laboratory studies using astrophysical ice mixtures (Hagen
et al. 1983) have provided constraints on the shape of the 3.1 micron absorption
band by using mixtures of water and ammonia. Van de Bult et al. (1985) confirmed this constraint using Mie calculations on H
![]() mixtures. However, it should be noted that ground based observations (Smith
et al. 1989) obtained a low upper limit of only 2% of ammonia in two
sources (BN and GL989). The upper limit placed by Whittet et al. (1996) in HH100-IR
is only 8%, and the discussion on the detectability of this band under low mixing ratios with water ice questioned.
mixtures. However, it should be noted that ground based observations (Smith
et al. 1989) obtained a low upper limit of only 2% of ammonia in two
sources (BN and GL989). The upper limit placed by Whittet et al. (1996) in HH100-IR
is only 8%, and the discussion on the detectability of this band under low mixing ratios with water ice questioned.
Detection of ammonia ice has been claimed by Lacy et al. (1998) through the observation of its umbrella mode located in the wing of the strong silicate feature observed in the NGC 7538 IRS9 young stellar source. In the same paper however, no detection is reported for the other objects studied. ISO SWS data of the same source does not show the same optical depth in
this peculiar feature. One of the difficulties in the interpretation of this band lies in the high abundance of methanol in NGC 7538 IRS9, at a level of 10% of water ice, which contributes significantly to the optical depth in this spectral region. The derived abundance of ammonia in this source (10%) must be confirmed by the observation of other bands. Indeed, when the silicate band is extracted, there still exist two additional adjacent lines of methanol (located at 8.85 and 9.75 ![]() m) to extract. Therefore, the exact percentage of NH3 has to be taken with care as an uncertainty of 100% would be easily hidden in the saturation effects. Recently, Chiar et al. (2000) claimed the detection of very large amounts of ammonia (20-30%) in ice mantles toward Galactic Centre sources. We show, in the remaining of this paper that this detection is indeed questionable.
m) to extract. Therefore, the exact percentage of NH3 has to be taken with care as an uncertainty of 100% would be easily hidden in the saturation effects. Recently, Chiar et al. (2000) claimed the detection of very large amounts of ammonia (20-30%) in ice mantles toward Galactic Centre sources. We show, in the remaining of this paper that this detection is indeed questionable.
A low abundance of ammonia may result from locking of nitrogen in the form of N2, as suggested by the model calculations of d'Hendecourt et al. (1985), where, after the complete transformation of atomic hydrogen in H2, hydrides are not formed so efficiently and species such as O2 and N2 start to dominate the overall ice mantle composition. N2 being almost totally unobservable in the IR, its vibration band (weakly activated by interactions in an ice mixture, like O2, Ehrenfreund et al. 1992) falls right on the deep absorption of CO2, a solid state feature ubiquitous in the ISM (de Graauw et al. 1996).
Finally, the last and rather important aspect of the search for solid state ammonia is linked to the presence of a strong absorption band located at 4.62 ![]() m in many protostellar sources. This band was easily reproduced in laboratory experiments involving photochemical evolution of ice mixtures, as early as its discovery (Lacy et al. 1984). Tentatively attributed to a CN stretching mode by d'Hendecourt et al. (1986) in a unspecified species, its identification to the OCN-ion was convincingly made in subsequent laboratory experiments (Grim & Greenberg 1987; Schutte & Greenberg 1997;
Demyk et al. 1998;
Pendleton et al. 1999;
Palumbo et al. 2000 and references therein).
It is, until now, the best hint for the presence of nitrogen containing species in grain mantles. Laboratory mixtures used to photochemically produce the species at the origin of this band make a large use of ammonia and CO. Thus, constraints on the abundance of ammonia in interstellar ices appear a powerful tool to understand the overall chemistry (e.g. photochemistry, ion bombardments or surface chemistry) of molecular mantles in the ISM.
m in many protostellar sources. This band was easily reproduced in laboratory experiments involving photochemical evolution of ice mixtures, as early as its discovery (Lacy et al. 1984). Tentatively attributed to a CN stretching mode by d'Hendecourt et al. (1986) in a unspecified species, its identification to the OCN-ion was convincingly made in subsequent laboratory experiments (Grim & Greenberg 1987; Schutte & Greenberg 1997;
Demyk et al. 1998;
Pendleton et al. 1999;
Palumbo et al. 2000 and references therein).
It is, until now, the best hint for the presence of nitrogen containing species in grain mantles. Laboratory mixtures used to photochemically produce the species at the origin of this band make a large use of ammonia and CO. Thus, constraints on the abundance of ammonia in interstellar ices appear a powerful tool to understand the overall chemistry (e.g. photochemistry, ion bombardments or surface chemistry) of molecular mantles in the ISM.
In this paper, we first present the ISO-SWS data set of young stellar
objects observations. We then focus on the reduction procedure used to
obtain an homogeneous sample of objects. The observations are then fitted
using Mie theory and we search for NH3 absorptions in the fit residuals.
Negative results of this search are discussed. We show that if ammonia is
present, the formation of an hydrate of ammonium takes place that is
responsible for the formation of a weak band at 3.47 ![]() m, a band that is
observed in many objects (Allamandola et al. 1992; Brooke et al. 1996) as well as in some of the objects in our sample. If our interpretation is
correct, then an amount of about 5% of ammonia at most can be deduced from
our spectral analysis.
m, a band that is
observed in many objects (Allamandola et al. 1992; Brooke et al. 1996) as well as in some of the objects in our sample. If our interpretation is
correct, then an amount of about 5% of ammonia at most can be deduced from
our spectral analysis.
A byproduct of this study is to propose a
simple explanation to the problem of the discrepancy obtained in water
ice column densities derived when interpreting two different modes at 3.1
and 6 ![]() m.
m.
The spectra presented here come from the ISO data archive (http://www.iso.vilspa.esa.es/). We have reduced moderate resolution (SWS01) and high resolution (SWS06) spectra in the following manner. The simple method discussed here makes sure that any feature discussed is not contaminated by a bad detector response or a misalignment between sub-bands that cover the observed very broad band, which must be taken into account when broad solid state features are considered.
The ISO observing mode recorded the spectra with a grating, scanning wavelengths alternatively in the increasing and decreasing wavelength directions, the resultant spectrum being the sum of at least one "upward'' and one "downward'' scan. When a strong flux gradient in the observed band, or a strong narrow line (such as an H2 or HI line) is present, some memory effects can affect the final spectrum and upward and downward scans are not superimposable. Moreover, when looking at broad bands, such as the water ice modes, the spectrum is constructed with sub-bands from different detectors that overlap and whose aperture in the sky can change, depending on the sub-band considered. Consequently, the sum of the two scans may not be representative of the true flux.
To disentangle these effects, during the reduction we separate the downward scans from the upward ones. In doing so, we lose a factor
![]() in the signal-to-noise ratio, but we get in return two individual scans that must be identical (above the signal-to-noise ratio), any deviation in one scan being considered as a bad behaviour. The limits we will derive hereafter are therefore conservative. To be sure that no problem arises in the overlapping of two sub-bands, we decide to use a gain correction in a small overlapping region between bands (a few percent of the total bandwidth). If two scans overlap in a small spectral region, we calculate the average of the flux in the wavelength region they have in common and apply a multiplying factor to the second scan so that the overlapping regions match in flux. This correction is especially needed in the case of the NH3 stretching modes which are located near the overlapping of the ISO-SWS 1b and 1d band. All these corrections, as well as the resulting spectra in
upward and downward scans are presented in Fig. 1.
in the signal-to-noise ratio, but we get in return two individual scans that must be identical (above the signal-to-noise ratio), any deviation in one scan being considered as a bad behaviour. The limits we will derive hereafter are therefore conservative. To be sure that no problem arises in the overlapping of two sub-bands, we decide to use a gain correction in a small overlapping region between bands (a few percent of the total bandwidth). If two scans overlap in a small spectral region, we calculate the average of the flux in the wavelength region they have in common and apply a multiplying factor to the second scan so that the overlapping regions match in flux. This correction is especially needed in the case of the NH3 stretching modes which are located near the overlapping of the ISO-SWS 1b and 1d band. All these corrections, as well as the resulting spectra in
upward and downward scans are presented in Fig. 1.
![\begin{figure}
\par\includegraphics[bb=7 207 583 540,width=8cm,clip]{ds10157f9.p...
...m}
\includegraphics[bb=7 207 593 540,width=8cm,clip]{ds10157f12.ps}
\end{figure}](/articles/aa/full/2001/02/aa10157/Timg13.gif) |
Figure 1:
b) Same as in a)
the sources with no data in between
3.5 and 3.98 |
The 3 micron astronomical water ice band appears to be relatively broader in the observed
sources than the one measured in laboratory transmittance spectra. Furthermore,
the observed asymmetry (Fig. 1) giving a more extended red wing is typical
of what is expected from scattering of light by particles that approach a size
comparable to the wavelength (see Bohren & Hoffman 1983 for a comprehensive
description).
We know from the rest of the infrared
protostellar spectra that good fits to the data can be obtained
using thin film spectra measured directly in the laboratory,
without taking care of scattering effects, in the bands observed at
wavelengths above ![]() 5 microns (d'Hendecourt et al. 1998).
5 microns (d'Hendecourt et al. 1998).
The important parameter when discussing the possible scattering effects is
![]() which must remain
which must remain ![]() ,
m being the complex refractive index of the material considered, in order to neglect scattering and consider only pure absorption.
,
m being the complex refractive index of the material considered, in order to neglect scattering and consider only pure absorption.
These considerations tend to point out that the grain size distribution must contain grains of the order of
![]() to 0.5
to 0.5 ![]() m for scattering to affect the shape of the 3
m for scattering to affect the shape of the 3 ![]() m band (typical index of refraction of ices in this wavelength region lies between 1. and 1.5).
m band (typical index of refraction of ices in this wavelength region lies between 1. and 1.5).
To investigate the effects of scattering of such
grains on the spectra we use a Mie scattering code,
developed by Bohren & Huffman (1983). This
code allows us to derive the extinction coefficients
(
![]() )
in the case of a coated spherical particle. To run this program we need to specify the complex index of refraction of the
material in the core and in the mantle of the grain, as well as the respective grain radius for each component. We use here the classical Draine and Lee optical constants (1984) for the silicate core of the grain. As there is no strong transition from silicates falling in this spectral region, modifying the kind of silicates used for the core will not strongly affect the results presented here. For the ice mantle we need more refinements to explain the various line shapes observed. In particular we need to specify precisely its degree of crystallisation (associated with the temperature of the grains). For this, we use the constants carefully derived by Schmitt et al. (1998) for the extreme ice temperature components. For intermediate temperatures we calculate the optical constants from pure transmittance spectra recorded in the laboratory (from Institut d'Astrophysique Spatiale, Orsay, France and University of Leiden, The Netherlands).
)
in the case of a coated spherical particle. To run this program we need to specify the complex index of refraction of the
material in the core and in the mantle of the grain, as well as the respective grain radius for each component. We use here the classical Draine and Lee optical constants (1984) for the silicate core of the grain. As there is no strong transition from silicates falling in this spectral region, modifying the kind of silicates used for the core will not strongly affect the results presented here. For the ice mantle we need more refinements to explain the various line shapes observed. In particular we need to specify precisely its degree of crystallisation (associated with the temperature of the grains). For this, we use the constants carefully derived by Schmitt et al. (1998) for the extreme ice temperature components. For intermediate temperatures we calculate the optical constants from pure transmittance spectra recorded in the laboratory (from Institut d'Astrophysique Spatiale, Orsay, France and University of Leiden, The Netherlands).
To calculate the constants, we assume that the transmittance is given by the classical equation:
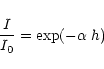
We calculate the refractive index n using the Kramers-Kronig (Bohren & Huffman) relation:
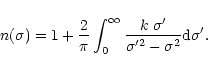
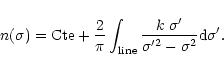
The resultant optical constants used for the Mie calculations are presented in Fig. 2 for water ice. They are presented in increasing temperature order (from 10 K to 160 K from top to bottom), except for the last one which is entirely crystalline, but have been annealed and measured at 60 K. This last spectrum is however representative of the totally crystalline ice band. Note that the main effect in changing the constant is the crystallinity. The constants we calculate are not determined with the same accuracy as the ones of Trotta and Schmitt, but are sufficient for the study we present hereafter.
To represent the variation in temperature of the ice mantle we do not use the constants as they are, but rather use the Maxwell-Garnett theory to produce mean optical constants for an ice mantle including several ice phases. This theory allows us to calculate the average dielectric function of mixed materials embbeded in a matrix, the other constituents being inclusions. The Maxwell Garnett average dielectric function is given by:
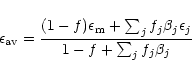
This dielectric function
![]() is easily related to n+ik through the constitutive relations:
is easily related to n+ik through the constitutive relations:
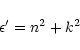

To extract the transmittance spectrum, we fit the same order polynomial as the one
we used to extract the water ice band in the ISO observations. An example of the
procedure is shown for the Orion BN case in Fig. 3, assuming AV for this
object is 17 (Gezari et al. 1998).
To illustrate the effect of the continuum extraction as well as scattering,
we show this extraction in the case we take into account only the absorption
coefficient
![]() and the case of the full extinction
and the case of the full extinction
![]() .
We first present the fit obtained extracting directly the optical depth from
.
We first present the fit obtained extracting directly the optical depth from
![]() .
However in the observations we measure a flux corresponding to
.
However in the observations we measure a flux corresponding to
![]() ), where
), where
![]() )
is the Planck function at temperature T. That is why the spectra present
a steep rise with wavelength. Therefore to show that our
fit in the upper panel is correct, we present just below the same curves (fit and transmittance) multiplied by a blackbody of
)
is the Planck function at temperature T. That is why the spectra present
a steep rise with wavelength. Therefore to show that our
fit in the upper panel is correct, we present just below the same curves (fit and transmittance) multiplied by a blackbody of ![]() 500 K, typical of the apparent temperature of the underlying continuum source. The final optical depths derived in both cases (with total extinction or just absorption) as well as the corresponding optical depths differences are presented below to clearly denote the effects of scattering. Of course all of this approach is not what would require the full radiative transfer treatment of the problem. However, it allows us to show we can explain to first order the entire water ice mode with the combination of different temperatures and simple scattering effects, without adding any other compound opacity.
500 K, typical of the apparent temperature of the underlying continuum source. The final optical depths derived in both cases (with total extinction or just absorption) as well as the corresponding optical depths differences are presented below to clearly denote the effects of scattering. Of course all of this approach is not what would require the full radiative transfer treatment of the problem. However, it allows us to show we can explain to first order the entire water ice mode with the combination of different temperatures and simple scattering effects, without adding any other compound opacity.
This probably explains why there still exists some lack of extinction in part of
the red wing of the band. However, in some cases (e.g. SGRA*, IRAS 17424),
the 3.4 ![]() m absorption band is clearly due to the presence of an aliphatic organic component.
In almost all other sources a right wing as well as a
band at 3.47
m absorption band is clearly due to the presence of an aliphatic organic component.
In almost all other sources a right wing as well as a
band at 3.47 ![]() m remains, the later one thoroughly studied by Brooke &
Sellgren (1999) in numerous objects. As emphasised by
the authors, this band appears to be correlated with the water ice band.
m remains, the later one thoroughly studied by Brooke &
Sellgren (1999) in numerous objects. As emphasised by
the authors, this band appears to be correlated with the water ice band.
We do not claim our fits to be unique. They are intended to demonstrate
that the whole band can be adjusted by water ice at various states of
crystallinity. Because of the interplay between too many physical parameters,
we believe that the problem cannot be simply inverted and the exact parameters
of the band (size distribution, exact ice temperature, ...)
extracted. However, these fits allow us to search for the NH3
stretching mode absorption bands in the residuals between the fit and
the observation, as shown in the upper part of each panel of Fig. 4.
We do not detect any convincing band which could be attributed to
NH3 in any source, and estimate an upper limit on its abundance in
the following manner. We calculate the
2![]() level of the residual of
the fit in the interval of the band for the upward and downward scans separately.
We multiply this by the expected width of the feature (40 cm-1). We obtain in
this way an integrated absorbance. The ammonia spectra corresponding to the
maximum contribution to the residual are presented for each source in
Figs. 4a and 4b (upper traces, one for each wavelength scanning directions).
level of the residual of
the fit in the interval of the band for the upward and downward scans separately.
We multiply this by the expected width of the feature (40 cm-1). We obtain in
this way an integrated absorbance. The ammonia spectra corresponding to the
maximum contribution to the residual are presented for each source in
Figs. 4a and 4b (upper traces, one for each wavelength scanning directions).
In parallel we integrate the fit performed on the water ice band and derive the ratio of the resulting two integrated absorbances. This is done for each scan direction. We did not only fit the region where the 2.97 ![]() m feature occurs, but the whole water ice stretching mode, i.e. including the red part of the absorption for example that is not reproduced e.g. in the Chiar et al. (2000) analysis. To derive a more stringent upper limit on NH3 we would have to ask what
H2O ice parameters allow the largest amount of NH3, and not what parameters allow the best fits of the spectra without
NH3. However, we believe that the global fit performed gives a good representation of the real state of water ice in all the wavelength range of the spectrum in the region free of NH3 lines, and that it is therefore very close to the actual water ice absorption.
m feature occurs, but the whole water ice stretching mode, i.e. including the red part of the absorption for example that is not reproduced e.g. in the Chiar et al. (2000) analysis. To derive a more stringent upper limit on NH3 we would have to ask what
H2O ice parameters allow the largest amount of NH3, and not what parameters allow the best fits of the spectra without
NH3. However, we believe that the global fit performed gives a good representation of the real state of water ice in all the wavelength range of the spectrum in the region free of NH3 lines, and that it is therefore very close to the actual water ice absorption.
If we multiply the integrated absorbances ratios derived by the ratio of the
measured integrated absorption coefficient of the H2O and NH3 stretching modes,
we are then able to derive the maximum percentage of ammonia undetected
in the water ice. Note that this ratio can vary upon the mixture involved. We have
searched for values in the literature for the NH3 stretching mode absorption
coefficient. The one determined by d'Hendecourt et al. (1986)
is
![]() cmmol-1. We also
determine this absorption
coefficient using the relation:
cmmol-1. We also
determine this absorption
coefficient using the relation:

| Source name | up | down | upper limit |
| ratio (%) | ratio (%) | NH3/H2O | |
| SGRA* | 0.71 | 0.71 | <11-17% |
| S140 | 0.23 | 0.22 | <3.4-5.3% |
| Orion | 0.31 | 0.27 | <4.4-6.9% |
| NGC 7538 IRS1 | 0.93 | 1.20 | <16-25% |
| IRAS 17424 | 0.82 | 0.75 | <12-19% |
| GL989 | 0.31 | 0.29 | <4.5-7.1% |
| GL490 | 0.83 | 0.74 | <12-19% |
| GL2591 | 0.42 | 0.57 | <7.4-12% |
| GL2136 | 1.19 | 0.94 | <16-25% |
| NGC 2024 IRS2 | 0.40 | 0.47 | <6.5-10% |
| RCRA | 1.25 | 1.07 | <17-27% |
| ELIAS 16 | 0.70 | 0.63 | <9.9-15% |
For water ice, d'Hendecourt et al. (1986) and
Gerakines et al. (1995)
determined
![]() 10-16 cmmol-1
whereas Hudgins et al. (1993) gives (
10-16 cmmol-1
whereas Hudgins et al. (1993) gives (
![]() cmmol-1), depending on the two interstellar ice mixtures they studied.
cmmol-1), depending on the two interstellar ice mixtures they studied.
We adopt the extreme values for the ratio of integrated absorbance of H2O/NH3, leading to a multiplicative factor of 15-24. This number must be multiplied by the integrated ratio measured on the spectra to derive the NH3 to H2O percentage upper limit. The results of the analysis are summarised in Table 1.
We emphasise here that the overall quality of ISO-SWS spectra on
these faint objects does not allow a very constraining upper
limit on the quantity of ammonia as the derived upper
limits are mostly due to the ultimate signal-to-noise
of the spectra. Ground based spectra in the 3
micron window, such as the ones discussed by Brooke et al.
(1999), provides a better S/N data in this range.
Yet no detection of any ammonia features at
![]() m is reported in this set of data, and none of the spectra seem to present any feature in this wavelength range.
m is reported in this set of data, and none of the spectra seem to present any feature in this wavelength range.
Among the infrared absorptions detected toward young stellar objects, resides a well known 3.47 ![]() m feature. This absorption can be studied from the ground as it falls in a spectral region almost free of H2O vapour. It was first observed and discussed by Allamandola et al. (1992), which attributed it to a CH stretch on a diamond surface. Since then, it has been systematically studied in many molecular clouds by Sellgren et al. (1994), Brooke et al. (1996), Brooke et al. (1999). It was also reported in two Taurus sources by Chiar et al. (1996). In 1996, Brooke et al. showed that the optical depth of this feature is correlated with H2O ice, a trend that is confirmed in their recent study (Brooke et al. 1999).
m feature. This absorption can be studied from the ground as it falls in a spectral region almost free of H2O vapour. It was first observed and discussed by Allamandola et al. (1992), which attributed it to a CH stretch on a diamond surface. Since then, it has been systematically studied in many molecular clouds by Sellgren et al. (1994), Brooke et al. (1996), Brooke et al. (1999). It was also reported in two Taurus sources by Chiar et al. (1996). In 1996, Brooke et al. showed that the optical depth of this feature is correlated with H2O ice, a trend that is confirmed in their recent study (Brooke et al. 1999).
In parallel, in the laboratory, when ice mixtures containing H2O and NH3 are deposited at low temperature, an additional absorption arises on the red wing of the water ice stretching mode feature. This new mode is in fact the consequence of the formation of an ammonia monohydrate in the matrix. We present here a laboratory study that shows that this band should participate to the unidentified 3.47 ![]() m band. It would fulfill all the requirements as compared to observations, that is to say:
m band. It would fulfill all the requirements as compared to observations, that is to say:
NH3 is actually known to form hydrates with H2O (Sill et al. 1980; Bertie & Morrison 1980; Mukai & Kratschmer 1985). As ice mantles on top of interstellar grains are dominated by water ice, we therefore expect such hydrates to be present if ammonia is indeed present in the ice. One of the main bands that is apparent when the formation of H2O.NH3 hydrate occurs in the ice is the
![]() (O-H ... N) mode around
2900 cm-1 (3.45
(O-H ... N) mode around
2900 cm-1 (3.45 ![]() m). This new mode is due to
the perturbation by ammonia molecules of the water
ice stretching frequency, following the length of the
(O-H ... N) hydrogen bond formed. To first order, it should not influence the 2.97
m). This new mode is due to
the perturbation by ammonia molecules of the water
ice stretching frequency, following the length of the
(O-H ... N) hydrogen bond formed. To first order, it should not influence the 2.97 ![]() m feature's integrated absorption cross section.
m feature's integrated absorption cross section.
Under very low mixing ratios, the ammonia modes will be very difficult to
detect in astronomical spectra. The strongest one around ![]() 9
9 ![]() m (
m (![]() 2,
umbrella mode) will form part of the silicate absorption band. To retrieve it,
we then need to obtain high signal to noise spectra in this region
and to be able to precisely evaluate the underlying silicate and other ices
absorptions. Given the width of this feature (see Fig. 5), it is a very difficult task.
2,
umbrella mode) will form part of the silicate absorption band. To retrieve it,
we then need to obtain high signal to noise spectra in this region
and to be able to precisely evaluate the underlying silicate and other ices
absorptions. Given the width of this feature (see Fig. 5), it is a very difficult task.
Let us now focus on the deformation modes (![]() 6.2
6.2 ![]() m).
They fall in the water ice bending mode and their spectral
signature vanish in the ice band,
if ammonia is not present at least at the 5-10% abundance level relative to water ice.
Similar processes affect the N-H stretching modes (
m).
They fall in the water ice bending mode and their spectral
signature vanish in the ice band,
if ammonia is not present at least at the 5-10% abundance level relative to water ice.
Similar processes affect the N-H stretching modes (![]() 2.97
2.97![]() m) which will suffer
from both effects (contrast and optical depth), due to its presence in the water ice
stretching mode wing.
m) which will suffer
from both effects (contrast and optical depth), due to its presence in the water ice
stretching mode wing.
To illustrate the points discussed above, we present in Fig. 5
the spectra of H2O:NH3 mixtures at a dilution level of 7%.
The intended dilution is monitored afterward by measuring the integrated
absorbances of the NH3 umbrella mode at ![]() 9
9![]() m and comparing it to the 6
m and comparing it to the 6 ![]() m bending mode of water ice. The respective integrated cross sections are of
m bending mode of water ice. The respective integrated cross sections are of
![]() cmmol-1 and
cmmol-1 and
![]() cmmol-1 (d'Hendecourt et al. 1986). We therefore deduce the dilution to be
cmmol-1 (d'Hendecourt et al. 1986). We therefore deduce the dilution to be ![]() 7%. The spectra are presented at three temperatures to show the temperature evolution of the features. At 10 K, in the left panel, the small bump at 2.96
7%. The spectra are presented at three temperatures to show the temperature evolution of the features. At 10 K, in the left panel, the small bump at 2.96 ![]() m in the water ice stretching mode is due to the NH3 stretching mode. When the temperature of the sample is raised, the observed band is dominated by the water ice crystallisation
shoulder.
m in the water ice stretching mode is due to the NH3 stretching mode. When the temperature of the sample is raised, the observed band is dominated by the water ice crystallisation
shoulder.
In Fig. 5, a new (large) absorption appears in the spectrum around 3.5 ![]() m,
indicated by a vertical dashed line. This band is due to the ammonia hydrate.
In Fig. 6 we present a close-up on the
m,
indicated by a vertical dashed line. This band is due to the ammonia hydrate.
In Fig. 6 we present a close-up on the
![]() m spectral region of
water ice spectra containing from zero to seven percent of ammonia. The lowest
spectrum of each panel is the superposition of the water ice mode and the 7%
spectrum. The difference is shaded to evidence the hydrate band. From this
difference we can evaluate the integrated absorbance of the pure water ice
and compare it to the hydrate one. The ratio of the two bands is almost the
same as the ratio of NH3 to H2O abundance in the mixture, indicating
that the hydrate integrated cross section is of
m spectral region of
water ice spectra containing from zero to seven percent of ammonia. The lowest
spectrum of each panel is the superposition of the water ice mode and the 7%
spectrum. The difference is shaded to evidence the hydrate band. From this
difference we can evaluate the integrated absorbance of the pure water ice
and compare it to the hydrate one. The ratio of the two bands is almost the
same as the ratio of NH3 to H2O abundance in the mixture, indicating
that the hydrate integrated cross section is of
![]() cmmol-1.
cmmol-1.
We address in this section an interesting byproduct of our study. To derive ice column densities in interstellar spectra one generally draws a local continuum on both sides of the band to integrate, taking ln(
![]() )
and use the fact that:
)
and use the fact that:

Once the spectra have been obtained, we normalise the integrated absorbance to the
3 ![]() m ice band, and apply this normalisation factor to the 6
m ice band, and apply this normalisation factor to the 6 ![]() m bending mode
extracted in both cases. We observe that the resultant spectra in the 3
m bending mode
extracted in both cases. We observe that the resultant spectra in the 3 ![]() m
window are not superimposed. Moreover, the 6
m
window are not superimposed. Moreover, the 6 ![]() m bending mode spectra differ
by a multipling factor (
m bending mode spectra differ
by a multipling factor (![]() )
but are otherwise identical.
)
but are otherwise identical.
The direct consequence is that if one decides to use the 3 ![]() m band to evaluate the column
density of water ice, it will disagree in this case by a factor
m band to evaluate the column
density of water ice, it will disagree in this case by a factor ![]() from the estimate made at
6
from the estimate made at
6 ![]() m. Finally, if the 3
m. Finally, if the 3 ![]() m band is saturated in the band center, estimating the column
density by adjusting the blue wing of the line is rather uncertain, and again a factor of two can
easily be introduced. This probably explains why, deriving the ice water column density from the
3
m band is saturated in the band center, estimating the column
density by adjusting the blue wing of the line is rather uncertain, and again a factor of two can
easily be introduced. This probably explains why, deriving the ice water column density from the
3 ![]() m band,
gives systematically a different number than the one derived from the 6
m band,
gives systematically a different number than the one derived from the 6 ![]() m
estimate, as noted by Allamandola et al. (1992). We emphasize here that we qualitatively demonstrate this effect by using two unique grain sizes. A full quantitative treatment of this effect must include the use of a grain size distribution as well as a proper radiative transfer model. This is beyond the scope of this paper.
m
estimate, as noted by Allamandola et al. (1992). We emphasize here that we qualitatively demonstrate this effect by using two unique grain sizes. A full quantitative treatment of this effect must include the use of a grain size distribution as well as a proper radiative transfer model. This is beyond the scope of this paper.
We have evaluated the integrated absorption cross section of the hydrate band in the laboratory. Brooke and Sellgren have measured a correlation between the optical depths of the water ice and 3.47 ![]() m absorptions. This relation,
m absorptions. This relation,
![]() ,
establishes the link in abundance between ammonia and water if the 3.47
,
establishes the link in abundance between ammonia and water if the 3.47 ![]() m band is attributed to the hydrate.
m band is attributed to the hydrate.
Working with this hypothesis, the NH3/H2O abundance ratio is therefore given by:

Using the integrated absorption cross-sections for water
ice and the one derived above for the hydrate,
the
![]() can been evaluated and equals
can been evaluated and equals
![]() .
Therefore the ammonia content is of 0.033 times this number, i.e.
.
Therefore the ammonia content is of 0.033 times this number, i.e.
![]() % in the sources used in the correlation by Brooke et al.
% in the sources used in the correlation by Brooke et al.
![\begin{figure}
\par\includegraphics[bb=42 207 548 540,width=8.8cm,clip]{ds10157f35.ps}\end{figure}](/articles/aa/full/2001/02/aa10157/Timg81.gif) |
Figure 8: Mie calculation of the resultant transmittance spectra of a coated grain composed of a silicate core and a water:ammonia (100:4) ice mantle. The long wavelength wing is better reproduced than in Fig. 4a, which point to the possible presence of an ammonia hydrate in the mantle |